At this point in the course, we should be familiar with what a derivative is and how a derivative relates to something known as the tangent line. These are both things that we've learned about in previous videos. Now in this video, we're going to be talking about situations that you're going to see in this course where you are given the graph of some function \( f(x) \), and you will need to sketch the derivative of that graph simply based on what the function looks like. Now, this might sound like something that's totally scary or abstract, but don't sweat it because in this video, I'm going to be walking you through an example of this type of problem. And I think you're going to find that if you can relate the derivative to the idea of a tangent line, then solving these types of problems actually becomes pretty straightforward.
So without further ado, let's just jump right into things to see what an example of this would look like. So in this problem, we are told for each \( x \) value or interval, determine if the slope of \( f(x) \), our function, is positive, negative, 0, or undefined, and then sketch the derivative \( f'(x) \). Now that's a lot just when we hear all those words, but let's just take this by the steps. So our first step is going to be to understand how this function in general behaves, and what the tangent lines are going to look like based on this function. Now recall that the derivative is the exact same thing as the slope of a tangent line.
So wherever we find the slope of a tangent line, that's going to be what the derivative value is at that specific point. Now, of course, what we could do to graph the derivative is literally just fill this curve with a bunch of points and find a bunch of tangent lines at every single point and write each one out. But that would take a lot of time because we could draw millions of points on this graph and millions of tangent lines, and that would just take forever. So it's not the most efficient way of solving these types of problems. But, again, if you understand the general behavior of these functions, then you can actually understand this and graph it in a much more efficient way.
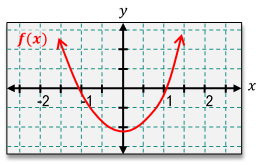
Now let's go ahead and start by just taking a look at what this graph does as we go from left to right. And the way that I like to solve these types of problems is to imagine there's a person literally standing on this graph, and they're walking from left to right. Now what we're going to do is first focus on this portion of the interval, which is negative infinity all the way down here, all the way up to negative one. So it's going to be at that point. Now how is this function going to be behaving as this person walks from left to right all the way to negative one?
Well, as this is happening, notice the person is going uphill. So you could say that the function is increasing. Now whenever the function is increasing, notice any time we draw a tangent line here, the tangent line is going to have a positive slope. And you can see that the derivative is going to be positive where the function is increasing. But what would happen as this person walked all the way up this hill and got to this point right here?
We'll notice at this point, we if we draw a tangent line, it's going to be completely flat. So we would get a horizontal tangent line, and the slope of any kind of horizontal or flat line is always going to be 0. So at this point, negative 1, the derivative is going to be 0. But now let's see what happens as we go to this next interval, which goes from negative one all the way over here to positive one. Well, at this interval, notice how a person would be walking downhill.
And because they're walking downhill, we could see that the function decreases. And when the function decreases, the slope of the tangent lines are all going to be negative, because notice how they slope down now instead of up. So we're going to have a negative derivative from this interval. So this is something that's important to recognize. Whenever you have a situation where your function is increasing, the derivative is going to be positive.
However, if it's decreasing, the function is going to be negative. And whenever you see a situation where you have a horizontal tangent line or you're basically flat, that means that your derivative is going to be 0. So this is the behavior that you need to understand when looking at a graph of some function. Now we're going to take a look next at this point right here at positive one. And notice once again, we can get a horizontal tangent line right there.
So because we get another flat line, the derivative is going to be 0. Now lastly, we'll take a look at this final interval from 1 to positive infinity, and notice at this point, the person is going to be going uphill. Because they're going uphill at this entire interval, that means that the derivative here is going to be positive because the function is increasing. So we've basically solved this entire portion of the problem, but now the question becomes, how exactly could we use all of this information to sketch what the derivative looks like? Well, the way that we can do this is by first finding the obvious points on our graph.
And in my opinion, the obvious points are always going to be where we have these flat tangent lines, because that's going to be where the slope is 0. So if I know that my derivative is 0, that means that it's literally touching the x axis. So notice that we have a flat tangent line here and there. Whenever you have these peaks and valleys in your graph, the tangent lines are going to be flat. So what I can do is I can draw this point to be right there, right on the x axis, and I can draw this point to be right here on the x axis.
Because, again, the y values are going to be 0 because the derivative is 0 at those points. Now notice here, we said that the_derivative_is_positive, and that's going to be on this whole interval, which on our graph here is going to correspond to this whole interval. So what that means is that if we are positive, that means it's going to be some value above the x axis where the outputs would be positive. Now notice at this interval right here, we said that we have a negative derivative. And because our derivatives are negative, that means somewhere here we're going to be below the x axis.
And then we can see for this last interval, the derivatives are positive, which means that we're going to be again above the x axis. Now what I can see at this part of the interval is these tangent lines are going to get closer and closer to flat until we reach this point where we actually are flat. So that means that we're going to start above the x I