Table of contents
- 0. Functions7h 52m
- Introduction to Functions16m
- Piecewise Functions10m
- Properties of Functions9m
- Common Functions1h 8m
- Transformations5m
- Combining Functions27m
- Exponent rules32m
- Exponential Functions28m
- Logarithmic Functions24m
- Properties of Logarithms34m
- Exponential & Logarithmic Equations35m
- Introduction to Trigonometric Functions38m
- Graphs of Trigonometric Functions44m
- Trigonometric Identities47m
- Inverse Trigonometric Functions48m
- 1. Limits and Continuity2h 2m
- 2. Intro to Derivatives1h 33m
- 3. Techniques of Differentiation3h 18m
- 4. Applications of Derivatives2h 38m
- 5. Graphical Applications of Derivatives6h 2m
- 6. Derivatives of Inverse, Exponential, & Logarithmic Functions2h 37m
- 7. Antiderivatives & Indefinite Integrals1h 26m
0. Functions
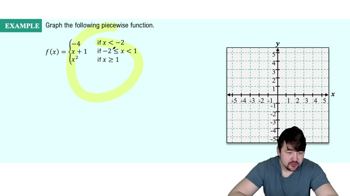
Piecewise Functions
Problem 1.25
Textbook Question
Defining piecewise functions Write a definition of the function whose graph is given <IMAGE>
 Verified step by step guidance
Verified step by step guidance1
<strong>Step 1:</strong> Identify the different segments of the graph. Look for changes in the slope, discontinuities, or any other features that indicate a new piece of the function.
<strong>Step 2:</strong> Determine the mathematical expression for each segment. This could be a linear equation, a quadratic, or another type of function, depending on the shape of the graph.
<strong>Step 3:</strong> Identify the domain for each piece of the function. This involves determining the x-values over which each segment is defined.
<strong>Step 4:</strong> Write the piecewise function using the expressions and domains identified in the previous steps. Use the format: \( f(x) = \begin{cases} \text{expression 1,} & \text{if } \text{domain 1} \\ \text{expression 2,} & \text{if } \text{domain 2} \\ \end{cases} \)
<strong>Step 5:</strong> Verify the piecewise function by checking that it matches the graph over the specified domains. Ensure continuity or note any discontinuities as needed.
Recommended similar problem, with video answer:
 Verified Solution
Verified SolutionThis video solution was recommended by our tutors as helpful for the problem above
Video duration:
4mPlay a video:
Was this helpful?
Key Concepts
Here are the essential concepts you must grasp in order to answer the question correctly.
Piecewise Functions
A piecewise function is defined by multiple sub-functions, each applying to a specific interval of the function's domain. This means that the function can take different forms based on the input value. For example, a piecewise function might be defined as f(x) = x^2 for x < 0 and f(x) = 2x + 1 for x ≥ 0, illustrating how the function behaves differently in different regions.
Recommended video:
Piecewise Functions
Domain and Range
The domain of a function refers to the set of all possible input values (x-values) for which the function is defined, while the range is the set of all possible output values (y-values). Understanding the domain and range is crucial for defining piecewise functions, as each piece may have its own specific domain, affecting how the function behaves overall.
Recommended video:
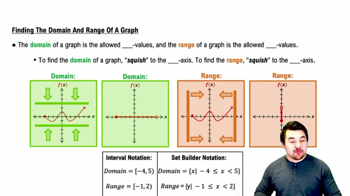
Finding the Domain and Range of a Graph
Graph Interpretation
Interpreting the graph of a function involves analyzing its shape, slopes, and intercepts to understand its behavior. For piecewise functions, the graph may consist of distinct segments, each corresponding to different rules. Recognizing where these segments start and end is essential for accurately defining the function and understanding its continuity and discontinuities.
Recommended video:

Graphing The Derivative
Related Videos
Related Practice