Table of contents
- 0. Functions7h 52m
- Introduction to Functions16m
- Piecewise Functions10m
- Properties of Functions9m
- Common Functions1h 8m
- Transformations5m
- Combining Functions27m
- Exponent rules32m
- Exponential Functions28m
- Logarithmic Functions24m
- Properties of Logarithms34m
- Exponential & Logarithmic Equations35m
- Introduction to Trigonometric Functions38m
- Graphs of Trigonometric Functions44m
- Trigonometric Identities47m
- Inverse Trigonometric Functions48m
- 1. Limits and Continuity2h 2m
- 2. Intro to Derivatives1h 33m
- 3. Techniques of Differentiation3h 18m
- 4. Applications of Derivatives2h 38m
- 5. Graphical Applications of Derivatives6h 2m
- 6. Derivatives of Inverse, Exponential, & Logarithmic Functions2h 37m
- 7. Antiderivatives & Indefinite Integrals1h 26m
- 8. Definite Integrals4h 44m
- 9. Graphical Applications of Integrals2h 27m
- 10. Physics Applications of Integrals 3h 16m
- 11. Integrals of Inverse, Exponential, & Logarithmic Functions2h 34m
0. Functions
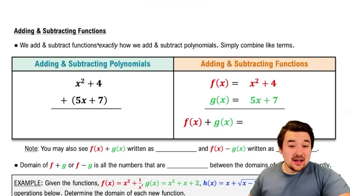
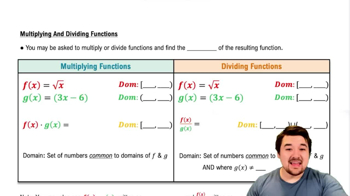
Combining Functions
Problem 60
Textbook Question
Missing piece Let g(x) = x² + 3 Find a function ƒ that produces the given composition.
(g o ƒ ) (x) = x²⸍³ + 3
 Verified step by step guidance
Verified step by step guidance1
Step 1: Understand the composition (g \circ f)(x) = g(f(x)). We need to find a function f(x) such that when g is applied to f(x), it results in x^{2/3} + 3.
Step 2: Recall that g(x) = x^2 + 3. We want g(f(x)) = f(x)^2 + 3 to equal x^{2/3} + 3.
Step 3: Set up the equation f(x)^2 + 3 = x^{2/3} + 3.
Step 4: Subtract 3 from both sides to isolate the squared term: f(x)^2 = x^{2/3}.
Step 5: Solve for f(x) by taking the square root of both sides: f(x) = \sqrt{x^{2/3}}.
Was this helpful?
Key Concepts
Here are the essential concepts you must grasp in order to answer the question correctly.
Function Composition
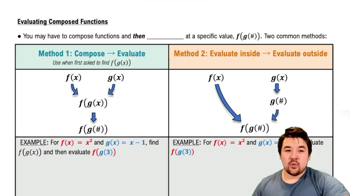
Function composition involves combining two functions where the output of one function becomes the input of another. In this case, the composition (g o ƒ)(x) means applying function ƒ first and then applying function g to the result. Understanding how to manipulate and combine functions is essential for solving problems involving compositions.
Recommended video:

Evaluate Composite Functions - Special Cases
Identifying Functions
To find the function ƒ that satisfies the composition (g o ƒ)(x) = x² + 3, we need to identify the structure of g(x) and how it relates to ƒ. Here, g(x) = x² + 3 suggests that ƒ must produce an input that, when squared and increased by 3, results in the desired output. Recognizing the form of g helps in determining the appropriate form of ƒ.
Recommended video:
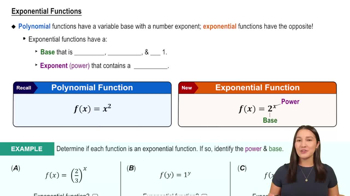
Exponential Functions
Inverse Functions
Inverse functions are crucial in understanding how to 'reverse' the operations of a function. If we can express g(x) in terms of its inverse, we can derive ƒ by manipulating the equation. For instance, if we can isolate x in terms of g, we can find the function that, when composed with g, yields the original input, aiding in solving the composition problem.
Recommended video:

Inverse Cosine

 5:56m
5:56mWatch next
Master Adding & Subtracting Functions with a bite sized video explanation from Nick
Start learning