Table of contents
- 0. Functions7h 52m
- Introduction to Functions16m
- Piecewise Functions10m
- Properties of Functions9m
- Common Functions1h 8m
- Transformations5m
- Combining Functions27m
- Exponent rules32m
- Exponential Functions28m
- Logarithmic Functions24m
- Properties of Logarithms34m
- Exponential & Logarithmic Equations35m
- Introduction to Trigonometric Functions38m
- Graphs of Trigonometric Functions44m
- Trigonometric Identities47m
- Inverse Trigonometric Functions48m
- 1. Limits and Continuity2h 2m
- 2. Intro to Derivatives1h 33m
- 3. Techniques of Differentiation3h 18m
- 4. Applications of Derivatives2h 38m
- 5. Graphical Applications of Derivatives6h 2m
- 6. Derivatives of Inverse, Exponential, & Logarithmic Functions2h 37m
- 7. Antiderivatives & Indefinite Integrals1h 26m
- 8. Definite Integrals4h 44m
- 9. Graphical Applications of Integrals2h 27m
- 10. Physics Applications of Integrals 2h 22m
0. Functions
Introduction to Functions
Problem 1.32
Textbook Question
In Exercises 19–32, find the (a) domain and (b) range.
𝔂 = 3 cos x + 4 sin x (Hint: A trig identity is required.)
 Verified step by step guidance
Verified step by step guidance1
Step 1: Recognize that the function y = 3 cos(x) + 4 sin(x) is a linear combination of sine and cosine functions. The domain of sine and cosine functions is all real numbers, so the domain of y is also all real numbers.
Step 2: To find the range, use the trigonometric identity for a linear combination of sine and cosine: a cos(x) + b sin(x) = R cos(x - φ), where R = √(a² + b²) and φ is a phase shift.
Step 3: Calculate R using the coefficients of the sine and cosine terms: R = √(3² + 4²) = √(9 + 16) = √25 = 5.
Step 4: The expression R cos(x - φ) has a range of [-R, R] because the cosine function oscillates between -1 and 1. Therefore, the range of y = 3 cos(x) + 4 sin(x) is [-5, 5].
Step 5: Conclude that the domain of the function is all real numbers, and the range is [-5, 5].
 Verified video answer for a similar problem:
Verified video answer for a similar problem:This video solution was recommended by our tutors as helpful for the problem above
Video duration:
5mPlay a video:
Was this helpful?
Key Concepts
Here are the essential concepts you must grasp in order to answer the question correctly.
Domain of a Function
The domain of a function refers to the set of all possible input values (x-values) for which the function is defined. For trigonometric functions like sine and cosine, the domain is typically all real numbers, as these functions can take any real number as input. However, when combined in an expression, the overall domain may be influenced by other factors, such as restrictions from the context of the problem.
Recommended video:
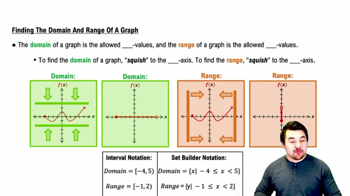
Finding the Domain and Range of a Graph
Range of a Function
The range of a function is the set of all possible output values (y-values) that the function can produce. For the function given, 𝔶 = 3 cos x + 4 sin x, the range can be determined by analyzing the maximum and minimum values of the expression. Using trigonometric identities, one can express this function in a form that reveals its amplitude and thus its range.
Recommended video:

Finding the Domain and Range of a Graph
Trigonometric Identities
Trigonometric identities are equations that involve trigonometric functions and are true for all values of the variables involved. A common identity used in problems involving sine and cosine is the Pythagorean identity, which states that sin²(x) + cos²(x) = 1. In this case, a relevant identity can help simplify the expression 3 cos x + 4 sin x into a single sinusoidal function, making it easier to determine the range.
Recommended video:
Verifying Trig Equations as Identities

 1:36m
1:36mWatch next
Master Introduction to Calculus Channel with a bite sized video explanation from Callie
Start learning