Table of contents
- 0. Functions7h 52m
- Introduction to Functions16m
- Piecewise Functions10m
- Properties of Functions9m
- Common Functions1h 8m
- Transformations5m
- Combining Functions27m
- Exponent rules32m
- Exponential Functions28m
- Logarithmic Functions24m
- Properties of Logarithms34m
- Exponential & Logarithmic Equations35m
- Introduction to Trigonometric Functions38m
- Graphs of Trigonometric Functions44m
- Trigonometric Identities47m
- Inverse Trigonometric Functions48m
- 1. Limits and Continuity2h 2m
- 2. Intro to Derivatives1h 33m
- 3. Techniques of Differentiation3h 18m
- 4. Applications of Derivatives2h 38m
- 5. Graphical Applications of Derivatives6h 2m
- 6. Derivatives of Inverse, Exponential, & Logarithmic Functions2h 37m
- 7. Antiderivatives & Indefinite Integrals1h 26m
- 8. Definite Integrals4h 44m
- 9. Graphical Applications of Integrals2h 27m
- 10. Physics Applications of Integrals 2h 22m
4. Applications of Derivatives
Differentials
Problem 4.6.53c
Textbook Question
Explain why or why not Determine whether the following statements are true and give an explanation or counterexample.
c. If f(x) = mx + b, then the linear approximation to f at any point is L(x) = f(x).
 Verified step by step guidance
Verified step by step guidance1
First, understand the concept of linear approximation. Linear approximation is a method used to approximate the value of a function near a given point using the tangent line at that point.
For a function f(x), the linear approximation at a point x = a is given by the formula L(x) = f(a) + f'(a)(x - a), where f'(a) is the derivative of f at x = a.
Consider the function f(x) = mx + b, which is a linear function. The derivative of f(x) with respect to x is f'(x) = m, since the derivative of mx is m and the derivative of a constant b is 0.
Substitute f(x) = mx + b and f'(x) = m into the linear approximation formula: L(x) = f(a) + m(x - a). Since f(a) = ma + b, the formula becomes L(x) = ma + b + m(x - a).
Simplify the expression: L(x) = ma + b + mx - ma = mx + b. Notice that L(x) = f(x), which confirms that for a linear function, the linear approximation at any point is indeed the function itself.
 Verified video answer for a similar problem:
Verified video answer for a similar problem:This video solution was recommended by our tutors as helpful for the problem above
Video duration:
2mPlay a video:
Was this helpful?
Key Concepts
Here are the essential concepts you must grasp in order to answer the question correctly.
Linear Functions
A linear function is a polynomial function of degree one, typically expressed in the form f(x) = mx + b, where m is the slope and b is the y-intercept. This function produces a straight line when graphed, and its properties include constant rate of change and predictable behavior across its domain.
Recommended video:
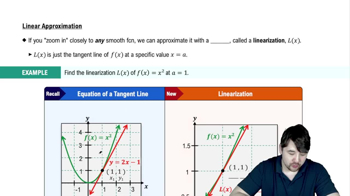
Linearization
Linear Approximation
Linear approximation is a method used to estimate the value of a function near a given point using the tangent line at that point. For a function f at point a, the linear approximation L(x) is given by L(x) = f(a) + f'(a)(x - a). This technique is particularly useful for simplifying complex functions in calculus.
Recommended video:

Linearization
Tangent Line
The tangent line to a curve at a given point is a straight line that touches the curve at that point and has the same slope as the curve at that point. In the context of linear approximation, the tangent line serves as the best linear estimate of the function's behavior near that point, making it crucial for understanding how well the approximation represents the function.
Recommended video:
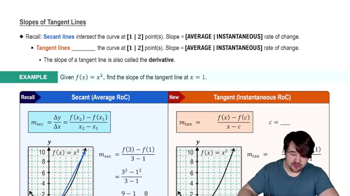
Slopes of Tangent Lines