Table of contents
- 0. Functions7h 52m
- Introduction to Functions16m
- Piecewise Functions10m
- Properties of Functions9m
- Common Functions1h 8m
- Transformations5m
- Combining Functions27m
- Exponent rules32m
- Exponential Functions28m
- Logarithmic Functions24m
- Properties of Logarithms34m
- Exponential & Logarithmic Equations35m
- Introduction to Trigonometric Functions38m
- Graphs of Trigonometric Functions44m
- Trigonometric Identities47m
- Inverse Trigonometric Functions48m
- 1. Limits and Continuity2h 2m
- 2. Intro to Derivatives1h 33m
- 3. Techniques of Differentiation3h 18m
- 4. Applications of Derivatives2h 38m
- 5. Graphical Applications of Derivatives6h 2m
- 6. Derivatives of Inverse, Exponential, & Logarithmic Functions2h 37m
- 7. Antiderivatives & Indefinite Integrals1h 26m
- 8. Definite Integrals4h 44m
- 9. Graphical Applications of Integrals2h 27m
- 10. Physics Applications of Integrals 2h 22m
4. Applications of Derivatives
Differentials
Problem 4.2.42
Textbook Question
Avalanche forecasting Avalanche forecasters measure the temperature gradient dT/dh, which is the rate at which the temperature in a snowpack T changes with respect to its depth h. A large temperature gradient may lead to a weak layer in the snowpack. When these weak layers collapse, avalanches occur. Avalanche forecasters use the following rule of thumb: If dT/dh exceeds 10° C/m anywhere in the snowpack, conditions are favorable for weak-layer formation, and the risk of avalanche increases. Assume the temperature function is continuous and differentiable.
a. An avalanche forecaster digs a snow pit and takes two temperature measurements. At the surface (h = 0), the temperature is -16° C. At a depth of 1.1 m, the temperature is -2° C. Using the Mean Value Theorem, what can he conclude about the temperature gradient? Is the formation of a weak layer likely?
 Verified step by step guidance
Verified step by step guidance1
Identify the given information: The temperature at the surface (h = 0) is -16°C, and at a depth of 1.1 m, the temperature is -2°C.
Recall the Mean Value Theorem (MVT) for derivatives, which states that if a function f is continuous on [a, b] and differentiable on (a, b), then there exists at least one c in (a, b) such that f'(c) = (f(b) - f(a)) / (b - a).
Apply the Mean Value Theorem to the temperature function T(h) over the interval [0, 1.1]. Here, f(a) = T(0) = -16°C and f(b) = T(1.1) = -2°C.
Calculate the average rate of change of temperature over the interval [0, 1.1] using the formula: (T(1.1) - T(0)) / (1.1 - 0).
Interpret the result: If the calculated average rate of change exceeds 10°C/m, then according to the rule of thumb, the conditions are favorable for weak-layer formation, increasing the risk of an avalanche.
 Verified video answer for a similar problem:
Verified video answer for a similar problem:This video solution was recommended by our tutors as helpful for the problem above
Video duration:
4mPlay a video:
Was this helpful?
Key Concepts
Here are the essential concepts you must grasp in order to answer the question correctly.
Mean Value Theorem
The Mean Value Theorem states that for a continuous function that is differentiable on an interval, there exists at least one point in that interval where the derivative (slope) of the function equals the average rate of change over the interval. In this context, it allows the forecaster to conclude that there is a specific depth at which the temperature gradient can be calculated, providing insight into the behavior of the temperature function in the snowpack.
Recommended video:

Fundamental Theorem of Calculus Part 1
Temperature Gradient
The temperature gradient, denoted as dT/dh, measures how temperature changes with respect to depth in the snowpack. A steep gradient indicates a rapid change in temperature over a small depth, which can lead to the formation of weak layers. Understanding this gradient is crucial for avalanche forecasting, as it helps predict conditions that may lead to instability in the snowpack.
Weak Layer Formation
Weak layer formation in a snowpack occurs when there are significant temperature differences within the layers of snow, often indicated by a high temperature gradient. When the gradient exceeds a certain threshold, such as 10° C/m, it suggests that the snow structure may be compromised, increasing the likelihood of avalanches. Recognizing these conditions is essential for avalanche forecasters to assess risk and implement safety measures.
Recommended video:
Guided course
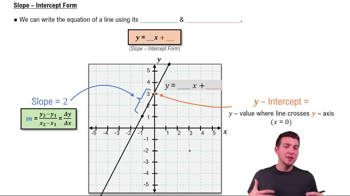
Slope-Intercept Form