Table of contents
- 0. Functions7h 52m
- Introduction to Functions16m
- Piecewise Functions10m
- Properties of Functions9m
- Common Functions1h 8m
- Transformations5m
- Combining Functions27m
- Exponent rules32m
- Exponential Functions28m
- Logarithmic Functions24m
- Properties of Logarithms34m
- Exponential & Logarithmic Equations35m
- Introduction to Trigonometric Functions38m
- Graphs of Trigonometric Functions44m
- Trigonometric Identities47m
- Inverse Trigonometric Functions48m
- 1. Limits and Continuity2h 2m
- 2. Intro to Derivatives1h 33m
- 3. Techniques of Differentiation3h 18m
- 4. Applications of Derivatives2h 38m
- 5. Graphical Applications of Derivatives6h 2m
- 6. Derivatives of Inverse, Exponential, & Logarithmic Functions2h 37m
- 7. Antiderivatives & Indefinite Integrals1h 26m
2. Intro to Derivatives
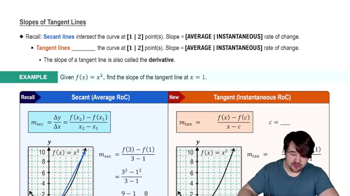
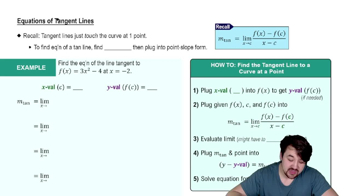
Tangent Lines and Derivatives
Problem 3.2.73c
Textbook Question
Vertical tangent lines If a function f is continuous at a and lim x→a| f′(x)|=∞, then the curve y=f(x) has a vertical tangent line at a, and the equation of the tangent line is x=a. If a is an endpoint of a domain, then the appropriate one-sided derivative (Exercises 71–72) is used. Use this information to answer the following questions.
73. {Use of Tech} Graph the following functions and determine the location of the vertical tangent lines.
c. f(x) = √|x-4|
 Verified step by step guidance
Verified step by step guidance1
Step 1: Understand the problem. We need to find the location of vertical tangent lines for the function f(x) = \sqrt{|x-4|}. A vertical tangent line occurs where the derivative of the function approaches infinity.
Step 2: Analyze the function. The function f(x) = \sqrt{|x-4|} is continuous everywhere in its domain, which is all real numbers. However, the expression inside the square root, |x-4|, changes behavior at x = 4.
Step 3: Find the derivative of the function. To find where the vertical tangent line occurs, we need to compute the derivative f'(x). Start by rewriting the function as f(x) = (|x-4|)^{1/2}. Use the chain rule and the derivative of the absolute value function to find f'(x).
Step 4: Evaluate the behavior of the derivative at x = 4. Since the absolute value function has a corner at x = 4, check the limit of the derivative as x approaches 4 from both sides. Specifically, calculate \lim_{x \to 4^-} f'(x) and \lim_{x \to 4^+} f'(x).
Step 5: Determine the location of the vertical tangent line. If either of the one-sided limits from Step 4 approaches infinity, then there is a vertical tangent line at x = 4. The equation of this vertical tangent line is x = 4.
Recommended similar problem, with video answer:
 Verified Solution
Verified SolutionThis video solution was recommended by our tutors as helpful for the problem above
Video duration:
6mPlay a video:
Was this helpful?

 5:13m
5:13mWatch next
Master Slopes of Tangent Lines with a bite sized video explanation from Nick
Start learningRelated Videos
Related Practice