Table of contents
- 0. Functions7h 52m
- Introduction to Functions16m
- Piecewise Functions10m
- Properties of Functions9m
- Common Functions1h 8m
- Transformations5m
- Combining Functions27m
- Exponent rules32m
- Exponential Functions28m
- Logarithmic Functions24m
- Properties of Logarithms34m
- Exponential & Logarithmic Equations35m
- Introduction to Trigonometric Functions38m
- Graphs of Trigonometric Functions44m
- Trigonometric Identities47m
- Inverse Trigonometric Functions48m
- 1. Limits and Continuity2h 2m
- 2. Intro to Derivatives1h 33m
- 3. Techniques of Differentiation3h 18m
- 4. Applications of Derivatives2h 38m
- 5. Graphical Applications of Derivatives6h 2m
- 6. Derivatives of Inverse, Exponential, & Logarithmic Functions2h 37m
- 7. Antiderivatives & Indefinite Integrals1h 26m
- 8. Definite Integrals4h 44m
- 9. Graphical Applications of Integrals2h 27m
- 10. Physics Applications of Integrals 2h 22m
5. Graphical Applications of Derivatives
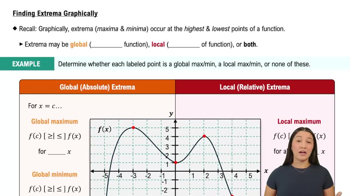
Intro to Extrema
Problem 4.1.31
Textbook Question
Locating critical points Find the critical points of the following functions. Assume a is a nonzero constant.
ƒ(t) = t/ t² + 1
 Verified step by step guidance
Verified step by step guidance1
To find the critical points of the function \( f(t) = \frac{t}{t^2 + 1} \), we first need to find its derivative \( f'(t) \).
Use the quotient rule for differentiation, which states that if \( f(t) = \frac{u(t)}{v(t)} \), then \( f'(t) = \frac{u'(t)v(t) - u(t)v'(t)}{(v(t))^2} \). Here, \( u(t) = t \) and \( v(t) = t^2 + 1 \).
Calculate \( u'(t) \) and \( v'(t) \). We have \( u'(t) = 1 \) and \( v'(t) = 2t \).
Substitute \( u(t) \), \( v(t) \), \( u'(t) \), and \( v'(t) \) into the quotient rule formula to find \( f'(t) = \frac{(1)(t^2 + 1) - (t)(2t)}{(t^2 + 1)^2} \). Simplify the expression to get \( f'(t) = \frac{1 - t^2}{(t^2 + 1)^2} \).
Set \( f'(t) = 0 \) to find the critical points. This gives \( 1 - t^2 = 0 \). Solve for \( t \) to find the critical points.
 Verified video answer for a similar problem:
Verified video answer for a similar problem:This video solution was recommended by our tutors as helpful for the problem above
Video duration:
4mPlay a video:
Was this helpful?
Key Concepts
Here are the essential concepts you must grasp in order to answer the question correctly.
Critical Points
Critical points of a function occur where its derivative is either zero or undefined. These points are essential for identifying local maxima, minima, and points of inflection. To find critical points, one typically differentiates the function and solves for the values of the variable that satisfy these conditions.
Recommended video:

Critical Points
Derivative
The derivative of a function measures the rate at which the function's value changes with respect to changes in its input. It is a fundamental concept in calculus that provides information about the function's slope and behavior. For the function given, finding the derivative will help identify where the critical points are located.
Recommended video:

Derivatives
Rational Functions
A rational function is a ratio of two polynomials. In the given function, ƒ(t) = t / (t² + 1), the numerator is a polynomial of degree one, and the denominator is a polynomial of degree two. Understanding the properties of rational functions, such as their domain and behavior at asymptotes, is crucial for analyzing their critical points and overall shape.
Recommended video:
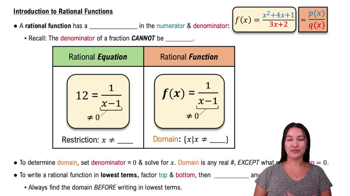
Intro to Rational Functions

 5:58m
5:58mWatch next
Master Finding Extrema Graphically with a bite sized video explanation from Callie
Start learning