Table of contents
- 0. Functions7h 52m
- Introduction to Functions16m
- Piecewise Functions10m
- Properties of Functions9m
- Common Functions1h 8m
- Transformations5m
- Combining Functions27m
- Exponent rules32m
- Exponential Functions28m
- Logarithmic Functions24m
- Properties of Logarithms34m
- Exponential & Logarithmic Equations35m
- Introduction to Trigonometric Functions38m
- Graphs of Trigonometric Functions44m
- Trigonometric Identities47m
- Inverse Trigonometric Functions48m
- 1. Limits and Continuity2h 2m
- 2. Intro to Derivatives1h 33m
- 3. Techniques of Differentiation3h 18m
- 4. Applications of Derivatives2h 38m
- 5. Graphical Applications of Derivatives6h 2m
- 6. Derivatives of Inverse, Exponential, & Logarithmic Functions2h 37m
- 7. Antiderivatives & Indefinite Integrals1h 26m
- 8. Definite Integrals4h 44m
- 9. Graphical Applications of Integrals2h 27m
- 10. Physics Applications of Integrals 2h 22m
0. Functions
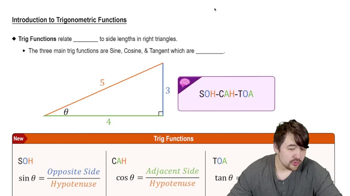
Introduction to Trigonometric Functions
Problem 1.3
Textbook Question
A point P in the first quadrant lies on the parabola 𝔂 = 𝔁². Express the coordinates of P as functions of the angle of inclination of the line joining P to the origin.
 Verified step by step guidance
Verified step by step guidance1
Start by understanding the problem: We need to express the coordinates of a point P on the parabola y = x² in terms of the angle θ, which is the angle of inclination of the line joining P to the origin.
Recall that the angle of inclination θ of a line with respect to the positive x-axis can be expressed using the tangent function: tan(θ) = y/x. Since P lies on the parabola y = x², substitute y = x² into the tangent expression: tan(θ) = x²/x = x.
From the equation tan(θ) = x, solve for x in terms of θ: x = tan(θ). This gives us the x-coordinate of point P as a function of θ.
Substitute x = tan(θ) back into the parabola equation y = x² to find the y-coordinate: y = (tan(θ))².
Thus, the coordinates of point P can be expressed as functions of θ: P(tan(θ), (tan(θ))²).
 Verified video answer for a similar problem:
Verified video answer for a similar problem:This video solution was recommended by our tutors as helpful for the problem above
Video duration:
4mPlay a video:
Was this helpful?
Key Concepts
Here are the essential concepts you must grasp in order to answer the question correctly.
Parabola
A parabola is a symmetric curve defined by a quadratic equation, such as y = x². In this case, it opens upwards and has its vertex at the origin (0,0). Understanding the properties of parabolas, including their vertex, axis of symmetry, and how points on the curve relate to the equation, is essential for analyzing the position of point P.
Recommended video:
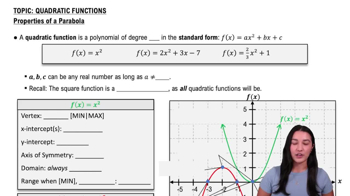
Properties of Parabolas
Polar Coordinates
Polar coordinates represent points in a plane using a distance from a reference point (the origin) and an angle from a reference direction (usually the positive x-axis). The relationship between Cartesian coordinates (x, y) and polar coordinates (r, θ) is given by x = r cos(θ) and y = r sin(θ). This concept is crucial for expressing the coordinates of point P in terms of the angle of inclination.
Recommended video:

Determining Concavity from the Graph of f' or f'' Example 1
Angle of Inclination
The angle of inclination refers to the angle formed between a line and the positive x-axis. In this context, it describes the direction of the line connecting point P to the origin. Understanding how to relate this angle to the coordinates of point P allows for the expression of P's coordinates as functions of the angle, facilitating the analysis of its position on the parabola.
Recommended video:
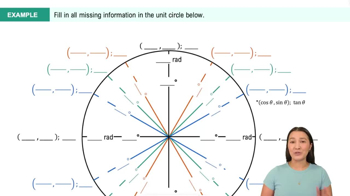
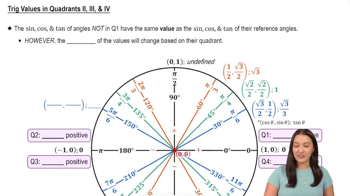
Trig Values in Quadrants II, III, & IV Example 2

 5:4m
5:4mWatch next
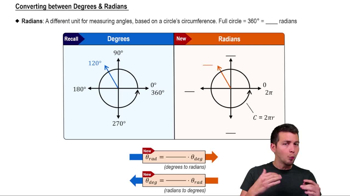
Master Converting between Degrees & Radians with a bite sized video explanation from Patrick Ford
Start learning