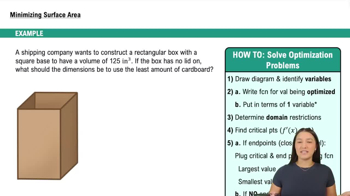
Maximum-volume cone A cone is constructed by cutting a sector from a circular sheet of metal with radius 20. The cut sheet is then folded up and welded (see figure). Find the radius and height of the cone with maximum volume that can be formed in this way. <IMAGE>

Verified step by step guidance

1. Let the angle of the sector be θ (in radians). The arc length of the sector will become the circumference of the base of the cone, which can be expressed as 20θ = 2πr, where r is the radius of the cone's base.
2. From the equation 20θ = 2πr, solve for r in terms of θ: r = (20θ)/(2π) = (10θ)/π.
3. The height h of the cone can be determined using the Pythagorean theorem. The slant height of the cone is the radius of the original circle (20), so h = √(20² - r²). Substitute r from the previous step into this equation.
4. The volume V of the cone can be expressed as V = (1/3)πr²h. Substitute the expressions for r and h in terms of θ into this volume formula.
5. To find the maximum volume, take the derivative of V with respect to θ, set the derivative equal to zero, and solve for θ. Then use this value to find the corresponding r and h.