Table of contents
- 0. Functions7h 52m
- Introduction to Functions16m
- Piecewise Functions10m
- Properties of Functions9m
- Common Functions1h 8m
- Transformations5m
- Combining Functions27m
- Exponent rules32m
- Exponential Functions28m
- Logarithmic Functions24m
- Properties of Logarithms34m
- Exponential & Logarithmic Equations35m
- Introduction to Trigonometric Functions38m
- Graphs of Trigonometric Functions44m
- Trigonometric Identities47m
- Inverse Trigonometric Functions48m
- 1. Limits and Continuity2h 2m
- 2. Intro to Derivatives1h 33m
- 3. Techniques of Differentiation3h 18m
- 4. Applications of Derivatives2h 38m
- 5. Graphical Applications of Derivatives6h 2m
- 6. Derivatives of Inverse, Exponential, & Logarithmic Functions2h 37m
- 7. Antiderivatives & Indefinite Integrals1h 26m
- 8. Definite Integrals4h 44m
- 9. Graphical Applications of Integrals2h 27m
- 10. Physics Applications of Integrals 2h 22m
5. Graphical Applications of Derivatives
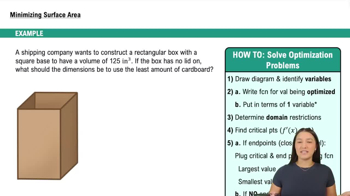
Applied Optimization
Problem 4.5.70d
Textbook Question
Rectangles in triangles Find the dimensions and area of the rectangle of maximum area that can be inscribed in the following figures.
d. An arbitrary triangle with a given area A (The result applies to any triangle, but first consider triangles for which all the angles are less than or equal to 90° .)
 Verified step by step guidance
Verified step by step guidance1
Consider an arbitrary triangle with a given area A. We want to inscribe a rectangle of maximum area within this triangle.
Position the triangle in the coordinate plane such that one of its sides lies along the x-axis. This simplifies the problem by allowing us to use the base and height of the triangle in our calculations.
Let the base of the triangle be b and the height be h. The area of the triangle is given by A = (1/2) * b * h.
To maximize the area of the inscribed rectangle, we can use calculus. Let the width of the rectangle be x and the height be y. The area of the rectangle is A_rect = x * y.
Using similar triangles, express y in terms of x, b, and h. Then, substitute this expression into A_rect to get a function of x. Differentiate this function with respect to x, set the derivative to zero, and solve for x to find the dimensions that maximize the area of the rectangle.
 Verified video answer for a similar problem:
Verified video answer for a similar problem:This video solution was recommended by our tutors as helpful for the problem above
Video duration:
1mPlay a video:
Was this helpful?
Key Concepts
Here are the essential concepts you must grasp in order to answer the question correctly.
Inscribed Figures
An inscribed figure is a shape placed within another shape such that all vertices of the inscribed figure touch the boundary of the outer shape. In this context, we are interested in a rectangle inscribed within a triangle, which means that the rectangle's corners will touch the sides of the triangle. Understanding how to position the rectangle optimally is crucial for maximizing its area.
Recommended video:

Changing Geometries
Optimization
Optimization in calculus involves finding the maximum or minimum values of a function. In this problem, we need to determine the dimensions of the rectangle that yield the maximum area while being constrained by the triangle's sides. This typically involves using techniques such as derivatives to find critical points and applying the second derivative test to confirm whether these points yield a maximum area.
Recommended video:

Intro to Applied Optimization: Maximizing Area
Area of a Triangle
The area of a triangle can be calculated using the formula A = 1/2 * base * height. This concept is essential because the area of the triangle provides a constraint for the dimensions of the inscribed rectangle. Knowing the area allows us to relate the dimensions of the rectangle to the triangle's geometry, which is vital for solving the problem effectively.
Recommended video:

Estimating the Area Under a Curve Using Left Endpoints

 1:13m
1:13mWatch next
Master Intro to Applied Optimization: Maximizing Area with a bite sized video explanation from Callie
Start learningRelated Videos
Related Practice