Table of contents
- 0. Functions7h 52m
- Introduction to Functions16m
- Piecewise Functions10m
- Properties of Functions9m
- Common Functions1h 8m
- Transformations5m
- Combining Functions27m
- Exponent rules32m
- Exponential Functions28m
- Logarithmic Functions24m
- Properties of Logarithms34m
- Exponential & Logarithmic Equations35m
- Introduction to Trigonometric Functions38m
- Graphs of Trigonometric Functions44m
- Trigonometric Identities47m
- Inverse Trigonometric Functions48m
- 1. Limits and Continuity2h 2m
- 2. Intro to Derivatives1h 33m
- 3. Techniques of Differentiation3h 18m
- 4. Applications of Derivatives2h 38m
- 5. Graphical Applications of Derivatives6h 2m
- 6. Derivatives of Inverse, Exponential, & Logarithmic Functions2h 37m
- 7. Antiderivatives & Indefinite Integrals1h 26m
- 8. Definite Integrals4h 44m
- 9. Graphical Applications of Integrals2h 27m
- 10. Physics Applications of Integrals 2h 22m
5. Graphical Applications of Derivatives
Curve Sketching
Problem 4.4.77
Textbook Question
{Use of Tech} Special curves The following classical curves have been studied by generations of mathematicians. Use analytical methods (including implicit differentiation) and a graphing utility to graph the curves. Include as much detail as possible.
x⁴ - x² + y² = 0 (Figure-8 curve)
 Verified step by step guidance
Verified step by step guidance1
Start by understanding the equation of the curve: \(x^4 - x^2 + y^2 = 0\). This is known as the Figure-8 curve due to its shape.
To analyze the curve, we can use implicit differentiation. Differentiate both sides of the equation with respect to \(x\). Remember that \(y\) is a function of \(x\), so when differentiating \(y^2\), apply the chain rule.
The differentiation of \(x^4\) with respect to \(x\) is \(4x^3\), and the differentiation of \(-x^2\) is \(-2x\). For \(y^2\), use the chain rule: \(2y \frac{dy}{dx}\). Set the derivative of the entire equation to zero: \(4x^3 - 2x + 2y \frac{dy}{dx} = 0\).
Solve for \(\frac{dy}{dx}\) to find the slope of the tangent line at any point on the curve. Rearrange the equation to isolate \(\frac{dy}{dx}\): \(\frac{dy}{dx} = \frac{2x - 4x^3}{2y}\). This gives the rate of change of \(y\) with respect to \(x\).
Use a graphing utility to plot the curve \(x^4 - x^2 + y^2 = 0\). Observe the symmetry and the figure-8 shape. Analyze the behavior of the curve at critical points, such as where \(x = 0\) or \(y = 0\), to understand its structure and features.
 Verified video answer for a similar problem:
Verified video answer for a similar problem:This video solution was recommended by our tutors as helpful for the problem above
Video duration:
10mPlay a video:
Was this helpful?
Key Concepts
Here are the essential concepts you must grasp in order to answer the question correctly.
Implicit Differentiation
Implicit differentiation is a technique used to find the derivative of a function defined implicitly by an equation involving both x and y. Instead of solving for y explicitly, we differentiate both sides of the equation with respect to x, applying the chain rule when differentiating y terms. This method is particularly useful for curves that cannot be easily expressed as y = f(x).
Recommended video:
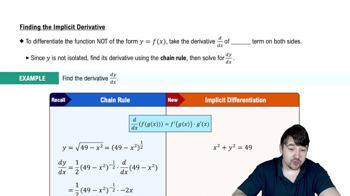
Finding The Implicit Derivative
Graphing Utility
A graphing utility is a software tool or calculator that allows users to visualize mathematical functions and equations. It can plot curves, analyze their properties, and provide insights into their behavior. For the given equation, a graphing utility can help illustrate the shape of the figure-8 curve, making it easier to understand its characteristics and intersections.
Recommended video:

Graphing The Derivative
Classical Curves
Classical curves refer to well-studied mathematical shapes that have significant historical and theoretical importance, such as conics, cycloids, and the figure-8 curve. These curves often arise in various fields of mathematics and physics, and their properties, such as symmetry and continuity, are essential for understanding their behavior. The figure-8 curve, defined by the equation x⁴ - x² + y² = 0, is an example of a classical curve that exhibits interesting geometric features.
Recommended video:

Summary of Curve Sketching

 11:41m
11:41mWatch next
Master Summary of Curve Sketching with a bite sized video explanation from Callie
Start learning