Table of contents
- 0. Functions7h 52m
- Introduction to Functions16m
- Piecewise Functions10m
- Properties of Functions9m
- Common Functions1h 8m
- Transformations5m
- Combining Functions27m
- Exponent rules32m
- Exponential Functions28m
- Logarithmic Functions24m
- Properties of Logarithms34m
- Exponential & Logarithmic Equations35m
- Introduction to Trigonometric Functions38m
- Graphs of Trigonometric Functions44m
- Trigonometric Identities47m
- Inverse Trigonometric Functions48m
- 1. Limits and Continuity2h 2m
- 2. Intro to Derivatives1h 33m
- 3. Techniques of Differentiation3h 18m
- 4. Applications of Derivatives2h 38m
- 5. Graphical Applications of Derivatives6h 2m
- 6. Derivatives of Inverse, Exponential, & Logarithmic Functions2h 37m
- 7. Antiderivatives & Indefinite Integrals1h 26m
- 8. Definite Integrals4h 44m
- 9. Graphical Applications of Integrals2h 27m
- 10. Physics Applications of Integrals 3h 16m
- 11. Integrals of Inverse, Exponential, & Logarithmic Functions2h 34m
0. Functions
Common Functions
Problem 17
Textbook Question
Find the linear function whose graph passes through the point (3, 2) and is parallel to the line .
 Verified step by step guidance
Verified step by step guidance1
<insert step 1> Identify the slope of the given line, which is parallel to the line we need to find. The given line is \( y = 3x + 8 \), so the slope \( m \) is 3.
<insert step 2> Use the point-slope form of a linear equation, which is \( y - y_1 = m(x - x_1) \), where \( (x_1, y_1) \) is a point on the line and \( m \) is the slope.
<insert step 3> Substitute the point \( (3, 2) \) and the slope \( m = 3 \) into the point-slope form: \( y - 2 = 3(x - 3) \).
<insert step 4> Simplify the equation to get it into the slope-intercept form \( y = mx + b \).
<insert step 5> Distribute the 3 on the right side: \( y - 2 = 3x - 9 \), then add 2 to both sides to solve for \( y \).
 Verified video answer for a similar problem:
Verified video answer for a similar problem:This video solution was recommended by our tutors as helpful for the problem above
Video duration:
4mPlay a video:
Was this helpful?
Key Concepts
Here are the essential concepts you must grasp in order to answer the question correctly.
Linear Functions
A linear function is a mathematical expression that describes a straight line when graphed. It is typically represented in the form y = mx + b, where m is the slope and b is the y-intercept. Understanding linear functions is crucial for determining the relationship between variables and for solving problems involving lines.
Recommended video:
Linearization
Slope
The slope of a line measures its steepness and direction, calculated as the change in y divided by the change in x (rise over run). For two parallel lines, the slopes are equal. In this question, the slope of the given line y = 3x + 8 is 3, which will be the same for the linear function we need to find.
Recommended video:
Guided course
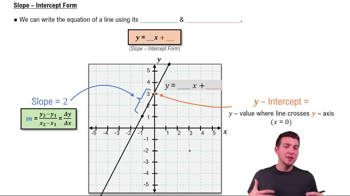
Slope-Intercept Form
Point-Slope Form
The point-slope form of a linear equation is expressed as y - y1 = m(x - x1), where (x1, y1) is a specific point on the line and m is the slope. This form is particularly useful for writing the equation of a line when you know a point on the line and its slope, allowing for straightforward calculations in this problem.
Recommended video:
Guided course

Slope-Intercept Form

 5:57m
5:57mWatch next
Master Graphs of Common Functions with a bite sized video explanation from Nick
Start learningRelated Videos
Related Practice