Table of contents
- 0. Functions7h 52m
- Introduction to Functions16m
- Piecewise Functions10m
- Properties of Functions9m
- Common Functions1h 8m
- Transformations5m
- Combining Functions27m
- Exponent rules32m
- Exponential Functions28m
- Logarithmic Functions24m
- Properties of Logarithms34m
- Exponential & Logarithmic Equations35m
- Introduction to Trigonometric Functions38m
- Graphs of Trigonometric Functions44m
- Trigonometric Identities47m
- Inverse Trigonometric Functions48m
- 1. Limits and Continuity2h 2m
- 2. Intro to Derivatives1h 33m
- 3. Techniques of Differentiation3h 18m
- 4. Applications of Derivatives2h 38m
- 5. Graphical Applications of Derivatives6h 2m
- 6. Derivatives of Inverse, Exponential, & Logarithmic Functions2h 37m
- 7. Antiderivatives & Indefinite Integrals1h 26m
0. Functions
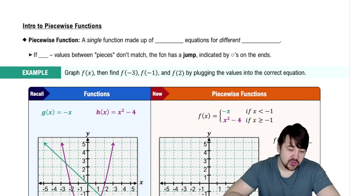
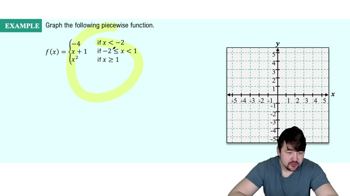
Piecewise Functions
Problem 31
Textbook Question
Piecewise linear functions Graph the following functions.
 Verified step by step guidance
Verified step by step guidance1
Step 1: Understand the piecewise function definition. The function \( f(x) \) is defined as \( 3x - 1 \) for \( x \leq 0 \) and \( -2x - 1 \) for \( x > 0 \).
Step 2: Graph the first piece of the function, \( 3x - 1 \), for \( x \leq 0 \). This is a linear function with a slope of 3 and a y-intercept of -1. Plot the line starting from the y-intercept at (0, -1) and extend it to the left.
Step 3: Graph the second piece of the function, \( -2x - 1 \), for \( x > 0 \). This is a linear function with a slope of -2 and a y-intercept of -1. Plot the line starting from the y-intercept at (0, -1) and extend it to the right.
Step 4: Consider the point where the two pieces meet at \( x = 0 \). For \( x = 0 \), the value from the first piece is \( 3(0) - 1 = -1 \). Since the condition is \( x \leq 0 \), the point (0, -1) is included in the graph.
Step 5: Combine the two pieces on the graph. The graph will have a solid point at (0, -1) for the first piece and an open point at (0, -1) for the second piece, indicating that the second piece does not include \( x = 0 \).
Recommended similar problem, with video answer:
 Verified Solution
Verified SolutionThis video solution was recommended by our tutors as helpful for the problem above
Video duration:
7mPlay a video:
Was this helpful?
Related Videos
Related Practice