Determine whether the following statements are true and give an explanation or counterexample.


a. The graph of a function can never cross one of its horizontal asymptotes.

Key Concepts

Horizontal Asymptotes

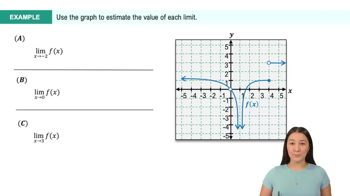
A horizontal asymptote is a line that a graph approaches as the input values (x) approach positive or negative infinity. It indicates the behavior of a function at extreme values. For example, the function f(x) = 1/x has a horizontal asymptote at y = 0, meaning as x becomes very large or very small, the function values get closer to 0.

Behavior of Functions Near Asymptotes

Functions can behave differently near their asymptotes. While a function may approach a horizontal asymptote, it is not restricted from crossing it at finite values of x. For instance, the function f(x) = sin(x)/x approaches the horizontal asymptote y = 0 as x approaches infinity, but it crosses the x-axis multiple times.

True/False Statements in Mathematics

In mathematics, determining the truth of a statement often requires understanding definitions and properties. A statement can be true in some contexts and false in others. In this case, the assertion that a function cannot cross its horizontal asymptote is false, as demonstrated by functions that do cross their asymptotes at finite points.