Table of contents
- 0. Functions7h 52m
- Introduction to Functions16m
- Piecewise Functions10m
- Properties of Functions9m
- Common Functions1h 8m
- Transformations5m
- Combining Functions27m
- Exponent rules32m
- Exponential Functions28m
- Logarithmic Functions24m
- Properties of Logarithms34m
- Exponential & Logarithmic Equations35m
- Introduction to Trigonometric Functions38m
- Graphs of Trigonometric Functions44m
- Trigonometric Identities47m
- Inverse Trigonometric Functions48m
- 1. Limits and Continuity2h 2m
- 2. Intro to Derivatives1h 33m
- 3. Techniques of Differentiation3h 18m
- 4. Applications of Derivatives2h 38m
- 5. Graphical Applications of Derivatives6h 2m
- 6. Derivatives of Inverse, Exponential, & Logarithmic Functions2h 37m
- 7. Antiderivatives & Indefinite Integrals1h 26m
- 8. Definite Integrals4h 44m
- 9. Graphical Applications of Integrals2h 27m
- 10. Physics Applications of Integrals 2h 22m
4. Applications of Derivatives
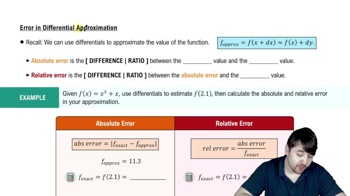
Differentials
Problem 4.R.71
Textbook Question
60–81. Limits Evaluate the following limits. Use l’Hôpital’s Rule when needed.
lim_x→0 csc x sin⁻¹ x
 Verified step by step guidance
Verified step by step guidance1
Identify the form of the limit as x approaches 0. The expression csc(x) is 1/sin(x), and sin⁻¹(x) is the inverse sine function. As x approaches 0, both sin(x) and sin⁻¹(x) approach 0, leading to an indeterminate form of 0/0.
Apply l'Hôpital's Rule, which is used to evaluate limits of indeterminate forms like 0/0 or ∞/∞. According to l'Hôpital's Rule, take the derivative of the numerator and the derivative of the denominator separately.
Differentiate the numerator: The derivative of csc(x) is -csc(x)cot(x).
Differentiate the denominator: The derivative of sin⁻¹(x) is 1/√(1-x²).
Re-evaluate the limit using the derivatives: Substitute the derivatives back into the limit expression and evaluate the limit as x approaches 0. Simplify the expression if necessary to find the limit.
 Verified video answer for a similar problem:
Verified video answer for a similar problem:This video solution was recommended by our tutors as helpful for the problem above
Video duration:
5mPlay a video:
Was this helpful?
Key Concepts
Here are the essential concepts you must grasp in order to answer the question correctly.
Limits
Limits are fundamental in calculus, representing the value that a function approaches as the input approaches a certain point. They are essential for understanding continuity, derivatives, and integrals. In this context, evaluating the limit as x approaches 0 requires analyzing the behavior of the function near that point.
Recommended video:

One-Sided Limits
L'Hôpital's Rule
L'Hôpital's Rule is a method for evaluating limits that result in indeterminate forms, such as 0/0 or ∞/∞. It states that if the limit of f(x)/g(x) leads to an indeterminate form, the limit can be found by taking the derivative of the numerator and the derivative of the denominator. This rule simplifies the process of finding limits in complex expressions.
Recommended video:
Guided course
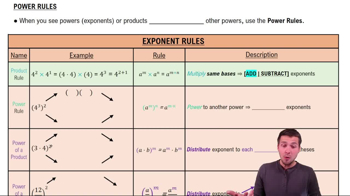
Power Rules
Cosecant and Inverse Sine Functions
The cosecant function, csc(x), is the reciprocal of the sine function, defined as csc(x) = 1/sin(x). The inverse sine function, sin⁻¹(x), returns the angle whose sine is x. Understanding these functions is crucial for evaluating the limit in the question, as they interact in a way that may lead to an indeterminate form, necessitating the use of L'Hôpital's Rule.
Recommended video:

Inverse Sine