Table of contents
- 0. Functions7h 52m
- Introduction to Functions16m
- Piecewise Functions10m
- Properties of Functions9m
- Common Functions1h 8m
- Transformations5m
- Combining Functions27m
- Exponent rules32m
- Exponential Functions28m
- Logarithmic Functions24m
- Properties of Logarithms34m
- Exponential & Logarithmic Equations35m
- Introduction to Trigonometric Functions38m
- Graphs of Trigonometric Functions44m
- Trigonometric Identities47m
- Inverse Trigonometric Functions48m
- 1. Limits and Continuity2h 2m
- 2. Intro to Derivatives1h 33m
- 3. Techniques of Differentiation3h 18m
- 4. Applications of Derivatives2h 38m
- 5. Graphical Applications of Derivatives6h 2m
- 6. Derivatives of Inverse, Exponential, & Logarithmic Functions2h 37m
- 7. Antiderivatives & Indefinite Integrals1h 26m
1. Limits and Continuity
Introduction to Limits
Problem 51a
Textbook Question
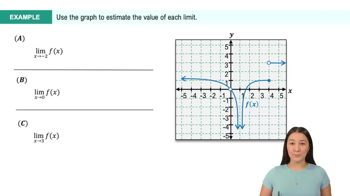
Analyze the following limits. Then sketch a graph of y=tanx with the window [−π,π]×[−10,10]and use your graph to check your work.
lim x→π/2^+ tan x
 Verified step by step guidance
Verified step by step guidance1
Step 1: Understand the behavior of the tangent function. The function \( y = \tan x \) has vertical asymptotes where the cosine of \( x \) is zero, which occurs at \( x = \frac{\pi}{2} + k\pi \) for any integer \( k \).
Step 2: Identify the limit direction. The limit \( \lim_{x \to \frac{\pi}{2}^+} \tan x \) means we are approaching \( \frac{\pi}{2} \) from the right side, or from values slightly greater than \( \frac{\pi}{2} \).
Step 3: Analyze the behavior of \( \tan x \) as \( x \to \frac{\pi}{2}^+ \). As \( x \) approaches \( \frac{\pi}{2} \) from the right, \( \tan x \) tends to increase without bound because the cosine of \( x \) approaches zero from the positive side, making \( \tan x = \frac{\sin x}{\cos x} \) approach positive infinity.
Step 4: Sketch the graph of \( y = \tan x \) over the interval \([-\pi, \pi]\). Note the vertical asymptotes at \( x = -\frac{\pi}{2} \) and \( x = \frac{\pi}{2} \), and the periodic nature of the tangent function with period \( \pi \).
Step 5: Use the graph to verify the limit. On the graph, observe that as \( x \) approaches \( \frac{\pi}{2} \) from the right, the value of \( \tan x \) increases towards positive infinity, confirming the limit analysis.
Recommended similar problem, with video answer:
 Verified Solution
Verified SolutionThis video solution was recommended by our tutors as helpful for the problem above
Video duration:
1mPlay a video:
Was this helpful?
Key Concepts
Here are the essential concepts you must grasp in order to answer the question correctly.
Limits
A limit is a fundamental concept in calculus that describes the behavior of a function as its input approaches a certain value. In this case, we are interested in the limit of the tangent function as x approaches π/2 from the right. Understanding limits helps in analyzing the continuity and behavior of functions, especially at points where they may not be defined.
Recommended video:

One-Sided Limits
Tangent Function
The tangent function, denoted as tan(x), is a periodic function defined as the ratio of the sine and cosine functions: tan(x) = sin(x)/cos(x). It has vertical asymptotes where the cosine function is zero, such as at x = π/2, leading to undefined values. Recognizing the properties of the tangent function is crucial for analyzing its limits and graphing its behavior.
Recommended video:
Slopes of Tangent Lines
Graphing and Asymptotes
Graphing functions involves plotting their values on a coordinate system to visualize their behavior. For the tangent function, it is essential to identify vertical asymptotes, which occur at points where the function approaches infinity. In this case, as x approaches π/2 from the right, the graph of y = tan(x) will rise steeply, illustrating the concept of limits and the function's undefined nature at that point.
Recommended video:

Introduction to Cotangent Graph

 6:47m
6:47mWatch next
Master Finding Limits Numerically and Graphically with a bite sized video explanation from Callie
Start learning