Table of contents
- 0. Functions7h 52m
- Introduction to Functions16m
- Piecewise Functions10m
- Properties of Functions9m
- Common Functions1h 8m
- Transformations5m
- Combining Functions27m
- Exponent rules32m
- Exponential Functions28m
- Logarithmic Functions24m
- Properties of Logarithms34m
- Exponential & Logarithmic Equations35m
- Introduction to Trigonometric Functions38m
- Graphs of Trigonometric Functions44m
- Trigonometric Identities47m
- Inverse Trigonometric Functions48m
- 1. Limits and Continuity2h 2m
- 2. Intro to Derivatives1h 33m
- 3. Techniques of Differentiation3h 18m
- 4. Applications of Derivatives2h 38m
- 5. Graphical Applications of Derivatives6h 2m
- 6. Derivatives of Inverse, Exponential, & Logarithmic Functions2h 37m
- 7. Antiderivatives & Indefinite Integrals1h 26m
- 8. Definite Integrals4h 44m
- 9. Graphical Applications of Integrals2h 27m
- 10. Physics Applications of Integrals 2h 22m
5. Graphical Applications of Derivatives
Intro to Extrema
Problem 4.1.89a
Textbook Question
Values of related functions Suppose f is differentiable on (-∞,∞) and assume it has a local extreme value at the point x = 2, where f(2) = 0. Let g(x) = xf(x) + 1 and let h(x) = xf(x) + x +1, for all values of x.
a. Evaluate g(2), h(2), g'(2), and h'(2).
 Verified step by step guidance
Verified step by step guidance1
First, evaluate g(2) by substituting x = 2 into the function g(x) = xf(x) + 1. Since f(2) = 0, g(2) = 2 * f(2) + 1 = 2 * 0 + 1 = 1.
Next, evaluate h(2) by substituting x = 2 into the function h(x) = xf(x) + x + 1. Again, since f(2) = 0, h(2) = 2 * f(2) + 2 + 1 = 0 + 2 + 1 = 3.
To find g'(x), use the product rule for differentiation. The function g(x) = xf(x) + 1 can be differentiated as g'(x) = f(x) + x * f'(x). Substitute x = 2 to find g'(2).
For h'(x), differentiate h(x) = xf(x) + x + 1. Using the product rule and the sum rule, h'(x) = f(x) + x * f'(x) + 1. Substitute x = 2 to find h'(2).
Since f has a local extreme value at x = 2, f'(2) = 0. Use this information to simplify g'(2) and h'(2) further by substituting f'(2) = 0 into the expressions obtained in the previous steps.
 Verified video answer for a similar problem:
Verified video answer for a similar problem:This video solution was recommended by our tutors as helpful for the problem above
Video duration:
6mPlay a video:
Was this helpful?
Key Concepts
Here are the essential concepts you must grasp in order to answer the question correctly.
Differentiability and Local Extrema
A function is differentiable at a point if it has a defined derivative there, which implies it is smooth and has no sharp corners. A local extreme value occurs at a point where the function reaches a maximum or minimum compared to nearby points. For a differentiable function, local extrema can be identified using the first derivative test, where the derivative changes sign around the point.
Recommended video:
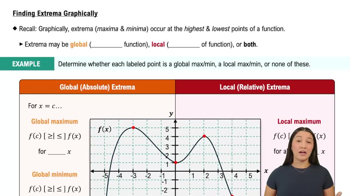
Finding Extrema Graphically
Function Evaluation
Function evaluation involves substituting a specific value into a function to determine its output. For example, to evaluate g(2) or h(2), we substitute x = 2 into the respective function definitions. This process is essential for finding specific values of functions at given points, which is a fundamental skill in calculus.
Recommended video:
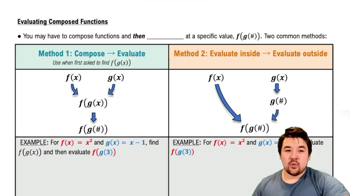
Evaluating Composed Functions
Derivative of a Product
The derivative of a product of two functions can be found using the product rule, which states that if u(x) and v(x) are functions, then the derivative of their product u(x)v(x) is u'(x)v(x) + u(x)v'(x). This rule is crucial when differentiating functions like g(x) and h(x), which are defined as products of x and f(x), allowing us to compute g'(2) and h'(2) effectively.
Recommended video:
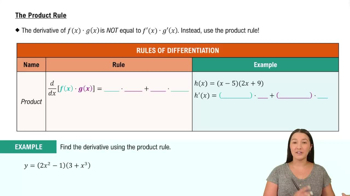
The Product Rule

 5:58m
5:58mWatch next
Master Finding Extrema Graphically with a bite sized video explanation from Callie
Start learning




