Table of contents
- 0. Functions7h 52m
- Introduction to Functions16m
- Piecewise Functions10m
- Properties of Functions9m
- Common Functions1h 8m
- Transformations5m
- Combining Functions27m
- Exponent rules32m
- Exponential Functions28m
- Logarithmic Functions24m
- Properties of Logarithms34m
- Exponential & Logarithmic Equations35m
- Introduction to Trigonometric Functions38m
- Graphs of Trigonometric Functions44m
- Trigonometric Identities47m
- Inverse Trigonometric Functions48m
- 1. Limits and Continuity2h 2m
- 2. Intro to Derivatives1h 33m
- 3. Techniques of Differentiation3h 18m
- 4. Applications of Derivatives2h 38m
- 5. Graphical Applications of Derivatives6h 2m
- 6. Derivatives of Inverse, Exponential, & Logarithmic Functions2h 37m
- 7. Antiderivatives & Indefinite Integrals1h 26m
- 8. Definite Integrals3h 25m
0. Functions
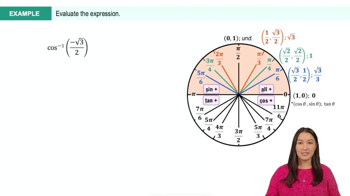
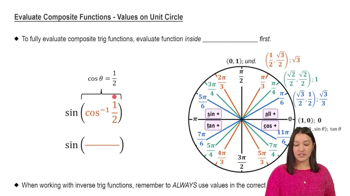
Inverse Trigonometric Functions
Problem 3.10.65a
Textbook Question
62–65. {Use of Tech} Graphing f and f'
a. Graph f with a graphing utility.
f(x)=e^−x tan^−1 x on [0,∞)
 Verified step by step guidance
Verified step by step guidance1
Start by defining the function f(x) = e^{-x} an^{-1}(x). This function combines an exponential decay component and the arctangent function, which will influence its behavior on the interval [0, ∞).
Use a graphing utility to plot the function f(x) over the interval [0, ∞). Make sure to set appropriate limits for the x-axis and y-axis to capture the behavior of the function as x approaches infinity.
Next, calculate the derivative f'(x) using the product and chain rules. The derivative will help you understand the rate of change of the function and identify critical points.
Once you have f'(x), graph this derivative function alongside f(x) on the same interval. This will allow you to compare the original function with its rate of change.
Analyze the graphs of f(x) and f'(x) to identify key features such as local maxima, minima, and points of inflection, which will provide insights into the behavior of the function.
Was this helpful?
Related Videos
Related Practice