Table of contents
- 0. Functions7h 52m
- Introduction to Functions16m
- Piecewise Functions10m
- Properties of Functions9m
- Common Functions1h 8m
- Transformations5m
- Combining Functions27m
- Exponent rules32m
- Exponential Functions28m
- Logarithmic Functions24m
- Properties of Logarithms34m
- Exponential & Logarithmic Equations35m
- Introduction to Trigonometric Functions38m
- Graphs of Trigonometric Functions44m
- Trigonometric Identities47m
- Inverse Trigonometric Functions48m
- 1. Limits and Continuity2h 2m
- 2. Intro to Derivatives1h 33m
- 3. Techniques of Differentiation3h 18m
- 4. Applications of Derivatives2h 38m
- 5. Graphical Applications of Derivatives6h 2m
- 6. Derivatives of Inverse, Exponential, & Logarithmic Functions2h 37m
- 7. Antiderivatives & Indefinite Integrals1h 26m
- 8. Definite Integrals4h 44m
- 9. Graphical Applications of Integrals2h 27m
- 10. Physics Applications of Integrals 2h 22m
5. Graphical Applications of Derivatives
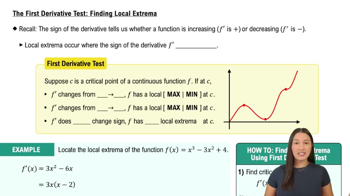
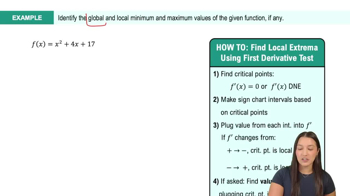
The First Derivative Test
Struggling with Calculus?
Join thousands of students who trust us to help them ace their exams!Watch the first videoMultiple Choice
Identify the open intervals on which the function is increasing or decreasing.
f(x)=3x4+8x3−18x2+7
A
Increasing on (−∞,−3)&(0,1), Decreasing on (−3,0)&(1,∞)
B
Increasing on (−3,0)&(1,∞), Decreasing on (−∞,−3)&(0,1)
C
Increasing on (−∞,0), Decreasing on (0,∞)
D
Increasing on (0,∞), Decreasing on (−∞,0)
 Verified step by step guidance
Verified step by step guidance1
To determine where the function is increasing or decreasing, we first need to find its derivative. The function given is \( f(x) = 3x^4 + 8x^3 - 18x^2 + 7 \).
Calculate the derivative \( f'(x) \) using the power rule. The power rule states that the derivative of \( ax^n \) is \( n \cdot ax^{n-1} \). Apply this to each term of the function.
The derivative is \( f'(x) = 12x^3 + 24x^2 - 36x \).
Find the critical points by setting \( f'(x) = 0 \) and solving for \( x \). This involves factoring the derivative or using the quadratic formula if necessary.
Once the critical points are found, use a sign chart or test intervals around these points to determine where \( f'(x) \) is positive (indicating the function is increasing) or negative (indicating the function is decreasing). This will help identify the open intervals where the function is increasing or decreasing.

 7:32m
7:32mWatch next
Master Determining Where a Function is Increasing & Decreasing with a bite sized video explanation from Callie
Start learningRelated Videos
Related Practice