Table of contents
- 0. Functions7h 52m
- Introduction to Functions16m
- Piecewise Functions10m
- Properties of Functions9m
- Common Functions1h 8m
- Transformations5m
- Combining Functions27m
- Exponent rules32m
- Exponential Functions28m
- Logarithmic Functions24m
- Properties of Logarithms34m
- Exponential & Logarithmic Equations35m
- Introduction to Trigonometric Functions38m
- Graphs of Trigonometric Functions44m
- Trigonometric Identities47m
- Inverse Trigonometric Functions48m
- 1. Limits and Continuity2h 2m
- 2. Intro to Derivatives1h 33m
- 3. Techniques of Differentiation3h 18m
- 4. Applications of Derivatives2h 38m
- 5. Graphical Applications of Derivatives6h 2m
- 6. Derivatives of Inverse, Exponential, & Logarithmic Functions2h 37m
- 7. Antiderivatives & Indefinite Integrals1h 26m
- 8. Definite Integrals3h 25m
2. Intro to Derivatives
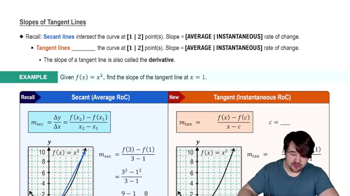
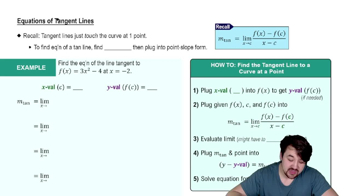
Tangent Lines and Derivatives
Problem 99b
Textbook Question
The population of the United States (in millions) by decade is given in the table, where t is the number of years after 1910. These data are plotted and fitted with a smooth curve y = p(t) in the figure. <IMAGE><IMAGE>
Explain why the average rate of growth from 1950 to 1960 is a good approximation to the (instantaneous) rate of growth in 1955.
 Verified step by step guidance
Verified step by step guidance1
Identify the population values at the beginning and end of the interval from 1950 to 1960, which corresponds to t = 40 and t = 50 respectively, using the provided data.
Calculate the average rate of growth over the interval by using the formula for average rate of change: \( \text{Average Rate} = \frac{p(50) - p(40)}{50 - 40} \).
Understand that the average rate of growth over a small interval can serve as an approximation for the instantaneous rate of growth at a point within that interval, in this case, at t = 45 (which corresponds to the year 1955).
Discuss the concept of continuity and differentiability of the population function \( p(t) \), which implies that if the function is smooth, the average rate of change will closely approximate the instantaneous rate of change at points within the interval.
Conclude that since the interval from 1950 to 1960 is relatively small and the population function is likely smooth, the average rate of growth calculated provides a good estimate for the instantaneous rate of growth at t = 45.
Recommended similar problem, with video answer:
 Verified Solution
Verified SolutionThis video solution was recommended by our tutors as helpful for the problem above
Video duration:
3mPlay a video:
Was this helpful?

 5:13m
5:13mWatch next
Master Slopes of Tangent Lines with a bite sized video explanation from Nick
Start learningRelated Videos
Related Practice