Table of contents
- 0. Functions7h 52m
- Introduction to Functions16m
- Piecewise Functions10m
- Properties of Functions9m
- Common Functions1h 8m
- Transformations5m
- Combining Functions27m
- Exponent rules32m
- Exponential Functions28m
- Logarithmic Functions24m
- Properties of Logarithms34m
- Exponential & Logarithmic Equations35m
- Introduction to Trigonometric Functions38m
- Graphs of Trigonometric Functions44m
- Trigonometric Identities47m
- Inverse Trigonometric Functions48m
- 1. Limits and Continuity2h 2m
- 2. Intro to Derivatives1h 33m
- 3. Techniques of Differentiation3h 18m
- 4. Applications of Derivatives2h 38m
- 5. Graphical Applications of Derivatives6h 2m
- 6. Derivatives of Inverse, Exponential, & Logarithmic Functions2h 37m
- 7. Antiderivatives & Indefinite Integrals1h 26m
- 8. Definite Integrals4h 44m
- 9. Graphical Applications of Integrals2h 27m
- 10. Physics Applications of Integrals 2h 22m
3. Techniques of Differentiation
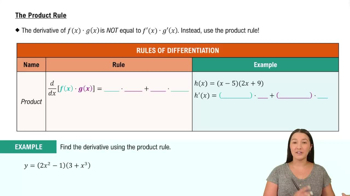
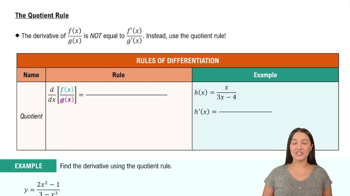
Product and Quotient Rules
Problem 43
Textbook Question
Derivative calculations Evaluate the derivative of the following functions at the given point.
f(t) = 1/t+1; a=1
 Verified step by step guidance
Verified step by step guidance1
Step 1: Identify the function f(t) = \frac{1}{t} + 1 and the point a = 1 where we need to evaluate the derivative.
Step 2: Rewrite the function in a form that is easier to differentiate: f(t) = t^{-1} + 1.
Step 3: Differentiate the function using the power rule. The derivative of t^{-1} is -t^{-2}, and the derivative of a constant (1) is 0.
Step 4: Combine the results from the differentiation: f'(t) = -t^{-2}.
Step 5: Evaluate the derivative at the given point a = 1: f'(1) = -1^{-2}.
 Verified video answer for a similar problem:
Verified video answer for a similar problem:This video solution was recommended by our tutors as helpful for the problem above
Video duration:
3mPlay a video:
Was this helpful?
Key Concepts
Here are the essential concepts you must grasp in order to answer the question correctly.
Derivative
The derivative of a function measures how the function's output value changes as its input value changes. It is defined as the limit of the average rate of change of the function over an interval as the interval approaches zero. In practical terms, the derivative provides the slope of the tangent line to the function's graph at a given point.
Recommended video:

Derivatives
Limit
A limit is a fundamental concept in calculus that describes the behavior of a function as its input approaches a certain value. It is essential for defining derivatives and integrals. Understanding limits allows us to analyze functions that may not be well-defined at specific points, enabling the calculation of derivatives even when direct substitution is not possible.
Recommended video:

One-Sided Limits
Function Evaluation
Function evaluation involves substituting a specific value into a function to determine its output. In the context of derivatives, evaluating the function at a particular point is crucial for finding the derivative at that point. This process often requires simplifying the function and applying the rules of calculus to derive the correct output.
Recommended video:
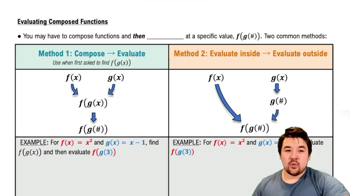
Evaluating Composed Functions
Related Videos
Related Practice