Table of contents
- 0. Functions7h 52m
- Introduction to Functions16m
- Piecewise Functions10m
- Properties of Functions9m
- Common Functions1h 8m
- Transformations5m
- Combining Functions27m
- Exponent rules32m
- Exponential Functions28m
- Logarithmic Functions24m
- Properties of Logarithms34m
- Exponential & Logarithmic Equations35m
- Introduction to Trigonometric Functions38m
- Graphs of Trigonometric Functions44m
- Trigonometric Identities47m
- Inverse Trigonometric Functions48m
- 1. Limits and Continuity2h 2m
- 2. Intro to Derivatives1h 33m
- 3. Techniques of Differentiation3h 18m
- 4. Applications of Derivatives2h 38m
- 5. Graphical Applications of Derivatives6h 2m
- 6. Derivatives of Inverse, Exponential, & Logarithmic Functions2h 37m
- 7. Antiderivatives & Indefinite Integrals1h 26m
- 8. Definite Integrals3h 25m
5. Graphical Applications of Derivatives
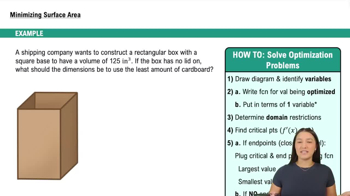
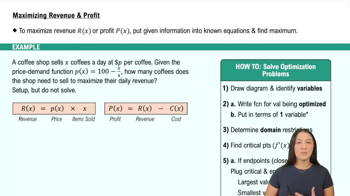
Applied Optimization
Problem 4.R.37
Textbook Question
{Use of Tech } Minimizing sound intensity Two sound speakers are 100 m apart and one speaker is three times as loud as the other speaker. At what point on a line segment between the speakers is the sound intensity the weakest? (Hint: Sound intensity is directly proportional to the sound level and inversely proportional to the square of the distance from the sound source.)
 Verified step by step guidance
Verified step by step guidance1
Define the variables: Let the intensity of the quieter speaker be I and the louder speaker be 3I, where I is the intensity of the quieter speaker.
Set up the distance: Let x be the distance from the quieter speaker to the point where the sound intensity is being measured. Therefore, the distance from the louder speaker to this point is (100 - x) meters.
Write the expression for sound intensity: The total sound intensity at point x can be expressed as I_total = I / x^2 + 3I / (100 - x)^2.
To find the minimum intensity, take the derivative of I_total with respect to x and set it equal to zero to find critical points: d(I_total)/dx = 0.
Analyze the critical points and endpoints to determine where the sound intensity is minimized, ensuring to check the second derivative or use the first derivative test for confirmation.
Was this helpful?

 1:13m
1:13mWatch next
Master Intro to Applied Optimization: Maximizing Area with a bite sized video explanation from Callie
Start learningRelated Videos
Related Practice