Table of contents
- 0. Functions7h 52m
- Introduction to Functions16m
- Piecewise Functions10m
- Properties of Functions9m
- Common Functions1h 8m
- Transformations5m
- Combining Functions27m
- Exponent rules32m
- Exponential Functions28m
- Logarithmic Functions24m
- Properties of Logarithms34m
- Exponential & Logarithmic Equations35m
- Introduction to Trigonometric Functions38m
- Graphs of Trigonometric Functions44m
- Trigonometric Identities47m
- Inverse Trigonometric Functions48m
- 1. Limits and Continuity2h 2m
- 2. Intro to Derivatives1h 33m
- 3. Techniques of Differentiation3h 18m
- 4. Applications of Derivatives2h 38m
- 5. Graphical Applications of Derivatives6h 2m
- 6. Derivatives of Inverse, Exponential, & Logarithmic Functions2h 37m
- 7. Antiderivatives & Indefinite Integrals1h 26m
- 8. Definite Integrals4h 44m
- 9. Graphical Applications of Integrals2h 27m
- 10. Physics Applications of Integrals 2h 22m
0. Functions
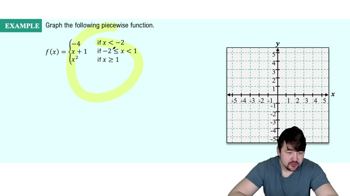
Piecewise Functions
Problem 1.31
Textbook Question
Graphing functions Sketch a graph of each function.
g(x) = { 4-2x if x ≤ 1 , (x-1)² + 2 if x > 1
 Verified step by step guidance
Verified step by step guidance1
Step 1: Identify the piecewise function. The function g(x) is defined in two parts: g(x) = 4 - 2x for x ≤ 1 and g(x) = (x - 1)^2 + 2 for x > 1.
Step 2: Analyze the first piece of the function, g(x) = 4 - 2x for x ≤ 1. This is a linear function with a slope of -2 and a y-intercept of 4. Sketch this line for x-values less than or equal to 1.
Step 3: Analyze the second piece of the function, g(x) = (x - 1)^2 + 2 for x > 1. This is a quadratic function, specifically a parabola that opens upwards with its vertex at (1, 2). Sketch this parabola for x-values greater than 1.
Step 4: Determine the point of transition at x = 1. For the first piece, when x = 1, g(x) = 4 - 2(1) = 2. For the second piece, as x approaches 1 from the right, g(x) = (1 - 1)^2 + 2 = 2. Both pieces meet at the point (1, 2), ensuring continuity at x = 1.
Step 5: Combine the sketches of both pieces. Draw the linear part for x ≤ 1 and the quadratic part for x > 1, ensuring they connect smoothly at the point (1, 2).
 Verified video answer for a similar problem:
Verified video answer for a similar problem:This video solution was recommended by our tutors as helpful for the problem above
Video duration:
11mPlay a video:
Was this helpful?
Key Concepts
Here are the essential concepts you must grasp in order to answer the question correctly.
Piecewise Functions
A piecewise function is defined by different expressions based on the input value. In this case, g(x) has two distinct formulas: one for x values less than or equal to 1, and another for x values greater than 1. Understanding how to evaluate and graph each piece separately is crucial for accurately representing the entire function.
Recommended video:
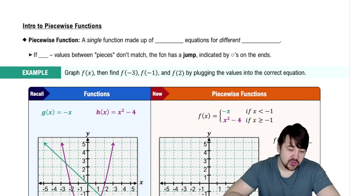
Piecewise Functions
Graphing Techniques
Graphing techniques involve plotting points and understanding the behavior of functions. For piecewise functions, it is important to identify key points, such as where the function changes from one expression to another, and to ensure continuity or discontinuity at those points. This helps in creating an accurate visual representation of the function.
Recommended video:

Graphing The Derivative
Continuity and Discontinuity
Continuity refers to a function being unbroken at a point, meaning the left-hand limit, right-hand limit, and the function value at that point are all equal. In the context of g(x), checking for continuity at x = 1 is essential, as it determines whether the graph has a jump or is smooth at that transition point.
Recommended video:

Intro to Continuity
Related Videos
Related Practice