Table of contents
- 0. Functions7h 52m
- Introduction to Functions16m
- Piecewise Functions10m
- Properties of Functions9m
- Common Functions1h 8m
- Transformations5m
- Combining Functions27m
- Exponent rules32m
- Exponential Functions28m
- Logarithmic Functions24m
- Properties of Logarithms34m
- Exponential & Logarithmic Equations35m
- Introduction to Trigonometric Functions38m
- Graphs of Trigonometric Functions44m
- Trigonometric Identities47m
- Inverse Trigonometric Functions48m
- 1. Limits and Continuity2h 2m
- 2. Intro to Derivatives1h 33m
- 3. Techniques of Differentiation3h 18m
- 4. Applications of Derivatives2h 38m
- 5. Graphical Applications of Derivatives6h 2m
- 6. Derivatives of Inverse, Exponential, & Logarithmic Functions2h 37m
- 7. Antiderivatives & Indefinite Integrals1h 26m
- 8. Definite Integrals4h 44m
- 9. Graphical Applications of Integrals2h 27m
- 10. Physics Applications of Integrals 2h 22m
5. Graphical Applications of Derivatives
Applied Optimization
Problem 4.R.44
Textbook Question
Minimum painting surface A metal cistern in the shape of a right circular cylinder with volume V = 50 m³ needs to be painted each year to reduce corrosion. The paint is applied only to surfaces exposed to the elements (the outside cylinder wall and the circular top). Find the dimensions r and h of the cylinder that minimize the area of the painted surfaces.
 Verified step by step guidance
Verified step by step guidance1
Start by identifying the formulas needed: The volume of a cylinder is given by \( V = \pi r^2 h \) and the surface area to be painted (the lateral surface area plus the top) is \( A = 2\pi r h + \pi r^2 \).
Since the volume \( V = 50 \) m³ is given, express the height \( h \) in terms of the radius \( r \) using the volume formula: \( h = \frac{V}{\pi r^2} = \frac{50}{\pi r^2} \).
Substitute \( h \) from the previous step into the surface area formula to express \( A \) solely in terms of \( r \): \( A(r) = 2\pi r \left(\frac{50}{\pi r^2}\right) + \pi r^2 \). Simplify this expression.
Differentiate the simplified surface area function \( A(r) \) with respect to \( r \) to find \( A'(r) \). This will help identify the critical points where the surface area could be minimized.
Set the derivative \( A'(r) \) equal to zero and solve for \( r \) to find the critical points. Use the second derivative test or analyze the behavior of \( A'(r) \) to confirm that the critical point corresponds to a minimum surface area. Once \( r \) is found, use the expression for \( h \) to find the corresponding height.
 Verified video answer for a similar problem:
Verified video answer for a similar problem:This video solution was recommended by our tutors as helpful for the problem above
Video duration:
7mPlay a video:
Was this helpful?
Key Concepts
Here are the essential concepts you must grasp in order to answer the question correctly.
Volume of a Cylinder
The volume of a right circular cylinder is calculated using the formula V = πr²h, where r is the radius and h is the height. In this problem, the volume is fixed at 50 m³, which means that any solution must satisfy this equation. Understanding how to manipulate this formula is essential for relating the dimensions of the cylinder to its volume.
Recommended video:

Example 5: Packaging Design
Surface Area of a Cylinder
The surface area of a right circular cylinder consists of the lateral area and the area of the circular top. The formula for the total surface area A is A = 2πrh + πr², where the first term represents the lateral surface area and the second term accounts for the top. Minimizing this surface area while maintaining a constant volume is the core objective of the problem.
Recommended video:
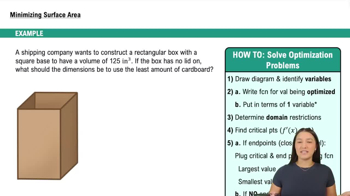
Example 1: Minimizing Surface Area
Optimization Techniques
Optimization in calculus involves finding the maximum or minimum values of a function. In this context, we will use techniques such as setting up a function for the surface area in terms of one variable (using the volume constraint) and applying derivatives to find critical points. Understanding how to apply the first and second derivative tests is crucial for determining the dimensions that minimize the painted surface area.
Recommended video:

Intro to Applied Optimization: Maximizing Area

 1:13m
1:13mWatch next
Master Intro to Applied Optimization: Maximizing Area with a bite sized video explanation from Callie
Start learningRelated Videos
Related Practice