Optimal popcorn box A small popcorn box is created from a 12" ...

Question

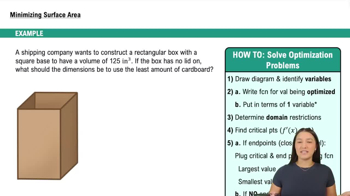
Optimal popcorn box A small popcorn box is created from a 12" x 12" sheet of paperboard by first cutting out four shaded rectangles, each of length x and width x/2 (see figure). The remaining paperboard is folded along the solid lines to form a box. What dimensions of the box maximize the volume of the box?

Accepted Answer

Welcome back, everyone. In this problem, a company designs an open top box by cutting out squares from each corner of a 20 inch by 20 inch cardboard sheet. The squares have a side length of 10 inches. To maximize the volume of the resulting box when the sides are folded up, what should the dimensions of the box be? No. To solve this problem, let's first try to visualize what's going on here. So we're saying that we're cutting out squares from a square box, a 20 inch or a square sheet, a 20 inch by 20 inch cardboard sheet. So let's say that this is our sheet, OK. And let's cut out our square corners. And now, we know that as our problem told us, these squares are supposed to have a side length of X inches. OK. So, uh let's put in our dimensions here. X and X for each. Dimension of our square corner, OK? Now from this we're supposed to maximize the volume of the resulting box, but before we can think about the volume, we'll need to think about the dimensions of our box. Now we know that the resulting flaps are folded upward to form the sides of the open top box. So for our box, we know that its height H is going to be equal to X. Now when it comes to the length and the width, if we think about it here. Since this is going to be the size of our box, let's say that this is the length and this is our width. Not that it would be equal to the entire length of the sheet 20 minus X minus X. In other words, L equals 20 minus 2 X. And since it's a square, that will be the same case for the width. OK, so L equals W, which equals 20 minus 2 X. So if that's the case, Then no, this means then that the volume of our box, which equals the length multiplied by the width multiplied by the height. Is going to be equal to, OK, 20 minus 2 X multiplied by 20 minus 2 X multiplied by our height, which is X. I know when we work that out, then we should get our volume as a function of X to be equal to 400 X. Minus 80 X squared. OK. Plus 4 XQ. Now that we have our volume as a function of X, we can maximize the volume, OK? And that resulting value, after we've maximized, is going to be, is going to help us to find our dimensions, L, W, and H. So let's maximize V of X. And now to maximize our volume, we know that essentially it means that we're going to find the first derivative of VX, set it to 0, solve for the value of X, and test whether this value is the maximum. And if it is, we can use it to figure out the rest of the dimensions. Now the first derivative of VFX. Let me put this in red, OK. First derivative of VF X is going to be equal to 400. OK, for about 400 X is 400 minus 160 X, OK, plus 12 x squared. And now this is what we're going to set to 0. Now, if we divide through our our expression our our equation here, sorry, uh by 4. Then we should find that we get 100 minus 40 X plus 3X squad to be equal to 0. Now, in that case, we can then factorize, use our quadratic factorization to get 3 x minus 10 multiplied by X minus 10 to be equal to 0 and no in that case, then we should get X to be equal to 10/3, OK, or X should be equal to 10. So either 10/3 of an inch or 10/3 inches or 10 inches. Now, if we think about it, when X equals 10, if we go back to our dimensions, remember that our length and our width are 20 minus 2 X. 20 minus 2 multiplied by 10 equals 0, so that would make our length and width 0, but that's not possible. So let's only consider where X is 10/3 of an inch, OK? So we know this is a critical point. Now we need to determine if it corresponds to a maximum. So testing or confirming whether. Confirming whether X equals 10/3 of an inch corresponds. To a maximum OK. To do that We can use the 2nd derivative test, OK? Using the second derivative test, and basically the second derivative test tells us that if the second derivative is negative, the function is concave done, and as such, that means the value corresponds to a maximum. Now we know our first derivative is 400 minus 160 X plus 12 X squad. So now our second derivative. Is going to be the derivative of that expression which is going to be -160 + 24 X. So now at X equals 10/3 of an inch. OK, then our second derivative. Is going to be equal to -160 plus 24 multiplied by 103. That's gonna be -160 + 80, which equals -80. 80 is less than 0, which means the function is concave down. So that tells us then that X equals 10/3 corresponds to a maximum. Now we know that it corresponds to a maximum. We can use it to find our maximum dimensions. Now remember. Now earlier we said that our height is equal to X, so that means H equals 10/3 of an inch, OK? Our box is going to be 10/3 inches tall. Next, remember that our length is equal to the width, which is equal to 20 minus 2 X. So that's 20 minus 2 multiplied by 10/3, and that is gonna be 20 minus 203 or 40/3 inches. So this tells us then that to maximize the volume of our box, its height must be 10/3 inches and its length and width must be 40/3 inches. Those have to be the dimensions. Thanks a lot for watching everyone. I hope this video helped.

Key Concepts

Volume of a Box

Optimization

Derivatives

Watch next