Table of contents
- 0. Functions7h 52m
- Introduction to Functions16m
- Piecewise Functions10m
- Properties of Functions9m
- Common Functions1h 8m
- Transformations5m
- Combining Functions27m
- Exponent rules32m
- Exponential Functions28m
- Logarithmic Functions24m
- Properties of Logarithms34m
- Exponential & Logarithmic Equations35m
- Introduction to Trigonometric Functions38m
- Graphs of Trigonometric Functions44m
- Trigonometric Identities47m
- Inverse Trigonometric Functions48m
- 1. Limits and Continuity2h 2m
- 2. Intro to Derivatives1h 33m
- 3. Techniques of Differentiation3h 18m
- 4. Applications of Derivatives2h 38m
- 5. Graphical Applications of Derivatives6h 2m
- 6. Derivatives of Inverse, Exponential, & Logarithmic Functions2h 37m
- 7. Antiderivatives & Indefinite Integrals1h 26m
- 8. Definite Integrals4h 44m
- 9. Graphical Applications of Integrals2h 27m
- 10. Physics Applications of Integrals 2h 22m
0. Functions
Combining Functions
Problem 1.R.24
Textbook Question
Evaluate and simplify the difference quotients (f(x + h) - f(x)) / h and (f(x) - f(a)) / (x - a) for each function.
f(x) = 7 / (x + 3)
 Verified step by step guidance
Verified step by step guidance1
Step 1: Identify the function f(x) = \frac{7}{x + 3}.
Step 2: For the first difference quotient, calculate f(x + h) by substituting x + h into the function: f(x + h) = \frac{7}{(x + h) + 3}.
Step 3: Substitute f(x + h) and f(x) into the first difference quotient: \frac{f(x + h) - f(x)}{h} = \frac{\frac{7}{x + h + 3} - \frac{7}{x + 3}}{h}.
Step 4: Simplify the expression from Step 3 by finding a common denominator for the fractions in the numerator: \frac{7(x + 3) - 7(x + h + 3)}{h(x + h + 3)(x + 3)}.
Step 5: For the second difference quotient, calculate f(a) by substituting a into the function: f(a) = \frac{7}{a + 3}, and then substitute f(x) and f(a) into the second difference quotient: \frac{f(x) - f(a)}{x - a} = \frac{\frac{7}{x + 3} - \frac{7}{a + 3}}{x - a}.
 Verified video answer for a similar problem:
Verified video answer for a similar problem:This video solution was recommended by our tutors as helpful for the problem above
Video duration:
5mPlay a video:
Was this helpful?
Key Concepts
Here are the essential concepts you must grasp in order to answer the question correctly.
Difference Quotient
The difference quotient is a fundamental concept in calculus that represents the average rate of change of a function over an interval. It is defined as (f(x + h) - f(x)) / h, where h is a small increment. This expression is crucial for understanding the derivative, as it approaches the instantaneous rate of change as h approaches zero.
Recommended video:
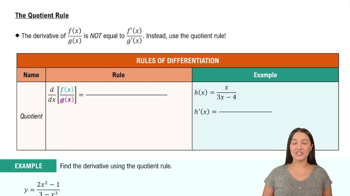
The Quotient Rule
Limit
The limit is a core concept in calculus that describes the behavior of a function as its input approaches a certain value. In the context of the difference quotient, taking the limit as h approaches zero allows us to find the derivative of a function. Limits help in analyzing the continuity and behavior of functions at specific points.
Recommended video:

One-Sided Limits
Derivative
The derivative of a function measures how the function's output changes as its input changes, representing the function's instantaneous rate of change. It is defined as the limit of the difference quotient as h approaches zero. Derivatives are essential for understanding the behavior of functions, including their slopes, tangents, and optimization problems.
Recommended video:

Derivatives

 5:56m
5:56mWatch next
Master Adding & Subtracting Functions with a bite sized video explanation from Nick
Start learning