Table of contents
- 0. Functions7h 52m
- Introduction to Functions16m
- Piecewise Functions10m
- Properties of Functions9m
- Common Functions1h 8m
- Transformations5m
- Combining Functions27m
- Exponent rules32m
- Exponential Functions28m
- Logarithmic Functions24m
- Properties of Logarithms34m
- Exponential & Logarithmic Equations35m
- Introduction to Trigonometric Functions38m
- Graphs of Trigonometric Functions44m
- Trigonometric Identities47m
- Inverse Trigonometric Functions48m
- 1. Limits and Continuity2h 2m
- 2. Intro to Derivatives1h 33m
- 3. Techniques of Differentiation3h 18m
- 4. Applications of Derivatives2h 38m
- 5. Graphical Applications of Derivatives6h 2m
- 6. Derivatives of Inverse, Exponential, & Logarithmic Functions2h 37m
- 7. Antiderivatives & Indefinite Integrals1h 26m
2. Intro to Derivatives
Basic Graphing of the Derivative
Multiple Choice
Based on the graph f(x), describe all points where the derivative f′(x) would have a jump.
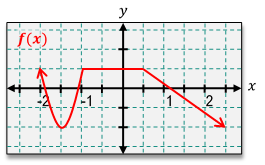
A
x=−1.5, x=−1, and x=0.5
B
x=−1 and x=0.5
C
x=−1.5
D
Derivative f′(x) has no jumps

 6:15m
6:15mWatch next
Master Graphing The Derivative with a bite sized video explanation from Nick
Start learning




