Table of contents
- 0. Functions7h 52m
- Introduction to Functions16m
- Piecewise Functions10m
- Properties of Functions9m
- Common Functions1h 8m
- Transformations5m
- Combining Functions27m
- Exponent rules32m
- Exponential Functions28m
- Logarithmic Functions24m
- Properties of Logarithms34m
- Exponential & Logarithmic Equations35m
- Introduction to Trigonometric Functions38m
- Graphs of Trigonometric Functions44m
- Trigonometric Identities47m
- Inverse Trigonometric Functions48m
- 1. Limits and Continuity2h 2m
- 2. Intro to Derivatives1h 33m
- 3. Techniques of Differentiation3h 18m
- 4. Applications of Derivatives2h 38m
- 5. Graphical Applications of Derivatives6h 2m
- 6. Derivatives of Inverse, Exponential, & Logarithmic Functions2h 37m
- 7. Antiderivatives & Indefinite Integrals1h 26m
- 8. Definite Integrals4h 44m
- 9. Graphical Applications of Integrals2h 27m
- 10. Physics Applications of Integrals 2h 22m
4. Applications of Derivatives
Implicit Differentiation
Problem 3.8.10
Textbook Question
Find the slope of the curve x²+y³=2 at each point where y=1 (see figure). <IMAGE>
 Verified step by step guidance
Verified step by step guidance1
First, understand that the problem requires finding the slope of the curve at points where y = 1. The slope of a curve at a point is given by the derivative of the curve with respect to x, which is dy/dx.
To find dy/dx, we need to differentiate the given equation implicitly. The equation is x² + y³ = 2. Differentiate both sides with respect to x. Remember that y is a function of x, so when differentiating y³, use the chain rule.
Differentiating x² with respect to x gives 2x. Differentiating y³ with respect to x gives 3y²(dy/dx) using the chain rule. The derivative of the constant 2 is 0.
Set up the equation from the differentiation: 2x + 3y²(dy/dx) = 0. Solve for dy/dx to find the slope of the curve. Rearrange the equation to isolate dy/dx: dy/dx = -2x / 3y².
Substitute y = 1 into the equation dy/dx = -2x / 3y² to find the slope at points where y = 1. This simplifies to dy/dx = -2x / 3. The slope at these points depends on the value of x, which can be found by substituting y = 1 back into the original equation to solve for x.
 Verified video answer for a similar problem:
Verified video answer for a similar problem:This video solution was recommended by our tutors as helpful for the problem above
Video duration:
3mPlay a video:
Was this helpful?
Key Concepts
Here are the essential concepts you must grasp in order to answer the question correctly.
Implicit Differentiation
Implicit differentiation is a technique used to find the derivative of a function defined implicitly by an equation involving both x and y. Instead of solving for y explicitly, we differentiate both sides of the equation with respect to x, treating y as a function of x. This allows us to find dy/dx, which represents the slope of the curve at any given point.
Recommended video:
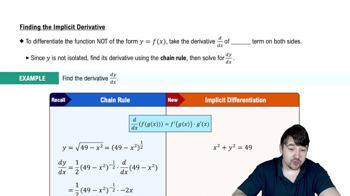
Finding The Implicit Derivative
Slope of a Curve
The slope of a curve at a given point is defined as the rate of change of the y-coordinate with respect to the x-coordinate at that point. Mathematically, it is represented by the derivative dy/dx. For a curve defined by an equation, the slope can be evaluated by substituting the coordinates of the point into the derivative obtained through implicit differentiation.
Recommended video:

Summary of Curve Sketching
Evaluating Derivatives at Specific Points
Once the derivative of the curve is found, evaluating it at specific points involves substituting the x and y values of those points into the derivative expression. In this case, since we are interested in points where y=1, we will first find the corresponding x values from the original equation and then substitute these into the derivative to find the slope at those points.
Recommended video:

Critical Points

 5:14m
5:14mWatch next
Master Finding The Implicit Derivative with a bite sized video explanation from Nick
Start learningRelated Videos
Related Practice


