Table of contents
- 0. Functions7h 52m
- Introduction to Functions16m
- Piecewise Functions10m
- Properties of Functions9m
- Common Functions1h 8m
- Transformations5m
- Combining Functions27m
- Exponent rules32m
- Exponential Functions28m
- Logarithmic Functions24m
- Properties of Logarithms34m
- Exponential & Logarithmic Equations35m
- Introduction to Trigonometric Functions38m
- Graphs of Trigonometric Functions44m
- Trigonometric Identities47m
- Inverse Trigonometric Functions48m
- 1. Limits and Continuity2h 2m
- 2. Intro to Derivatives1h 33m
- 3. Techniques of Differentiation3h 18m
- 4. Applications of Derivatives2h 38m
- 5. Graphical Applications of Derivatives6h 2m
- 6. Derivatives of Inverse, Exponential, & Logarithmic Functions2h 37m
- 7. Antiderivatives & Indefinite Integrals1h 26m
- 8. Definite Integrals3h 25m
5. Graphical Applications of Derivatives
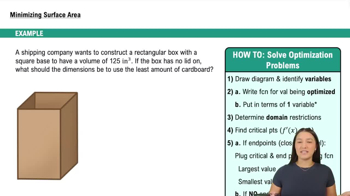
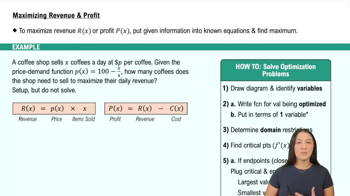
Applied Optimization
Problem 4.5.36
Textbook Question
Covering a marble Imagine a flat-bottomed cylindrical pot with a circular cross section of radius 4. A marble with radius 0 < r < 4 is placed in the bottom of the pot. What is the radius of the marble that requires the most water to cover it completely?
 Verified step by step guidance
Verified step by step guidance1
Understand that the problem involves finding the radius of a marble that maximizes the volume of water needed to cover it in a cylindrical pot.
Recognize that the volume of water required to cover the marble is related to the height of the water above the marble, which depends on the radius of the marble and the geometry of the pot.
Set up the relationship between the radius of the marble (r) and the height of the water (h) in the pot, using the formula for the volume of a cylinder: V = πR²h, where R is the radius of the pot.
Express the height of the water in terms of the radius of the marble, considering that the water will fill the space above the marble to the top of the pot.
Differentiate the volume function with respect to the radius of the marble and find the critical points to determine the radius that maximizes the volume of water needed.
Was this helpful?

 1:13m
1:13mWatch next
Master Intro to Applied Optimization: Maximizing Area with a bite sized video explanation from Callie
Start learningRelated Videos
Related Practice