Table of contents
- 0. Functions7h 52m
- Introduction to Functions16m
- Piecewise Functions10m
- Properties of Functions9m
- Common Functions1h 8m
- Transformations5m
- Combining Functions27m
- Exponent rules32m
- Exponential Functions28m
- Logarithmic Functions24m
- Properties of Logarithms34m
- Exponential & Logarithmic Equations35m
- Introduction to Trigonometric Functions38m
- Graphs of Trigonometric Functions44m
- Trigonometric Identities47m
- Inverse Trigonometric Functions48m
- 1. Limits and Continuity2h 2m
- 2. Intro to Derivatives1h 33m
- 3. Techniques of Differentiation3h 18m
- 4. Applications of Derivatives2h 38m
- 5. Graphical Applications of Derivatives6h 2m
- 6. Derivatives of Inverse, Exponential, & Logarithmic Functions2h 37m
- 7. Antiderivatives & Indefinite Integrals1h 26m
- 8. Definite Integrals4h 44m
- 9. Graphical Applications of Integrals2h 27m
- 10. Physics Applications of Integrals 2h 22m
2. Intro to Derivatives
Tangent Lines and Derivatives
Problem 3.1.14
Textbook Question
A projectile is fired vertically upward into the air; its position (in feet) above the ground after t seconds is given by the function s (t). For the following functions, use limits to determine the instantaneous velocity of the projectile at t = a seconds for the given value of a.
s(t) = -16t2 + 128t + 192; a = 2
 Verified step by step guidance
Verified step by step guidance1
Step 1: Understand that the instantaneous velocity of the projectile at time t = a is given by the derivative of the position function s(t) evaluated at t = a. This is because the derivative of a position function with respect to time gives the velocity function.
Step 2: The position function is s(t) = -16t^2 + 128t + 192. To find the instantaneous velocity, we need to find the derivative of s(t) with respect to t, which is denoted as s'(t).
Step 3: Differentiate the function s(t) = -16t^2 + 128t + 192. Use the power rule for differentiation: if f(t) = at^n, then f'(t) = n*at^(n-1). Apply this rule to each term in s(t).
Step 4: After differentiating, you will obtain s'(t) = -32t + 128. This is the velocity function, which gives the velocity of the projectile at any time t.
Step 5: Evaluate the velocity function s'(t) at t = a, where a = 2. Substitute t = 2 into s'(t) to find the instantaneous velocity at that specific time.
 Verified video answer for a similar problem:
Verified video answer for a similar problem:This video solution was recommended by our tutors as helpful for the problem above
Video duration:
4mPlay a video:
Was this helpful?
Key Concepts
Here are the essential concepts you must grasp in order to answer the question correctly.
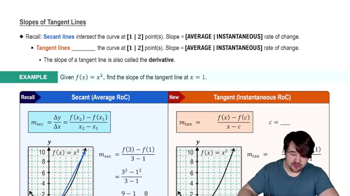
Limits
Limits are fundamental in calculus, representing the value that a function approaches as the input approaches a certain point. In the context of instantaneous velocity, limits help us find the derivative of a function at a specific point, which is the slope of the tangent line to the curve at that point. This concept is crucial for understanding how functions behave near particular values.
Recommended video:

One-Sided Limits
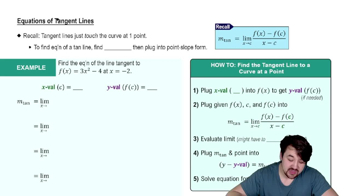
Derivatives
The derivative of a function measures how the function's output changes as its input changes, essentially providing the rate of change at any given point. For the position function s(t) of the projectile, the derivative s'(t) gives the instantaneous velocity. This concept is key to solving problems involving motion, as it allows us to determine how fast an object is moving at any moment.
Recommended video:

Derivatives
Projectile Motion
Projectile motion describes the motion of an object that is launched into the air and is subject to gravitational acceleration. The position function s(t) = -16t² + 128t + 192 models the height of the projectile over time, where the coefficients represent the effects of gravity and initial velocity. Understanding this concept is essential for analyzing the behavior of the projectile and applying calculus to find its instantaneous velocity.
Recommended video:

Derivatives Applied To Acceleration Example 2

 5:13m
5:13mWatch next
Master Slopes of Tangent Lines with a bite sized video explanation from Nick
Start learningRelated Videos
Related Practice