Table of contents
- 0. Functions7h 52m
- Introduction to Functions16m
- Piecewise Functions10m
- Properties of Functions9m
- Common Functions1h 8m
- Transformations5m
- Combining Functions27m
- Exponent rules32m
- Exponential Functions28m
- Logarithmic Functions24m
- Properties of Logarithms34m
- Exponential & Logarithmic Equations35m
- Introduction to Trigonometric Functions38m
- Graphs of Trigonometric Functions44m
- Trigonometric Identities47m
- Inverse Trigonometric Functions48m
- 1. Limits and Continuity2h 2m
- 2. Intro to Derivatives1h 33m
- 3. Techniques of Differentiation3h 18m
- 4. Applications of Derivatives2h 38m
- 5. Graphical Applications of Derivatives6h 2m
- 6. Derivatives of Inverse, Exponential, & Logarithmic Functions2h 37m
- 7. Antiderivatives & Indefinite Integrals1h 26m
- 8. Definite Integrals4h 44m
- 9. Graphical Applications of Integrals2h 27m
- 10. Physics Applications of Integrals 2h 22m
0. Functions
Introduction to Functions
Problem 10
Textbook Question
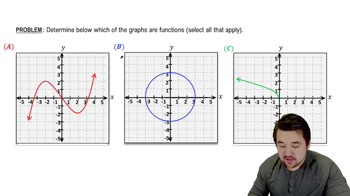
Evaluating functions from graphs Assume ƒ is an odd function and that both ƒ and g are one-to-one. Use the (incomplete) graph of ƒ and g the graph of to find the following function values. <IMAGE>
ƒ(g(4))
 Verified step by step guidance
Verified step by step guidance1
Step 1: Understand the properties of the functions involved. Since \( f \) is an odd function, it satisfies the property \( f(-x) = -f(x) \) for all \( x \). Both \( f \) and \( g \) are one-to-one functions, meaning they have unique outputs for each input and are invertible.
Step 2: Determine \( g(4) \) using the graph of \( g \). Locate the point on the graph where the input is 4 and find the corresponding output value, which is \( g(4) \).
Step 3: Use the value of \( g(4) \) found in Step 2 as the input for the function \( f \). This means you need to evaluate \( f(g(4)) \) by finding the output of \( f \) when the input is \( g(4) \).
Step 4: Use the graph of \( f \) to find \( f(g(4)) \). Locate the point on the graph of \( f \) where the input is \( g(4) \) and determine the corresponding output value.
Step 5: Verify the result by considering the properties of odd functions and one-to-one functions. Ensure that the value found for \( f(g(4)) \) is consistent with the properties of \( f \) being odd and both functions being one-to-one.
 Verified video answer for a similar problem:
Verified video answer for a similar problem:This video solution was recommended by our tutors as helpful for the problem above
Video duration:
2mPlay a video:
Was this helpful?
Key Concepts
Here are the essential concepts you must grasp in order to answer the question correctly.
Odd Functions
An odd function is defined by the property that f(-x) = -f(x) for all x in its domain. This symmetry about the origin implies that the graph of an odd function will reflect across both axes. Understanding this property is crucial when evaluating function compositions, as it can influence the output values based on the input.
Recommended video:

Properties of Functions
One-to-One Functions
A one-to-one function, or injective function, is one where each output is produced by exactly one input. This means that if f(a) = f(b), then a must equal b. This property is essential for determining the uniqueness of function values and is particularly important when composing functions, as it ensures that the inverse can be applied correctly.
Recommended video:

One-Sided Limits
Function Composition
Function composition involves applying one function to the result of another, denoted as f(g(x)). To evaluate f(g(4)), one must first find g(4) and then use that result as the input for f. Understanding how to properly execute this process is vital for solving problems that require evaluating nested functions.
Recommended video:

Evaluate Composite Functions - Special Cases

 1:36m
1:36mWatch next
Master Introduction to Calculus Channel with a bite sized video explanation from Callie
Start learning