Table of contents
- 0. Functions7h 52m
- Introduction to Functions16m
- Piecewise Functions10m
- Properties of Functions9m
- Common Functions1h 8m
- Transformations5m
- Combining Functions27m
- Exponent rules32m
- Exponential Functions28m
- Logarithmic Functions24m
- Properties of Logarithms34m
- Exponential & Logarithmic Equations35m
- Introduction to Trigonometric Functions38m
- Graphs of Trigonometric Functions44m
- Trigonometric Identities47m
- Inverse Trigonometric Functions48m
- 1. Limits and Continuity2h 2m
- 2. Intro to Derivatives1h 33m
- 3. Techniques of Differentiation3h 18m
- 4. Applications of Derivatives2h 38m
- 5. Graphical Applications of Derivatives6h 2m
- 6. Derivatives of Inverse, Exponential, & Logarithmic Functions2h 37m
- 7. Antiderivatives & Indefinite Integrals1h 26m
0. Functions
Introduction to Functions
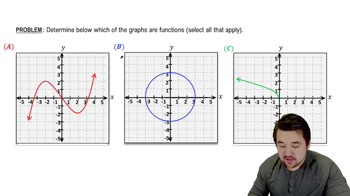
Problem 75c
Textbook Question
A GPS device tracks the elevation E (in feet) of a hiker walking in the mountains. The elevation t hours after beginning the hike is given in the figure. <IMAGE>
Notice that the curve in the figure is horizontal for an interval of time near t=5.5 hr. Give a plausible explanation for the horizontal line segment.
 Verified step by step guidance
Verified step by step guidance1
Identify that a horizontal line segment on a graph of elevation versus time indicates that the elevation is constant over that interval.
Understand that a constant elevation means there is no change in height, implying the hiker is neither ascending nor descending.
Consider possible real-world scenarios: the hiker could be taking a break, resting, or walking on a flat section of the trail.
Recognize that during this time, the derivative of the elevation with respect to time, which represents the rate of change of elevation, is zero.
Conclude that the horizontal segment suggests a period of no elevation change, possibly due to a rest or a flat terrain, which is common in hiking scenarios.
Recommended similar problem, with video answer:
 Verified Solution
Verified SolutionThis video solution was recommended by our tutors as helpful for the problem above
Video duration:
2mPlay a video:
Was this helpful?

 1:36m
1:36mWatch next
Master Introduction to Calculus Channel with a bite sized video explanation from Callie
Start learning