Table of contents
- 0. Functions7h 52m
- Introduction to Functions16m
- Piecewise Functions10m
- Properties of Functions9m
- Common Functions1h 8m
- Transformations5m
- Combining Functions27m
- Exponent rules32m
- Exponential Functions28m
- Logarithmic Functions24m
- Properties of Logarithms34m
- Exponential & Logarithmic Equations35m
- Introduction to Trigonometric Functions38m
- Graphs of Trigonometric Functions44m
- Trigonometric Identities47m
- Inverse Trigonometric Functions48m
- 1. Limits and Continuity2h 2m
- 2. Intro to Derivatives1h 33m
- 3. Techniques of Differentiation3h 18m
- 4. Applications of Derivatives2h 38m
- 5. Graphical Applications of Derivatives6h 2m
- 6. Derivatives of Inverse, Exponential, & Logarithmic Functions2h 37m
- 7. Antiderivatives & Indefinite Integrals1h 26m
- 8. Definite Integrals4h 44m
- 9. Graphical Applications of Integrals2h 27m
- 10. Physics Applications of Integrals 2h 22m
3. Techniques of Differentiation
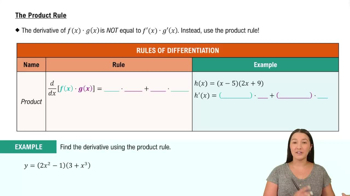
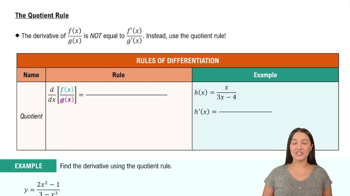
Product and Quotient Rules
Problem 66e
Textbook Question
Population growth Consider the following population functions.
e.Use a graphing utility to graph the population and its growth rate.
p(t) = 600 (t²+3/t²+9)
 Verified step by step guidance
Verified step by step guidance1
Step 1: Identify the function for the population, which is given as \( p(t) = 600 \left( \frac{t^2 + 3}{t^2 + 9} \right) \). This function represents the population at time \( t \).
Step 2: To find the growth rate of the population, we need to compute the derivative of \( p(t) \) with respect to \( t \). This involves using the quotient rule for derivatives, which is \( \frac{d}{dt} \left( \frac{u}{v} \right) = \frac{u'v - uv'}{v^2} \), where \( u = t^2 + 3 \) and \( v = t^2 + 9 \).
Step 3: Calculate the derivatives \( u' \) and \( v' \). For \( u = t^2 + 3 \), \( u' = 2t \). For \( v = t^2 + 9 \), \( v' = 2t \).
Step 4: Substitute \( u, v, u', \) and \( v' \) into the quotient rule formula to find \( p'(t) \), the growth rate of the population. This gives \( p'(t) = 600 \left( \frac{(2t)(t^2 + 9) - (t^2 + 3)(2t)}{(t^2 + 9)^2} \right) \).
Step 5: Simplify the expression for \( p'(t) \) to obtain a more manageable form for graphing. This involves expanding and combining like terms in the numerator, and then simplifying the entire expression.
 Verified video answer for a similar problem:
Verified video answer for a similar problem:This video solution was recommended by our tutors as helpful for the problem above
Video duration:
3mPlay a video:
Was this helpful?
Key Concepts
Here are the essential concepts you must grasp in order to answer the question correctly.
Population Functions
Population functions model the growth of a population over time, often represented as p(t), where t is time. In this case, the function p(t) = 600(t² + 3)/(t² + 9) describes how the population changes based on the variable t. Understanding the structure of this function is crucial for analyzing its behavior and growth patterns.
Recommended video:

Properties of Functions
Graphing Utilities
Graphing utilities are software tools or calculators that allow users to visualize mathematical functions. They can plot graphs of equations, helping to illustrate the relationship between variables. Using a graphing utility to plot the population function and its growth rate provides a visual representation that aids in understanding trends and behaviors in population dynamics.
Recommended video:

Graphing The Derivative
Growth Rate
The growth rate of a population refers to the change in population size over time, often expressed as a derivative of the population function. For the given function, calculating the derivative p'(t) will yield the growth rate, indicating how quickly the population is increasing or decreasing at any point in time. Analyzing the growth rate is essential for understanding the dynamics of population change.
Recommended video:
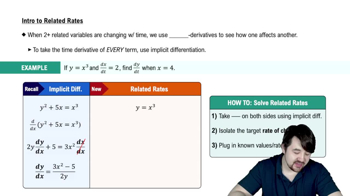
Intro To Related Rates
Related Videos
Related Practice