Use the definition of the derivative to evaluate the following limits.
${\lim }_{x\to 2}{\frac{5^x-25}{x-2}}_{}$

Key Concepts

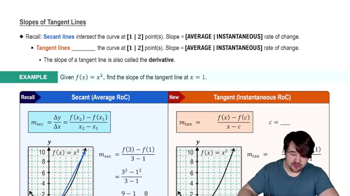
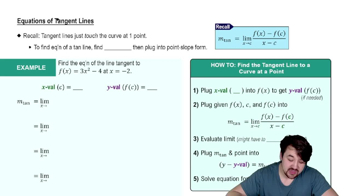
Definition of the Derivative

The derivative of a function at a point is defined as the limit of the average rate of change of the function as the interval approaches zero. Mathematically, it is expressed as f'(a) = lim (h→0) [f(a+h) - f(a)] / h. This concept is fundamental for evaluating limits that represent the slope of the tangent line to the curve at a specific point.

Limit Evaluation

Limits are used to analyze the behavior of functions as they approach a certain point. In this context, we are interested in the limit as x approaches 2 for the expression (5^x - 25) / (x - 2). Evaluating this limit often involves techniques such as direct substitution, factoring, or applying L'Hôpital's Rule when the limit results in an indeterminate form.

Exponential Functions

Exponential functions, such as 5^x, are functions where a constant base is raised to a variable exponent. They exhibit unique properties, including rapid growth and specific limits as x approaches certain values. Understanding the behavior of exponential functions is crucial for evaluating limits involving expressions like 5^x, especially when determining continuity and differentiability at specific points.