Table of contents
- 0. Functions7h 52m
- Introduction to Functions16m
- Piecewise Functions10m
- Properties of Functions9m
- Common Functions1h 8m
- Transformations5m
- Combining Functions27m
- Exponent rules32m
- Exponential Functions28m
- Logarithmic Functions24m
- Properties of Logarithms34m
- Exponential & Logarithmic Equations35m
- Introduction to Trigonometric Functions38m
- Graphs of Trigonometric Functions44m
- Trigonometric Identities47m
- Inverse Trigonometric Functions48m
- 1. Limits and Continuity2h 2m
- 2. Intro to Derivatives1h 33m
- 3. Techniques of Differentiation3h 18m
- 4. Applications of Derivatives2h 38m
- 5. Graphical Applications of Derivatives6h 2m
- 6. Derivatives of Inverse, Exponential, & Logarithmic Functions2h 37m
- 7. Antiderivatives & Indefinite Integrals1h 26m
3. Techniques of Differentiation
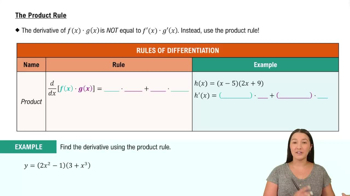
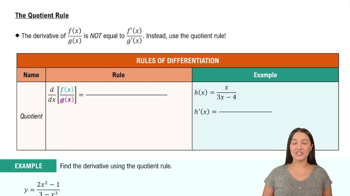
Product and Quotient Rules
Problem 3.4.46
Textbook Question
Derivatives Find and simplify the derivative of the following functions.
h(x) = (x−1)(2x²-1) / (x³-1)
 Verified step by step guidance
Verified step by step guidance1
Step 1: Identify the function as a quotient of two functions, where the numerator is \((x-1)(2x^2-1)\) and the denominator is \(x^3-1\). We will use the quotient rule for derivatives, which is given by \(\frac{d}{dx}\left(\frac{u}{v}\right) = \frac{v \cdot u' - u \cdot v'}{v^2}\), where \(u\) is the numerator and \(v\) is the denominator.
Step 2: Differentiate the numerator \(u = (x-1)(2x^2-1)\) using the product rule. The product rule states that \(\frac{d}{dx}(fg) = f'g + fg'\). Let \(f(x) = x-1\) and \(g(x) = 2x^2-1\). Find \(f'(x)\) and \(g'(x)\), then apply the product rule.
Step 3: Differentiate the denominator \(v = x^3-1\). The derivative of \(v\) is \(v' = 3x^2\).
Step 4: Substitute \(u\), \(u'\), \(v\), and \(v'\) into the quotient rule formula. Simplify the expression obtained from the quotient rule.
Step 5: Simplify the resulting expression further, if possible, by combining like terms and reducing any common factors in the numerator and the denominator.
Recommended similar problem, with video answer:
 Verified Solution
Verified SolutionThis video solution was recommended by our tutors as helpful for the problem above
Video duration:
7mPlay a video:
Was this helpful?
Related Videos
Related Practice