Table of contents
- 0. Functions7h 52m
- Introduction to Functions16m
- Piecewise Functions10m
- Properties of Functions9m
- Common Functions1h 8m
- Transformations5m
- Combining Functions27m
- Exponent rules32m
- Exponential Functions28m
- Logarithmic Functions24m
- Properties of Logarithms34m
- Exponential & Logarithmic Equations35m
- Introduction to Trigonometric Functions38m
- Graphs of Trigonometric Functions44m
- Trigonometric Identities47m
- Inverse Trigonometric Functions48m
- 1. Limits and Continuity2h 2m
- 2. Intro to Derivatives1h 33m
- 3. Techniques of Differentiation3h 18m
- 4. Applications of Derivatives2h 38m
- 5. Graphical Applications of Derivatives6h 2m
- 6. Derivatives of Inverse, Exponential, & Logarithmic Functions2h 37m
- 7. Antiderivatives & Indefinite Integrals1h 26m
- 8. Definite Integrals4h 44m
- 9. Graphical Applications of Integrals2h 27m
- 10. Physics Applications of Integrals 2h 22m
0. Functions
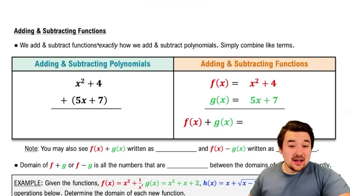
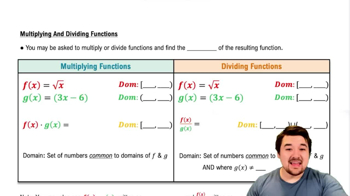
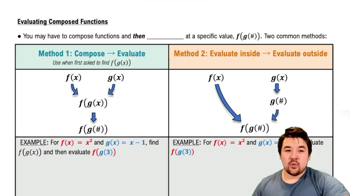
Combining Functions
Problem 46
Textbook Question
Graph ƒ₁ and ƒ₂ together. Then describe how applying the absolute value function in ƒ₂ affects the graph of ƒ₁.
ƒ₁(x) ƒ₂(x)
x² |x|²
 Verified step by step guidance
Verified step by step guidance1
Start by graphing the function ƒ₁(x) = x². This is a standard parabola that opens upwards with its vertex at the origin (0,0). The graph is symmetric about the y-axis.
Next, graph the function ƒ₂(x) = |x|². Note that |x| is the absolute value of x, which means it is always non-negative. However, since |x|² = x² for all real numbers x, the graph of ƒ₂(x) will be identical to the graph of ƒ₁(x).
Observe that applying the absolute value function to x before squaring it does not change the graph of the function. This is because squaring a number, whether positive or negative, results in a positive value, which is the same as squaring the absolute value of the number.
Consider the implications of the absolute value function. While |x| affects the graph by ensuring all x-values are non-negative, squaring negates this effect, resulting in the same graph as x².
Conclude that the application of the absolute value function in ƒ₂(x) does not affect the graph of ƒ₁(x) in this case, as both functions result in the same parabola y = x².
 Verified video answer for a similar problem:
Verified video answer for a similar problem:This video solution was recommended by our tutors as helpful for the problem above
Video duration:
6mPlay a video:
Was this helpful?
Key Concepts
Here are the essential concepts you must grasp in order to answer the question correctly.
Graphing Functions
Graphing functions involves plotting points on a coordinate plane to visualize the relationship between the input (x-values) and output (y-values) of a function. For the functions ƒ₁(x) = x² and ƒ₂(x) = |x|², understanding their shapes is crucial. ƒ₁ is a standard parabola opening upwards, while ƒ₂, due to the absolute value, also forms a parabola but reflects any negative x-values to positive, ensuring all outputs are non-negative.
Recommended video:

Graph of Sine and Cosine Function
Absolute Value Function
The absolute value function, denoted as |x|, transforms any negative input into its positive counterpart. This means that for the function ƒ₂(x) = |x|², the output is always non-negative, regardless of whether x is positive or negative. This property affects the graph by ensuring that all points lie above the x-axis, creating a symmetric shape about the y-axis.
Recommended video:

Average Value of a Function
Transformation of Graphs
Transformations of graphs refer to changes made to the original function that affect its position or shape. In this case, applying the absolute value to ƒ₁(x) = x² to create ƒ₂(x) = |x|² results in a graph that retains the parabolic shape of ƒ₁ but modifies it to reflect any negative portions of the graph upwards. This transformation emphasizes the non-negativity of the output values, altering the visual representation of the function.
Recommended video:
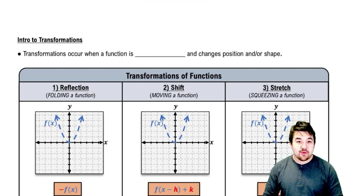
Intro to Transformations

 5:56m
5:56mWatch next
Master Adding & Subtracting Functions with a bite sized video explanation from Nick
Start learning