Table of contents
- 0. Functions7h 52m
- Introduction to Functions16m
- Piecewise Functions10m
- Properties of Functions9m
- Common Functions1h 8m
- Transformations5m
- Combining Functions27m
- Exponent rules32m
- Exponential Functions28m
- Logarithmic Functions24m
- Properties of Logarithms34m
- Exponential & Logarithmic Equations35m
- Introduction to Trigonometric Functions38m
- Graphs of Trigonometric Functions44m
- Trigonometric Identities47m
- Inverse Trigonometric Functions48m
- 1. Limits and Continuity2h 2m
- 2. Intro to Derivatives1h 33m
- 3. Techniques of Differentiation3h 18m
- 4. Applications of Derivatives2h 38m
- 5. Graphical Applications of Derivatives6h 2m
- 6. Derivatives of Inverse, Exponential, & Logarithmic Functions2h 37m
- 7. Antiderivatives & Indefinite Integrals1h 26m
- 8. Definite Integrals4h 44m
- 9. Graphical Applications of Integrals2h 27m
- 10. Physics Applications of Integrals 2h 22m
0. Functions
Introduction to Trigonometric Functions
Problem 70
Textbook Question
Observers at positions A and B 2 km apart simultaneously measure the angle of elevation of a weather balloon to be 40° and 70°, respectively. If the balloon is directly above a point on the line segment between A and B, find the height of the balloon.
 Verified step by step guidance
Verified step by step guidance1
First, visualize the problem by drawing a diagram. Place points A and B on a horizontal line, 2 km apart. Let point C be the point directly below the balloon on the line segment AB. The balloon is at point D, directly above C. You have two right triangles: ACD and BCD.
In triangle ACD, angle A is 40°. Use the tangent function, which relates the angle of elevation to the opposite side (height of the balloon, h) and the adjacent side (distance from A to C, x). The equation is: tan(40°) = h / x.
In triangle BCD, angle B is 70°. Similarly, use the tangent function: tan(70°) = h / (2 - x), where (2 - x) is the distance from B to C.
You now have two equations: tan(40°) = h / x and tan(70°) = h / (2 - x). Set these equations equal to each other to eliminate h: tan(40°) * x = tan(70°) * (2 - x).
Solve the equation for x, which represents the distance from A to C. Once you have x, substitute it back into either equation (e.g., h = x * tan(40°)) to find the height of the balloon, h.
 Verified video answer for a similar problem:
Verified video answer for a similar problem:This video solution was recommended by our tutors as helpful for the problem above
Video duration:
9mPlay a video:
Was this helpful?
Key Concepts
Here are the essential concepts you must grasp in order to answer the question correctly.
Trigonometric Functions
Trigonometric functions, such as sine, cosine, and tangent, relate the angles of a triangle to the lengths of its sides. In this problem, the tangent function is particularly useful, as it connects the angle of elevation to the height of the balloon and the horizontal distance from the observers to the point directly below the balloon.
Recommended video:
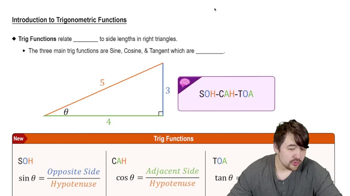
Introduction to Trigonometric Functions
Right Triangle Geometry
The scenario involves right triangles formed by the observers, the height of the balloon, and the horizontal distances from the observers to the point directly below the balloon. Understanding the properties of right triangles, including the Pythagorean theorem, is essential for calculating unknown lengths and heights based on the angles provided.
Recommended video:

Changing Geometries
Angle of Elevation
The angle of elevation is the angle formed between the horizontal line from an observer's eye to the object being observed and the line of sight to that object. In this problem, the angles of elevation from points A and B to the balloon allow us to set up equations that relate the height of the balloon to the distances from the observers, enabling us to solve for the height.
Recommended video:
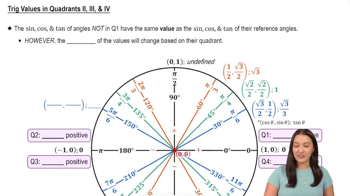
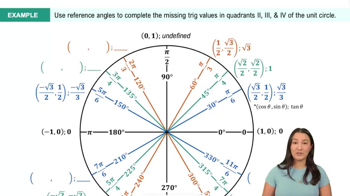
Trig Values in Quadrants II, III, & IV Example 2

 5:4m
5:4mWatch next
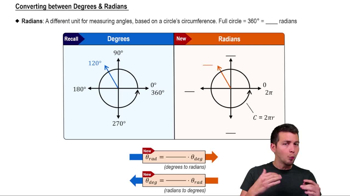
Master Converting between Degrees & Radians with a bite sized video explanation from Patrick Ford
Start learning