Table of contents
- 0. Functions7h 52m
- Introduction to Functions16m
- Piecewise Functions10m
- Properties of Functions9m
- Common Functions1h 8m
- Transformations5m
- Combining Functions27m
- Exponent rules32m
- Exponential Functions28m
- Logarithmic Functions24m
- Properties of Logarithms34m
- Exponential & Logarithmic Equations35m
- Introduction to Trigonometric Functions38m
- Graphs of Trigonometric Functions44m
- Trigonometric Identities47m
- Inverse Trigonometric Functions48m
- 1. Limits and Continuity2h 2m
- 2. Intro to Derivatives1h 33m
- 3. Techniques of Differentiation3h 18m
- 4. Applications of Derivatives2h 38m
- 5. Graphical Applications of Derivatives6h 2m
- 6. Derivatives of Inverse, Exponential, & Logarithmic Functions2h 37m
- 7. Antiderivatives & Indefinite Integrals1h 26m
- 8. Definite Integrals4h 44m
- 9. Graphical Applications of Integrals2h 27m
- 10. Physics Applications of Integrals 3h 16m
- 11. Integrals of Inverse, Exponential, & Logarithmic Functions2h 34m
1. Limits and Continuity
Continuity
Struggling with Calculus?
Join thousands of students who trust us to help them ace their exams!Watch the first videoMultiple Choice
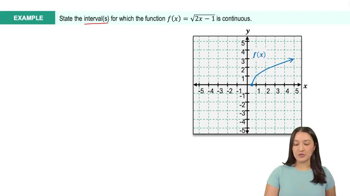
Determine the interval(s) for which the function is continuous.

A
B
(−∞,0),[0,∞)
C
(−∞,∞)
D
The function is not continuous anywhere.
 Verified step by step guidance
Verified step by step guidance1
Identify the piecewise function: f(x) = sqrt(9 - x^2) for -3 <= x < 0 and f(x) = 5 for x >= 0.
Determine the continuity of the first piece: sqrt(9 - x^2) is continuous for -3 <= x < 0 because it is a composition of continuous functions (square root and polynomial).
Determine the continuity of the second piece: The constant function f(x) = 5 is continuous for all x >= 0.
Check the continuity at the boundary x = 0: The left-hand limit as x approaches 0 from the left is sqrt(9 - 0^2) = 3, and the right-hand limit as x approaches 0 from the right is 5. Since these limits are not equal, f(x) is not continuous at x = 0.
Combine the intervals of continuity: The function is continuous on the intervals [-3, 0) and [0, ∞).
Related Videos
Related Practice