Table of contents
- 0. Functions7h 52m
- Introduction to Functions16m
- Piecewise Functions10m
- Properties of Functions9m
- Common Functions1h 8m
- Transformations5m
- Combining Functions27m
- Exponent rules32m
- Exponential Functions28m
- Logarithmic Functions24m
- Properties of Logarithms34m
- Exponential & Logarithmic Equations35m
- Introduction to Trigonometric Functions38m
- Graphs of Trigonometric Functions44m
- Trigonometric Identities47m
- Inverse Trigonometric Functions48m
- 1. Limits and Continuity2h 2m
- 2. Intro to Derivatives1h 33m
- 3. Techniques of Differentiation3h 18m
- 4. Applications of Derivatives2h 38m
- 5. Graphical Applications of Derivatives6h 2m
- 6. Derivatives of Inverse, Exponential, & Logarithmic Functions2h 37m
- 7. Antiderivatives & Indefinite Integrals1h 26m
- 8. Definite Integrals4h 44m
- 9. Graphical Applications of Integrals2h 27m
- 10. Physics Applications of Integrals 2h 22m
5. Graphical Applications of Derivatives
Finding Global Extrema
Problem 4.1.63
Textbook Question
Absolute maxima and minima Determine the location and value of the absolute extreme values of ƒ on the given interval, if they exist.
ƒ(x) = sec x on [-(π/4),π/4]
 Verified step by step guidance
Verified step by step guidance1
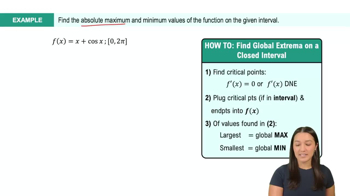
First, understand that absolute extrema refer to the highest and lowest values a function can take on a given interval. For the function ƒ(x) = sec(x), we need to find these values on the interval [-(π/4), π/4].
Calculate the derivative of ƒ(x) = sec(x). Recall that the derivative of sec(x) is sec(x)tan(x). This will help us find critical points where the derivative is zero or undefined.
Set the derivative sec(x)tan(x) equal to zero to find critical points. Since sec(x) is never zero, focus on tan(x) = 0. Solve for x to find critical points within the interval [-(π/4), π/4].
Evaluate the function ƒ(x) = sec(x) at the critical points found in the previous step, as well as at the endpoints of the interval, x = -(π/4) and x = π/4.
Compare the values of ƒ(x) at these points to determine the absolute maximum and minimum values on the interval. The largest value will be the absolute maximum, and the smallest will be the absolute minimum.
 Verified video answer for a similar problem:
Verified video answer for a similar problem:This video solution was recommended by our tutors as helpful for the problem above
Video duration:
6mPlay a video:
Was this helpful?
Key Concepts
Here are the essential concepts you must grasp in order to answer the question correctly.
Absolute Extrema
Absolute extrema refer to the highest and lowest values of a function over a specified interval. To find these values, one must evaluate the function at critical points, where the derivative is zero or undefined, as well as at the endpoints of the interval. The largest of these values is the absolute maximum, while the smallest is the absolute minimum.
Recommended video:
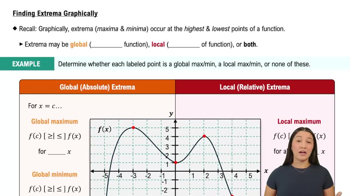
Finding Extrema Graphically
Critical Points
Critical points are values of the independent variable where the derivative of a function is either zero or undefined. These points are essential in determining the behavior of the function, as they can indicate potential locations for local maxima, minima, or points of inflection. In the context of finding absolute extrema, critical points within the interval must be evaluated alongside the endpoints.
Recommended video:

Critical Points
Secant Function
The secant function, denoted as sec(x), is the reciprocal of the cosine function, defined as sec(x) = 1/cos(x). It is important to consider the domain of the secant function, as it is undefined where cos(x) = 0. In the given interval [-(π/4), π/4], the secant function is continuous and differentiable, making it suitable for analysis in finding absolute extrema.
Recommended video:
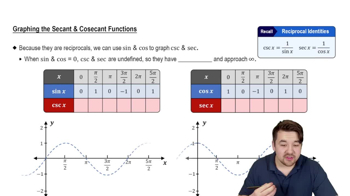
Graphs of Secant and Cosecant Functions
Related Videos
Related Practice