Table of contents
- 0. Functions7h 52m
- Introduction to Functions16m
- Piecewise Functions10m
- Properties of Functions9m
- Common Functions1h 8m
- Transformations5m
- Combining Functions27m
- Exponent rules32m
- Exponential Functions28m
- Logarithmic Functions24m
- Properties of Logarithms34m
- Exponential & Logarithmic Equations35m
- Introduction to Trigonometric Functions38m
- Graphs of Trigonometric Functions44m
- Trigonometric Identities47m
- Inverse Trigonometric Functions48m
- 1. Limits and Continuity2h 2m
- 2. Intro to Derivatives1h 33m
- 3. Techniques of Differentiation3h 18m
- 4. Applications of Derivatives2h 38m
- 5. Graphical Applications of Derivatives6h 2m
- 6. Derivatives of Inverse, Exponential, & Logarithmic Functions2h 37m
- 7. Antiderivatives & Indefinite Integrals1h 26m
- 8. Definite Integrals4h 44m
- 9. Graphical Applications of Integrals2h 27m
- 10. Physics Applications of Integrals 2h 22m
4. Applications of Derivatives
Implicit Differentiation
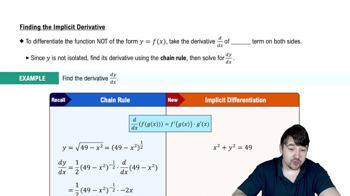
Problem 3.8.63d
Textbook Question
Witch of Agnesi Let y(x²+4)=8 (see figure). <IMAGE>
d. Verify that the results of parts (a) and (c) are consistent.
 Verified step by step guidance
Verified step by step guidance1
First, let's understand the equation given: y(x²+4)=8. This is a form of the Witch of Agnesi curve, which is a specific type of curve in calculus. The equation can be rearranged to solve for y: y = 8 / (x² + 4).
In part (a), you likely found a specific value or property of the curve, such as a point or a derivative. Let's assume you found the derivative of y with respect to x. To verify consistency, you need to compare this with the result from part (c).
In part (c), you might have found another property or value related to the curve, such as the slope at a specific point or the behavior of the curve as x approaches a certain value. Ensure you have this result ready for comparison.
To verify consistency, check if the derivative or property found in part (a) aligns with the behavior or value found in part (c). For example, if part (a) involved finding the slope at a point, and part (c) involved finding the tangent line at the same point, the slope should match the derivative value.
Finally, ensure that both parts (a) and (c) are based on the same assumptions and conditions. If they are consistent, the results should logically align, confirming the properties of the Witch of Agnesi curve as described by the equation y = 8 / (x² + 4).
 Verified video answer for a similar problem:
Verified video answer for a similar problem:This video solution was recommended by our tutors as helpful for the problem above
Video duration:
6mPlay a video:
Was this helpful?
Key Concepts
Here are the essential concepts you must grasp in order to answer the question correctly.
Witch of Agnesi
The Witch of Agnesi is a specific type of curve defined by the equation y = 8/(x² + 4). It is a bell-shaped curve that is symmetric about the y-axis and is often used in calculus to illustrate concepts of integration and area under a curve. Understanding its properties, such as its intercepts and asymptotic behavior, is crucial for analyzing related problems.
Verification of Results
Verification of results in calculus often involves checking that different methods or parts of a problem yield consistent outcomes. This can include comparing values obtained from derivatives, integrals, or graphical interpretations. Ensuring consistency helps validate the correctness of calculations and theorems applied in solving the problem.
Recommended video:

Integrals Resulting in Basic Trig Functions Example 1
Graphical Interpretation
Graphical interpretation involves analyzing the visual representation of mathematical functions and their properties. In the context of the Witch of Agnesi, understanding how the curve behaves graphically aids in comprehending its features, such as maxima, minima, and points of intersection. This visual approach can provide insights that complement algebraic methods.
Recommended video:
Determining Differentiability Graphically

 5:14m
5:14mWatch next
Master Finding The Implicit Derivative with a bite sized video explanation from Nick
Start learningRelated Videos
Related Practice