Solubilty Product Constant - Online Tutor, Practice Problems & Exam Prep

Solubility indicates the maximum amount of solute that can dissolve in a solvent, forming a homogeneous mixture called a solution. The solubility product constant (Ksp) relates to ionic solids; a larger Ksp signifies greater solubility. The reaction quotient (q) helps determine if a reaction is at equilibrium by comparing it to Ksp. If q equals Ksp, the system is at equilibrium. If q is greater than Ksp, the reaction shifts toward reactants, increasing precipitate. Conversely, if q is less than Ksp, the reaction shifts toward products, enhancing solubility and ion production.

Solubility Product Constant

So here we're going to say that solubility represents the maximum amount of solute that could successfully dissolve in a solvent. Remember, when a solute successfully dissolves within a solvent, we create a homogeneous mixture known as a solution. Now we're going to say associated with any solid, any ionic solid, is a K_sp value. This K_sp value is our solubility product constant. We're going to say here, the larger your solubility product constant, then the more soluble an ionic solid is in a solvent, so the more of it breaks up.

And then we're going to say here the smaller the solubility product constant, then the less soluble an ionic compound becomes within a solvent. Just remember, if we're looking at the solubility of any ionic compound, associated with it is a K_sp value. Now that we've gotten that out of the way, click on to the next video and see how we look at example 1 when we're asked to figure out what our solubility product constant is for a hypothetical ionic compound when given its molar solubility.

Solubility Product Constant

So here we're told we have a hypothetical compound with the formula M₃X and it has a molar solubility of 0.00562 molar. We're asked, what is the value of the solubility product constant for this hypothetical ionic compound? Now, with K_sp here, we're dealing with our ionic compound. It's going to break up into its ions. The metal which is M, breaks off.

This little 3 here came from the metal so its original charge was 3 plus. Remember, ions in solution are aqueous. Plus, there are 3 × negative ion. Here we have our equilibrium equation. With K_sp, we're talking about how it forms an equilibrium.

And with equilibriums, we'll set up an ICE chart. So we have initial change equilibrium. Remember, we ignore solids and liquids within an ICE chart. They're breaking the whole thing up in pure water, so initially, our ions are 0 and 0. But their products are being formed, so the change is going to be plus x for this.

This is a 3 here, so this is plus 3x. Bring down everything, so plus x and plus 3x. Now, K_sp is an equilibrium constant and like all other equilibrium constants, it equals products over reactants. But here when we're dealing with the saturated solution, we're going to say here that, we have our ionic compound as a solid so it's going to be ignored. Remember, we ignore solids and liquids.

So K_sp will just equal products. So in this case, it will equal M³⁺ and X³⁻. The 3 here makes this 3x but it also cubes it. It make it becomes the power. We're going to plug in what we know at equilibrium for each one of these ions.

So at equilibrium, M³⁺ is x and X⁻ is 3x. So plug it in. So x times 3x which is cubed. So that's going to be x times 3 cubed; 3 × 3 × 3 equals 27, and then this x cubed. Multiply together gives us 27x to the fourth power.

So that's what K_sp equals. Now realize here when they give us the solubility of the ionic compound, that solubility itself represents x. So all we're going to do now is plug that value in for x, so we'll write it over here. K_sp equals 27x to the fourth, so that's 27 times 0.00562 to the fourth. What you're going to do here is take that number to the fourth, then multiply by 27.

So 27 is going to multiply 9.9754 × 10⁻¹⁰. Multiply that by 27 so that comes out to 2.69 × 10⁻⁸. And like other equilibrium constants, K_sp has no units. So it's just that number which gives us option D. Just realize here when they're telling us the molar solubility, they're giving us what x equals for the entire ionic compound.

Using that is key to determining your K_sp. KSP. Now that you've seen example 1, take a look and see if you can do example 2. Here we're asked to figure out the highest molar solubility of each ionic compound given. Here we have to determine what the x variable backwards.

Moving in the opposite direction to help us to isolate x for each situation. And from that, you'll be able to see which one has the highest x value and therefore the highest molar solubility.

Solubility Product Constant

Here it states, which of the following compounds will have the highest molar solubility in pure water? Now to determine which one has the largest molar solubility, what we should do first is determine how many ions each ionic compound breaks up into. So first we have cobalt(II) hydroxide. That'll break up into when we work it out, we're gonna have 1 cobalt(II) ion plus 2 hydroxide ions. That's 3 ions total that we have here.

Next, we have strontium phosphate. That's gonna break up into 2. Let me work it out. 3 strontium ions plus 2 phosphate ions. So so far, we have 3 ions here, 5 ions here.

Next, we have lead(II) chloride. That breaks up into lead(II) ion plus 2 chloride ions. So that's 3 ions so far. Next, we have silver cyanide which breaks up into 1 silver ion plus 1 cyanide ion. So what do we have so far?

Two ions there. And then finally, we have lead(II) sulfate, which breaks up into lead(II) ion plus sulfate ion. All right. So let's look at the ones that have a number of ions in common. So we have first cobalt(II) hydroxide and lead(II) chloride.

They both have the same number of ions, so now we can look at their K_sp and determine which one is more, will have the higher molar solubility. Here, lead chloride has a higher K_sp value because it's 10 to the -5. So that means that it's more soluble than cobalt(II) hydroxide would be. Because it's more soluble, that means it's going to have a larger molar solubility. So automatically, we know that c is greater than a.

Next, we look at silver cyanide and lead sulfate because they both break up into 2 ions. Now comparing their Ksps here, we're going to say that this is to negative 8 whereas this one's to negative 17. So lead(II) sulfate is more soluble. Therefore, it's going to break up more easily into its ions, meaning you'll have a higher molar solubility. So this is out.

So what we have left are strontium phosphate, lead(II) chloride, and lead(II) sulfate. Here, we can't just simply look at them and look at their Ksps because they break up into a number of ions. In this case, we'll have to determine what our molar solubility or X is for each ionic compound. We'll start out first with strontium phosphate and see how it's done. Alright.

So we're gonna take this and work it out. So remember, we're dealing with an ionic solid breaking up. Since it's an ionic solid, this is out. These 2 are products so they're being made, so this is 0 and 0. There's a 3 here, so this is plus 3x.

There's a 2 here, so this is plus 2x. Plus 3x plus 2x. K_SP here will equal strontium ion, and it's cubed because of the 3 as its coefficient, times phosphate ion squared because also its coefficient. So we're gonna say here that comes out to 3x which is gonna be cubed, and 2x which is gonna be squared. So that's 27x³ times 4x², which comes out to 108x⁵.

So my K_SP here, which is 4.0 times 10 to the negative 28 equals 108x⁵. Alright. So at this point, divide both sides here by 108. So when we do that, we're gonna say now x⁵ equals 3.70 times 10 to the negative 30. Take the 5th root of both sides.

So x equals 1.30 times 10 to the negative 6 molar. This number here represents the molar solubility of the ionic compound itself. All we have to do now is do it also for lead(II) chloride and lead(II) sulfate. So we can get rid of this choice because we know that's not gonna be an answer. We're gonna bring this portion down over here.

Alright. So initial change equilibrium, x. So this is 0+0+x. There's a 2 here, so plus 2x, plus x, plus 2x. K_sp equals Pb²⁺ times Cl^- squared equals x times 2x², which comes out 4x³.

And this equals 1.60 times 10 to the negative 5. Divide both sides here by 4. So that's gonna give me now x³ equals 4.0 times 10 to the n

Solubility Product Constant Calculations 1

So here we're told that lanthanum(III) hydroxide has a K_sp of 2.0 times 10 to the negative 21. It says, how many grams of lanthanum(III) hydroxide are dissolved as hydroxide ions in 2.5 liters of a saturated solution of lanthanum(III) hydroxide. Alright. So we're dealing with the K_sp of this compound. If we're dealing with K_sp, that means we're dealing with the solid form of this compound and we look to see how it dissolves in water.

When it dissolves in water, it establishes an equilibrium. So we're going to have 1 lanthanum(III) ion. Ions when dissolved in water are aqueous plus 3 hydroxide ions. Here, we're going to set up an ICE chart when dealing with K_sp. So we have initial, change, equilibrium.

With an ICE chart, we ignore solids and liquids. So here, my solid will be ignored. Products are being formed over time, so this will be 0 + x. Because this is a 3, this is plus 3x, plus x, plus x plus 3x.

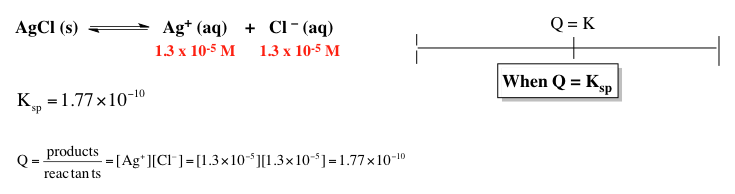
Now we're going to say K_sp, which is your solubility product constant equals products over reactants just like all of the equilibrium constants. But here for this equation, our reactant is a solid so we're going to ignore it. So K_sp here will just equal products. So it equals the concentration of lanthanum ion times hydroxide ion concentration because, again, this coefficient is 3.

Now this is going to be to the 3rd power. So that coefficient makes it 3x, but also makes it raised to that power. K_sp, again, is 2.0 times 10 to the negative 21. At equilibrium, La³⁺ is x, OH^- is 3x. So that's x times 27, and then that's x cubed.

27xx4=2.0×10-21

So here, we need to isolate x here. Divide both sides here by 27. When we do that here, that's going to give us 7.40741×10-23=x4. Now we need to just isolate x, not x to the 4th.

So I'm going to take the 4th root of both sides. For those of you who don't have a button for the 4th root, you may have to use the power function to calculate x to the 1/4. So that will give us x equals 2.934 times 10 to the negative 6 molar.

Now, regarding the original question, it's asking us to find grams. How many grams of lanthanum(III) hydroxide are dissolved as hydroxide ions?

We're looking for grams of hydroxide ions. The wording is a little tricky, but that's what we're looking for. If we need to find the grams of hydroxide ions, we need to first figure out its concentration. At equilibrium, hydroxide ion concentration equals 3x. So that's 3 times the answer we just got. So OH^- concentration here equals 8.80 times 10 to the negative 6 molar.

Remember that moles equals liters times molarity. We're told that we have 2.5 liters, so multiply that by the molarity we just isolated for hydroxide ions and that'll give us the moles of hydroxide ions, which comes out to 2.20 times 10 to the negative 5 moles of hydroxide ions.

All we have to do now is just change those moles into grams. So one mole of hydroxide ions consists of 1 oxygen and 1 hydrogen. So looking at your periodic table, the masses are 15.99994 grams and 1.00794 grams. Add them together gives us 17.00784 grams, roughly, of hydroxide ions.

Moles cancel out, and we'll get grams at the end which comes out to 3.74 times 10 to the minus 4 grams of hydroxide ion. So as you can see, there's a great deal of work that's required in terms of answering this question. Read the question very carefully. Here, we're not looking for grams of the entire ionic compound. We're looking for grams of lanthanum(III) hydroxide in the form of hydroxide ions.

So we're actually looking for the grams of hydroxide ions in this question. Anytime we find x, x gives us the solubility or concentration of our ionic compound as a whole. If we want the concentration of the particular ions, we have to look at the ICE chart. At equilibrium, this ion was just equal to x, so it'd be the same exact number. But for hydroxide ions, at equilibrium, it equals 3x.

So it'd be 3 times what we found for x to get the concentration of hydroxide ion. From there, we can change concentration to moles by using liters, and then change those moles into grams. Now that you've seen this example, move on ahead to example 2. See if you can approach this question. If you can't, don't worry.

Just come back and see how I tackle this example 2 question.

Solubility Product Constant Calculations 1

Here it says find the pH of a saturated solution of aluminum hydroxide. We're told that the Ksp of aluminum hydroxide is \(1.9 \times 10^{-10}\). Alright. We know that we're dealing with Ksp, so we're dealing with this ionic solid, and we're discussing how it breaks up into ions when thrown into solution. Breaks up into aluminum ion plus 3 hydroxide ions.

Hopefully, by following the example above, you're able to get this far in terms of this problem. The steps are very similar to what we saw above, except now we're being asked to find pH. Now here we're going to have initial change equilibrium. Again, remember, solids and liquids are ignored within our ice chart. We're going to say here, initially, this is 0 and this is 0.

This is plus x. Just like above, we have 3 OH_-s here, so this is 3x. So plus x plus 3x. Ksp equals products over reactants, but we ignore the reactants because it's a solid. So it equals \( \text{aluminum} \times \text{OH}^- \).

It's going to be cubed because of the 3 as a coefficient. Ksp is \(1.9 \times 10^{-10}\) x times \(3x\) which will be cubed. So just like above, we have \(27x^4\) again equals \(1.9 \times 10^{-10}\). Divide both sides here by 27, and we'll get what \(x^4\) equals. So \(x^4 = 7.03704 \times 10^{-12}\).

Again, like above, we're going to take the 4th power or you could do it to the 1/4th power to find x. X here equals \(1.629 \times 10^{-3} \text{molar}\). This represents the concentration of my entire ionic compound. But remember, we're looking for pH here. Now in our equation, we don't have H3O+ anywhere, but what we have instead is OH^-.

If I know OH_-'s concentration, I can find my pOH. And from there, I can find pH. So let's figure out what the concentration of OH_- is. At equilibrium, OH_- equals 3x. So OH_- equals 3x.

So that's 3 times \(1.629 \times 10^{-3}\). So that's going to come out to \(4.886 \times 10^{-3} \text{ molar}\). Now that we have the molarity, we can figure out what my pH is. So first, we're going to take the negative log of this number to find pOH, which is the negative log of OH^-. So that comes out to 2.31.

Now that we have pOH, we know pH because pH equals 14 minus pOH. So that comes out to 11.69 as my pH for this solution. If you're able to follow along with the example above, this question was similar up to a point. Once we found the concentration of OH^- in this example, we didn't need to figure out its grams or its moles. Instead, we'd use those concentrations that we found to figure out our pOH.

From pOH, transform it into pH, and we'd have our answer at the end. Following all the normal convergence we saw above, we see that our pH is equal to 11.69.

Solubility Product Constant Calculations

Here it says, calculate the molar solubility of iron(II) carbonate in a solution of 0.00167 molar sodium carbonate. Here we're told that the K_sp for iron(II) carbonate is 2.1 times 10^-11. We're dealing with iron(II) carbonate, and we're given its K_sp, which means that we know we're dealing with the solid form of it. We look at how it breaks up into ions.

Now realize, up to this point, we're normally seeing these ionic compounds breaking up in pure water. But in this case, it's breaking up in a solution comprised of sodium carbonate. Realize that sodium carbonate breaks up into sodium ions and carbonate ions. The carbonate ion is what's important because that carbonate is the same carbonate ion here. And we're going to say here because we're breaking up in a solution that already possesses carbonate ion, we're dealing with the common ion effect.

With the common ion effect, this is a solid so we're going to ignore it. There's no common ion of this. This is 0. With the common ion effect, because this carbonate which the solution is comprised of is the same as this ion here, the initial concentration will be 0.00167.

Then they are both products or they are both being made. So this is plus x, plus x, plus x and then 0.00167 plus x. Now with K_sp, remember we're concerned with the product because the reactant here, the ion, is ignored. K_sp equals Fe²⁺ times CO₃^2-.

K sp = 2.1 × 10 - 11 = x × 0.00167

So here K_sp is 2.1 times 10^-11 equals x times 0.00167. We're looking for the molar solubility of iron(II) carbonate. So that just means we're looking for x. So, divide both sides by 0.00167.

And when we do that, that's going to give us our x variable, which is our molar solubility for the entire ionic compound that comes out to 1.26 times 10^-8 molar. So remember, with the common ion effect, we're no longer breaking up our ionic compound in pure water. We're breaking up in a solution that possesses a common ion to one of the ions in our ionic compound. Taking this to heart, attempt to do practice question 1. If you get stuck, don't worry.

Just come back and take a look at how I approach that same exact practice question and how we get the answer that we're looking for.

What is the molar solubility of Fe(OH) ₃ (s) in a solution that is buffered at pH 3.50 at 25°C? The Ksp of Fe(OH)₃ is 6.3 x 10^–38 at 25°C.

• A. 1.99x10^-27 M
• B. 1.99x10^-34 M
• C. 6.64x10^-28 M
• D. 1.99x10^-6 M
• E. 1.25x10^-9 M

Arsenic trisulfide (As₂S₃) occurs naturally as the orange-yellow colored mineral orpiment. As₂S₃ is a highly insoluble substance with a Ksp value of 2.90×10⁻⁷². Calculate the solubility of As₂S₃ in g/100mL. (Molar mass of As₂S₃ = 246.04 g/mol)

• A. 2.12×10⁻¹⁷ g/100 mL
• B. 1.87×10⁻¹³ g/100 mL
• C. 6.25×10⁻¹⁵ g/100 mL
• D. 4.75×10⁻¹⁴ g/100 mL

Reaction Quotient & Precipitation

So here we're talking about the reaction quotient q. We're going to say here that the reaction quotient q is used to determine if our chemical reaction is at equilibrium when we compare it to k, or if we produce a precipitate solid. And that's when we compare it to ksp. Now remember, regular k is your equilibrium constant. Ksp is also an equilibrium constant.

But Ksp is an equilibrium constant for ionic solids. Now here we're going to say if the reaction quotient q is equal to the equilibrium constant k, then our reaction is at equilibrium. For example, here we have silver chloride as our solid. It breaks up into silver ion and chloride ion. Here, we're given the concentrations initially of silver and chloride as 1.3 × 10 - 5 molar.

Now, q is just like k. It equals products over reactants. Just like our equilibrium constant ksp, we ignore solids and liquids. So q equals just products, so it equals silver ion times chloride ion. Plugging those values in gives us our q value.

Here, our q is 1.77×10-10 just like Ksp is 1.77×10-10. They're equal to one another. So we can say because q and k equal each other here, we're at equilibrium. In terms of shifting of our chemical reaction, there would be no shifting of our chemical reaction because our chemical reaction is at equilibrium. Now later on when we look up new values for q, if q happens to be different from k, this will determine the direction our chemical reaction will shift in order to reattain equilibrium conditions.

This shifting can have an effect on the amount of reactant or product that's used or created. Now click on to the next video and see what happens when our q value is different from our k value or ksp value.

Reaction Quotient & Precipitation

So here we continue our discussion of the comparison of the reaction quotient q to any type of equilibrium constant including Ksp. Now in these next two scenarios, they will not be equal to one another. Because q and k will not be equal to one another, this will cause my chemical reaction to shift either in the forward direction or the reverse direction to reestablish equilibrium. Now in this first example here, we're given new concentrations for our ions. Here we have 0.250 molar for each one and when we plug them into the equilibrium expression for Q, we can see that the value of Q comes out to 6.25 × 10-2.

In this case, we're going to see that Q is bigger than our Ksp, which is still the same value. Remember, the only way this Ksp or any type of k would change is if there were a change in temperature. Our Ksp remains the same because the temperature is being held constant, but the concentration of my ions are changing which will have a direct impact on the value of q. Here, q is larger than Ksp. If q is larger than Ksp or k in general, then our reaction will shift.

So, in terms of our number line, q will always shift to wherever k is. Here, q will shift this way to get to k. And if it shifts that way to get to k, then our chemical reaction will also shift as a whole to get to equilibrium. Remember, wherever you shift, that direction will cause an increase. Here we're shifting towards the reactant side, so the reactant side would be increasing.

If the reactant side is increasing, that means our product side is decreasing. We're going to say, If q is larger than k, then our chemical reaction will shift to the reactant side. In this case, because we're shifting towards the reactant which happens to be a solid, that means that the solubility will increase. Now in this case, we have new concentrations yet again for the ions. Again, the temperature is not being affected, so our equilibrium constant Ksp remains the same.

In this case, we have these new concentrations which give us a new q value which is 5.06 × 10-24. We can now see that value is less than Ksp. So if q is smaller than KSP or k in general, so now q is no longer here. Instead, q will be over here. It's going to be smaller.

So we're going to have to shift this way now. We're going to shift that way in terms of the number line and in terms of the chemical reaction. Remember, wherever we're heading towards, that side is increasing. This side would increase. This side here would decrease.

Our chemical reaction will shift to the product side. That means that the solubility of my ionic compound is increasing because I'm making more ions, and the amount of precipitated solid will decrease. Just remember, q, our reaction quotient is just used as a way of determining if we are at equilibrium. When q equals Ksp, we're at equilibrium. We will say that our chemical reaction or actually our solution is a saturated solution with no real dominant movement to the reactant side or the product side.

We're at equilibrium. Everything is balanced, so we'd represent a saturated solution. Then if our q happens to be larger than our Ksp, that means we have an excess of ions, so that's why the reaction is shifting in the reverse direction towards the reactants to recreate some more precipitate. We'd say that this type of process happens within a supersaturated solution. And then finally, when q is less than Ksp, we can continue to break down our ionic solid to produce more ions.

When q is less than Ksp, we'd say that we are in an unsaturated solution. Now that we've explored the connection between our reaction quotient, q, and Ksp, we'll be more prepared when we take a look at questions dealing with more calculation-based questions on the formation or lack thereof of precipitates.

Reaction Quotient & Precipitation Calculations 1

Here it asks, will a precipitate form when 0.150 liters of 0.100 molar lead acetate and 0.100 liters of 0.20 molar sodium chloride are mixed. Here we're told that the K_sp value of lead (II) chloride is 1.2 × 10^-5.

We're given the K_sp of lead (II) chloride, so that's our ionic compound of interest. We're going to take that ionic compound and break it down. It's our solid and we show how it dissociates into its ions when it's thrown into our solution. That creates lead (II) ion, aqueous, plus 2 chloride ions. Here we have the initial change equilibrium. Realize at this point, we have this lead (II) chloride forming and what's happening here is we have a solution made up of lead (II) acetate and sodium chloride. They're breaking up into ions and those ions are combining together to help form this solid here.

All we're doing is showing its equilibrium equation. This lead (II) chloride exists in a solution made up of these four particular ions. It's not being formed in pure water. And if you look, those four ions from those compounds, we have lead (II) ion in it and we care about that because that's part of my equilibrium equation. And then we have chloride ion here which is also part of my equation.

Here we're dealing with the double common ion effect. These amounts that we're going to find for both the lead (II) ion and the chloride ion will be used to help us determine what Q will be. So we have to figure out what the concentration of this lead (II) ion is and the concentration of this chloride ion is. Remember, liters of a molarity means multiply. Remember that moles equal liters times molarity.

So we take 0.150 liters, multiply it by the 0.100 molar of lead (II) acetate. That's going to give me 0.0150 moles of lead (II) acetate. Realize here that we don't want the moles of lead (II) acetate; we just want the moles of lead (II) ion because that is one of our common ions. We're going to say here for every one mole of the entire compound, we have exactly 1 mole of lead (II) ion involved.

Now, we need to determine its concentration or its molarity. Realize here that we have 0.150 liters of one compound mixing with exactly 0.100 liters of another compound. Their combined volume forms the new overall volume of the solution. For the moles we just found, we're going to divide them by the total volume. That's the volume from the two mixtures, the two compounds.

Doing that will give me my new concentration for lead (II) ions which comes out to 0.060 M. Now we have to do the same thing and figure out the new concentration of chloride ions. So we have 0.100 liters of 0.20 M sodium chloride. Again, remember that liters times molarity will give me moles. So bring that down.

We don't want the moles of sodium chloride. We just want the moles of chloride ion itself. For every one mole of sodium chloride, we can see that there's exactly 1 chloride in the formula. That's 0.02 moles of chloride ions. Again, we want to find its new concentration, so we divide it by the total volume used.

So that's 0.150 liters plus 0.100 liters on the bottom. Together, all of that gives me a molarity of 0.080 M for my chloride ions. So take that concentration and plug it in.

So now we have initial concentrations for both. So this would be +x +2x, 0.060+x, 0.080 +2x. Because we have initial concentrations for both ions, this is going to help me figure out what my Q value will be. So we're going to say here: Q = Pb+2*Cl-2. So it's going to be 0.060 × (0.080)². When we plug that in, that gives me 3.84 × 10^-4 as my Q value.

Now remember, what we said in previous videos, is at this point when we find Q, we can compare it to our K_sp using a number line. That'll dictate which direction my chemical reaction will shift. Here's our number line here. We'll put K_sp in the middle. We're told that K_sp of lead (II) chloride is 1.2 × 10^-5.

We can see that Q is this number, 3.84 × 10^-4. Here, it's to the negative 4, so it's a larger value. So Q will be over here. All we say now at this point is because K_sp and Q are not equal to each other, and you are not at equilibrium, Q will shift in the direction needed to get to K_sp. So Q will shift this way to get to K_sp.

Remember, whichever direction you shift in to get to K is the same direction your reaction will shift to as well. Here, my chemical reaction would have to shift also this way. Wherever you're shifting will be increasing in amount. So my reactant here is increasing because we're shifting towards the reactants and this side here is decreasing.

We're saying here that my precipitate, which is my solid is increasing in amount. So we would say yes, a precipitate does form. Remember, when Q is greater than K_sp, a precipitate will begin to form because our solution represents a supersaturated solution. To get back to equilibrium, some of the extra dissolved solute has to recrystallize in the solution itself. Again, here because Q is greater than K_sp, our reaction moves in the reverse direction towards reactants, thereby creating more solid.

Now that you've seen this example, look to see if you can tackle example 2. If you get stuck or lost, don't worry. Just come back and take a look at how we approach example 2.

Reaction Quotient & Precipitation Calculations 1

So here the question states, what is the minimum pH at which iron(II) hydroxide will precipitate if the solution has a concentration of iron(II) ion equal to 0.0583 molar. Here we're told that the K_sp of iron(II) hydroxide is \(4.87 \times 10^{-17}\). This question here, although it's asking for precipitation, it's really piggybacking off of the idea of just the simple common ion effect of iron(II) ion. We're going to write the ionic compound. We know here it's going to form an equilibrium with its ions, so it's Fe²⁺ plus 2 hydroxide ions.

We're going to have initial change equilibrium. We ignore the solid. Here, this iron(II) hydroxide compound is not breaking up in pure water but in fact the solution already comprised of iron(II) ion. We have 0.0583 molar iron(II) ion. That there represents the initial concentration.

Here, we're going to say the initial concentration for hydroxide is 0 since we don't have that common ion at all present in our solution. This is going to be plus \(x\) plus \(2x\). Here, we're dealing with K_sp, not \(Q\). We dealt with \(Q\) in the previous question because we had initial amounts for both of my products initially.

Here because we only have one of them, it's just a simple common ion effect that also encompasses a question dealing with a precipitate. So \(K_{sp} = [\text{Fe}^{2+}][\text{OH}^-]^2 = 4.87 \times 10^{-17}\). With the concentration known to be 0.0583, \(2x\) will be squared, and multiplying it out will give 0.2332 times \(x^2\). Divide both sides by 0.2332, so \(x^2 = 2.08834 \times 10^{-16}\).

We want the square root of both sides now. So \(x\) here at this point equals \(1.445 \times 10^{-8}\) molar. Now they're asking us for the minimum pH. Realize here that, pH is just the negative log of \([H^+]\) concentration. What we have that's similar to that is \([OH^-]\).

What we're going to do here is we're actually going to figure out what the concentration of \([OH^-]\) is first. So at equilibrium, \([OH^-] = 2x\). Plug in the \(x\) that we just found. That's going to give me a concentration of \(2.89 \times 10^{-8}\) molar as the concentration of my hydroxide ion. If we know the concentration of hydroxide ion, then we know \(pOH\) by default because it's equal to the negative log of \([OH^-]\).

So that's negative log of \(2.89 \times 10^{-8}\). That's going to give me 7.54. That's my \(pOH\). I don't want \(pOH\), I want pH. \(pH = 14 - pOH\).

So it's \(14 - 7.54\) which comes out to 6.46. That there represents the minimum pH at which a precipitate of iron(II) hydroxide can become a precipitate. Now realize here again, we didn't use \(Q\) in this question because we didn't have the initial concentrations of both of my products. Because of that, this is just a common ion effect question to help us figure out the concentration in which we can get \([OH^-]\) and from that, we could figure out pH eventually.