Chemical Thermodynamics: Gibbs Free Energy - Online Tutor, Practice Problems & Exam Prep

Gibbs free energy (ΔG) is crucial for understanding spontaneous reactions, which occur naturally without external energy. For spontaneity, ΔG must be less than 0, the entropy of the universe (ΔS) greater than 0, and the equilibrium constant (K) greater than 1. In contrast, non-spontaneous reactions have ΔG > 0, ΔS < 0, and K < 1. At equilibrium, ΔG = 0, ΔS = 0, and K = 1. The relationship between ΔG and enthalpy (ΔH) is expressed as ΔG = ΔH - TΔS, where T is temperature in Kelvin.

Gibbs Free Energy

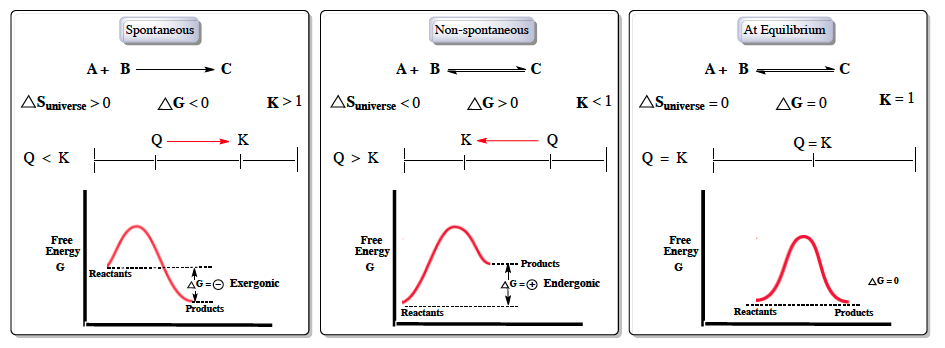
Hey everyone. So when we talk about Gibbs free energy, it's usually in reference to spontaneous reactions. Now, spontaneous reactions can occur without any outside energy being invested; they happen naturally. Non-spontaneous reactions, on the other hand, cannot occur naturally. Now, when we talk about spontaneous reactions, we're discussing spontaneous, non-spontaneous, and being at equilibrium. And with these ideas, we have the variables of Gibbs free energy, entropy of our universe, which is tied to the second law of thermodynamics, and then our equilibrium constant \( k \). So, if we take a look at spontaneous reactions, here we have our reactants A and B producing our product C. For this to be a spontaneous result we would say that the entropy of our universe would have to be greater than 0, following the second law of thermodynamics. Gibbs free energy \( \Delta G \) would have to be less than 0, and then our equilibrium constant \( K \) would have to be greater than 1. Typically when we're talking about a spontaneous reaction, that means that the reactants naturally flow in the forward direction to create products. We can attach this idea to the whole thing of the reaction quotient \( q \). To move in the forward direction, the reaction quotient \( q \) would have to be less than \( k \). Remember that \( q \) means to shift to get to equilibrium, to get to \( k \). Since \( q \) is less than \( k \), \( q \) would move in the forward direction to get to \( k \), which would mean that the reaction would also move in the forward direction. Now, if we're to talk about Gibbs free energy in terms of an energy diagram, realize that we have our reactants and then we're trying to get to our products. For a reaction to be favorable, we'd want our products to be lower in overall energy. So here we'd see that our reactants are starting up at a higher energy level and our products are ending at a lower level. Here to understand the value associated with this is just that \( \Delta G \) equals products minus reactants. So this is the equation you can link to each one of these energy diagrams. As a result of products being lower in energy, that means our \( \Delta G \) value overall is negative or what we call exergonic. For a non-spontaneous reaction, it's basically the opposite process. So signs get flipped, here we'd say that our entropy of the universe would be less than 0, Gibbs free energy will be greater than 0, \( k \) would be less than 1. In a non-spontaneous reaction, that means that it's not favorable in the forward direction, but it would be favorable in the reverse direction. So here, to favor the reverse direction that happens when \( q \), our reaction quotient, is greater than \( k \). So here since \( q \) is greater than \( k \), \( q \) always shifts to wherever \( k \) is, so it's going to shift in the forward reverse direction to obtain equilibrium. Here when we're talking about the energy diagram again, this time products would be higher in energy which is not something we want. The whole purpose of most chemical reactions is to create a product that is lower in energy. Following products minus reactants, \( \Delta G \) would be positive here, meaning that it is endergonic. And then finally when we're neither spontaneous nor non-spontaneous we are at equilibrium. So here \( \Delta S \) of the universe would be equal to 0. We'd say that Gibbs free energy is equal to 0. \( K \) is equal to 1. Since we're at equilibrium, our reaction quotient \( q \) is equal to our equilibrium constant \( k \) so there's no shifting. And then looking at our energy diagrams, reactant products would end at the same energy level, so overall our \( \Delta G \) value would be equal to 0. Now beside these conceptual ideas, we can also talk about the formulas associated with our Gibbs free energy. Gibbs free energy can also be calculated through the use of various equations. So here we have Gibbs free energy under non-standard conditions equals change in enthalpy minus \( T \times \Delta S \). Here, realize that there's no circle here. That means that when there's no circle here, that's non-standard conditions. If I were to talk about standard conditions, I'd put a little circle there for each of them. Standard conditions would mean that our pressure is 1 atmosphere. Standard conditions would also mean that our molarity is 1 molar. Standard conditions here could also mean that our temperature is 25 degrees Celsius. Now, with our Gibbs free energy under non-standard conditions, we can have it equal to Gibbs free energy under standard conditions plus \( RT \times \ln(q) \). \( R \) here represents our gas constant and since we're talking about energy, it would be in the form of 8.314 joules over moles times \( k \). \( T \) here will be our temperature in Kelvin and \( q \) again is our reaction quotient, which is equal to products over reactants. And then finally, we can associate our Gibbs free energy under standard conditions with our equilibrium constant \( k \) by the use of this equation: negative \( RT \ln(k) \). So, just remember that a spontaneous reaction can occur naturally without the use of outside energy. Associated with this idea of spontaneity, we have variables of \( \Delta S \) universe, our equilibrium constant \( k \), as well as, of course, our Gibbs free energy, which is \( \Delta G \).

Gibbs Free Energy

Recall that a spontaneous reaction is one that happens naturally without the need of outside energy. Whereas, a non-spontaneous reaction is one that doesn't normally occur unless some outside force is in play. So, if we take a look here, we have to figure out which of the following is an example of a non-spontaneous process. Alright. First, we have ice melting at room temperature. Now we know that as long as the temperature is above 0 degrees Celsius, which room temperature is, ice will naturally melt. Because this happens in real-world scenarios, it is a spontaneous process.

Next, sodium metal reacting violently with water. This one is probably not as well known. Just remember here that when it comes to group 1a and 2a metals, because of the low number of valence electrons they have, they will violently react with water in order to lose an electron to become more like a noble gas. Here, this is true. Because it happens naturally in the real world, it's a spontaneous process.

Here, rusting of iron at room temperature. So old cars, when it rains, they can rust. This is a natural process that happens every day and because of that, it's a spontaneous process. A ball rolling downhill. A ball can naturally roll down a hill using its momentum as well as gravity to push itself down that hill. We can see that this happens just by imagining it. You could take a ball and throw it down a hill. You'll see it roll down—a spontaneous process.

Even if you weren't able to determine if the initial four were spontaneous or not, you should know that e is a non-spontaneous process. Here, it says water freezing at room temperature. Room temperature is much too warm for water to freeze. Water has to be at 0 degrees Celsius or lower in order to begin to freeze. We should know that this makes no sense. It's not a natural occurrence. It could only happen if we invested some outside energy. For example, we have a refrigerator where we put an ice tray in. The refrigerator is in a room at room temperature, but inside the refrigerator, it's much lower than that. Therefore, water could freeze. The last option here, we're going to say, does not naturally occur. Water will not freeze at room temperature under natural conditions. Therefore, it is the process that is non-spontaneous.

Also remember, as we set up above, spontaneous reactions move in the forward direction. They never move in the reverse direction because if going to move forward, it is nonreversible or irreversible. Non-spontaneous processes are not spontaneous in the forward direction, so they tend to move in the reverse direction to become spontaneous. So, non-spontaneous processes are reversible.

Gibbs Free Energy Calculations 1

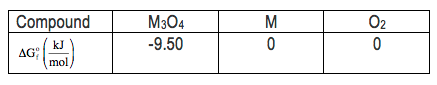
Here it says, consider the decomposition of a metal oxide to its elements where "M" represents a generic metal. Here we have M₂O₄ (solid) decomposes to give us 3 moles of M (solid) plus 2 moles of oxygen gas. We're also given the Gibbs free energies of formation, so ΔG_f here, of these four separate compounds. Now, if we look at part 1, it says, what is the standard change in Gibbs energy for the reaction as written in the forward direction? So, they're asking us to find ΔG° of the reaction. "Standard" here means that we have this little circle here. Remember, whether you're looking for ΔG of reaction, ΔS of reaction, or ΔH of reaction, all of them equals products minus reactants. So, products minus reactants. Realize here that the Gibbs free energy of formation for M (solid) and oxygen gas are both 0. That's because when an element is in its natural or standard state, its Gibbs free energy will be 0, just like its enthalpy would be 0. Here, we're going to say we have 3 moles of M (solid) which really isn't going to change anything, and the 3 comes from here, times 0 + 2 moles, O₂. Each one is also 0. Minus, we have 1 mole of M₂O₄. Its Gibbs free energy of formation is this negative 950 kilojoules per mole. So, all of this here is 0, so we could ignore that, and we have a negative of a negative which comes out to a positive. So that's a positive 950 kilojoules because here the moles cancel out.

Now for part 2, it says, what is the equilibrium constant of this reaction as written in the forward direction at 298 Kelvin? So from the previous page, we've seen that ΔG can be connected to our equilibrium constant K by this formula: ΔG = -RT ln K. But here's the thing. We don't want to find ΔG. We already found that in part 1. Now in part 2, we're looking for what our equilibrium constant K is. Finding K means we rearrange the equation now to become K = e, which is the inverse of the natural log, to the negative ΔG° divided by RT. So this is the equation we're going to use now. So it's e to the negative. R here is in joules, so we're going to have to convert the ΔG we found earlier also into joules. So remember, 1 kilojoule is equal to 1000 joules. So that's 9,500 joules. So 9,500 joules per mole here divided by 8.314 joules over moles times K, which is the value of R constant. And the temperature here is 298 Kelvin. Here, moles will cancel out, joules will cancel out, and kelvins will cancel out, meaning that our equilibrium constant has no units. This becomes e to the negative 3.8344. And when you plug that into your calculator, that's going to give you 2.16 times 10 to the negative 2 for my value, for my equilibrium constant K.

So now that we found that, we finally figure out the last portion, part 3. What is the equilibrium pressure of O₂ over M solid at 298 Kelvin? Now we're being asked to figure out the equilibrium pressure of this gas. Now here, because they're using the terminology of "equilibrium pressure" or "equilibrium concentration," that means you're going to have to use the equilibrium constant we just found. K equals products over reactants. Remember, your equilibrium constant ignores solids and liquids. This is a solid, so it's ignored. This is a solid, so it's ignored. That means that your equilibrium constant is just equal to O₂. Because there's a coefficient of 2 in front of that O₂ in the equation, that becomes the power. So it's O₂ squared. All we do now is, and we're going to say here, since we're looking for equilibrium pressure, this is actually K_p. So, K_p = 2.16 times 10 to the -2 equals the pressure O₂ squared. So, technically, because we're looking for the equilibrium pressure, we should have done this: We use those brackets to represent concentration if we're dealing with finding the equilibrium concentration of O₂. Here, all we do now is take the square root of this side and the square root of this side, and what's left will be 0.147. When we're dealing with K_p, the units are in atmospheres. This represents the equilibrium pressure of O₂ gas. If we were asked to find the equilibrium concentration, we would have used K_c and it would have looked like this. In that case, when we took the square root of both sides, we would have found our answer in molarity. But again, we're looking for equilibrium pressure here and not equilibrium concentration. So, realize these are the fundamental steps you need to take, whether you're looking for the Gibbs free energy, equilibrium concentration, or any given compound within your balanced equation. So keep these in mind as you approach questions like this later on. Now that you've seen this example, look to see if you can do example 2 that's given below. Remember, we have the equations that relate ΔG to different variables on the previous page. Utilize one of those equations to help answer example 2. Once you've done that, you can come back and see how I approach that same example 2 question.

Gibbs Free Energy Calculations 1

So here we're told that for the reaction of 2 moles of carbon graphite reacting with 1 mole of hydrogen gas produces 1 mole of ethylene gas. Here, we're told that Gibbs free energy under standard conditions is 209.2 kilojoules at 25 degrees Celsius. Now we're also told that if the partial pressure of hydrogen gas equals 100 atmospheres and the partial pressure of ethylene gas equals 0.10 atmospheres, calculate Gibbs free energy under non-standard conditions for the reaction. Here we're dealing with Gibbs free energy under standard conditions and non-standard conditions. The equation that connects them together is Gibbs free energy under non-standard conditions equals Gibbs free energy under standard conditions plus RTlnq. Remember, q is just your reaction quotient. It's equal to products over reactants just like your equilibrium constant.

Just like your equilibrium constant, we're going to ignore solids and liquids. Here this would be C₂H₂ divided by H₂. Here, we're going to plug in their partial pressures. Technically, again, we're dealing with pressure so technically, we should only reserve brackets when we're dealing with concentrations. Technically, it's p C₂H₂ and then p H₂ here on the bottom. So here, my q would be 0.10 divided by 100 for q. Now, here, R has joules in its units, so we should convert the 209.2 kilojoules into joules. Remember, 1 kilojoule is equal to 1000 joules, so that's 209,200 joules. Take that number, plug it in. Plus R which is 8.314 joules over moles times K. Temperature has to be in Kelvin. So to the 25 degrees Celsius, you add 273.15 And then ln of 0.10 And then ln of 0.10 divided by 100. So here, when we work this out, that's going to come out to be 192,077 joules as our units. But all the answers here are in kilojoules so we do one more conversion. Remember, 1 kilojoule is equal to 1000 joules. So that's 192.077 kilojoules, which rounds up to 192.1 kilojoules, making option A our correct choice. Just remember, in this question, we're dealing with Gibbs free energy both under standard conditions and non-standard conditions. So you just have to recall what equation connects those two variables together and then plug in desired units to find your answer at the end.

Gibbs Free Energy Calculations 2

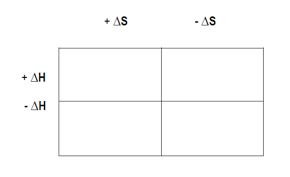
So here it states, sodium carbonate can be made by heating sodium bicarbonate. Here it says, given that the enthalpy under standard conditions equals 128.9 kilojoules per mole and the standard Gibbs free energy is 33.1 kilojoules per mole at 25 degrees Celsius. Above what minimum temperature will the reaction become spontaneous under standard state conditions? All right. We're given in this question delta H, delta G, and temperature. We know the equation that can link these variables together is ΔG = ΔH - TΔS. They're asking us what minimum temperature. Normally for a question like this where they're asking for the minimum temperature or asking for the temperature to make it spontaneous in general, we would set delta G to equal to 0. The issue with that is if I set delta G equal to 0, I'm still missing another variable. I'm missing delta S. What I need to do first is I need to determine what delta S will be within this question. So what we're going to do first is we're going to figure out what delta S is. We're going to input all the values we have for delta H, delta G, and temperature right now. Realize here we're going to plug in delta G, which is 33.1 kilojoules per mole. Delta H is 128.9 kilojoules per mole. Remember, temperature here needs to be in Kelvin, so we're going to add 273.15 to this. My temperature becomes 298.15 Kelvin. Then here, we don't know what delta S is. That's what we're solving for. First, we're going to subtract 128.9 kilojoules per mole. 128.9 kilojoules per mole. This cancels out here. We'll get here 95.8 kilojoules per mole equals negative 298.15 Kelvin times delta S. Divide out negative 298.15 Kelvin. This cancels out with this. At this point, we'll have a delta S equals. At this point, it equals 0.321315 kilojoules per moles times K. Now that we have that, we have to solve for the temperature needed for this question. So we write the equation again, ΔG = ΔH - TΔS. This time, to find the temperature, we're going to set delta G equal to 0 and we're going to input the values we have for delta H and our newly found delta S. So delta H is still 128.9 kilojoules per mole. We don't know what the new temperature is, that's what we're solving for. Delta S is 0.321315 kilojoules per moles times K like we found. Subtract 128.9 kilojoules per mole, from both sides. So negative 128.9 kilojoules per mole equals negative t times 0.321315 kilojoules per moles times K. So divide all this out. So cancels cancels. So right now, our temperature equals 401.164 Kelvin. Now let's talk about this answer. This question is saying, above what minimum temperature? Why exactly does it say above? Why doesn't it say below? Why doesn't it say at? Well, the first thing, we're looking for it to be spontaneous. Right? For it to be spontaneous, that means that delta G will have to be less than 0. At exactly 0, we are at equilibrium. At exactly this temperature, our reaction is not spontaneous. In fact, it is at equilibrium. Alright. We know why the answer is not exactly that number. But again, why is it above what minimum temperature? Why is it not below what minimum temperature? Well, if we think about the equation ΔG = ΔH - TΔS, we're going to come up with this chart here. Here, we're going to have delta H and delta H. Here, it's positive and here, it's negative. Delta S here is positive. Delta S here is negative. Using this Punnett square, we're going to say that if delta H is positive and delta S is positive, at higher temperatures, your reaction becomes spontaneous. It makes sense because if you think about it mathematically, this number here will be positive minus this temperature which is positive times delta S which is positive. Right? So think about it. You have these two numbers multiplying each other, so it's going to be positive. And the bigger this is and it subtracts from this positive number, the more negative delta G will be, so the more spontaneous they're going to be. You would want the temperature to be as high as possible to make this a larger value which subtracts from this positive here. If delta H is positive and delta S is negative, then we're non-spontaneous at all temperatures because again, this is positive. This here is positive but now this is negative. This is going to be a negative value and a negative minus a negative really means positive. You're really adding 2 positive values together, giving you a delta G that's positive. There'd be no situation in which delta G could be less than 0. Here, you'd always be spontaneous. And then here, you'd become more spontaneous the lower the temperature gets. Here, delta S, we found was a positive value and delta H from the beginning was a positive value, meaning you fall right here in terms of this Punnett square. The higher the temperature gets, the more spontaneous we get. That's why it says above what minimum temperature. That's why above this number, your reaction becomes more spontaneous. So d would be our answer. For it to be for the word below, we would have to fall here in the Punnett Square. That would mean that my Delta S would have to be negative, and my Delta H would have to be negative. In that case, we'd say the lower your temperature gets, so below what minimum temperature would you become spontaneous? Just remember, words are important here. It helps us to determine basically if a reaction will be spontaneous or not. Think about what conditions help to make delta G less than 0. The lower delta G becomes, the more spontaneous it becomes. And remember this Punnett square to help you understand how the signs of delta H and delta S have a direct relationship with determining the sign for delta G. Now that we've seen this one and seen this Punnett Square, look to see if you can solve this question given to us below. Recall all the things that we've said in terms of spontaneous reactions, the direction that's favored, and non-spontaneous reactions and what direction is favored. Once you do that, come back and take a look and see if your answer matches up with mine.

Here’s what students ask on this topic:

What is Gibbs free energy and why is it important in chemical reactions?

Gibbs free energy (ΔG) is a thermodynamic quantity that combines enthalpy (ΔH) and entropy (ΔS) to predict the spontaneity of a reaction. It is defined by the equation:

$\mathrm{\Delta G}=\mathrm{\Delta H}-T\mathrm{\Delta S}$

where T is the temperature in Kelvin. A negative ΔG indicates a spontaneous reaction, meaning it can occur without external energy. Conversely, a positive ΔG indicates a non-spontaneous reaction, requiring energy input. Understanding ΔG helps chemists predict reaction behavior, optimize conditions, and design processes efficiently.

How does Gibbs free energy relate to the equilibrium constant (K)?

Gibbs free energy (ΔG) is related to the equilibrium constant (K) through the equation:

$\mathrm{\Delta G}=-\mathrm{RT}lnK$

where R is the gas constant (8.314 J/mol·K) and T is the temperature in Kelvin. At equilibrium, ΔG is zero, and the equation simplifies to:

$\mathrm{\Delta G}=\mathrm{\Delta G}°+\mathrm{RT}lnQ$

where ΔG° is the standard Gibbs free energy change and Q is the reaction quotient. This relationship helps determine the direction and extent of a reaction under different conditions.

What is the significance of ΔG being less than, greater than, or equal to zero?

The sign of ΔG determines the spontaneity of a reaction:

• ΔG < 0: The reaction is spontaneous and can proceed without external energy. This is also known as an exergonic reaction.
• ΔG > 0: The reaction is non-spontaneous and requires energy input to proceed. This is known as an endergonic reaction.
• ΔG = 0: The reaction is at equilibrium, meaning the forward and reverse reactions occur at the same rate, and there is no net change in the concentrations of reactants and products.

Understanding these conditions helps predict and control chemical processes.

How do temperature and entropy affect Gibbs free energy?

Temperature (T) and entropy (ΔS) significantly influence Gibbs free energy (ΔG). The relationship is given by:

$\mathrm{\Delta G}=\mathrm{\Delta H}-T\mathrm{\Delta S}$

where ΔH is the enthalpy change. An increase in temperature can make a reaction more spontaneous if ΔS is positive, as the TΔS term becomes larger, reducing ΔG. Conversely, if ΔS is negative, increasing temperature can make the reaction less spontaneous. Thus, temperature and entropy are crucial in determining the spontaneity and feasibility of chemical reactions.

What are standard conditions in the context of Gibbs free energy?

Standard conditions for Gibbs free energy (ΔG°) refer to a set of specific conditions used to compare different reactions. These conditions are:

• Pressure: 1 atmosphere (atm)
• Concentration: 1 molar (M) for solutions
• Temperature: 25°C (298 K)

Under these conditions, the standard Gibbs free energy change (ΔG°) can be calculated and used to predict the spontaneity of reactions. It provides a reference point for comparing the thermodynamic properties of different reactions.