Chemical Thermodynamics: Gibbs Free Energy - Online Tutor, Practice Problems & Exam Prep

Gibbs free energy (ΔG) is crucial for understanding spontaneous reactions, which occur naturally without external energy. For spontaneity, ΔG must be less than 0, the entropy of the universe (ΔS) greater than 0, and the equilibrium constant (K) greater than 1. In contrast, non-spontaneous reactions have ΔG > 0, ΔS < 0, and K < 1. At equilibrium, ΔG = 0, ΔS = 0, and K = 1. The relationship between ΔG and enthalpy (ΔH) is expressed as ΔG = ΔH - TΔS, where T is temperature in Kelvin.

Gibbs Free Energy

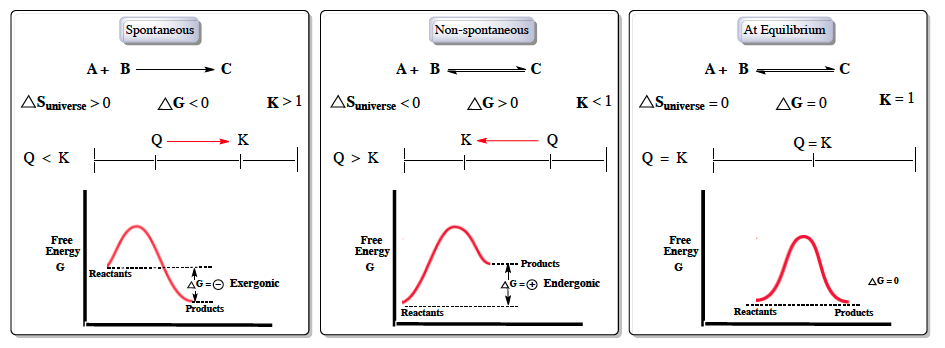
Hey everyone. So when we talk about Gibbs free energy, it's usually in reference to spontaneous reactions. Now, spontaneous reactions can occur without any outside energy being invested; they happen naturally. Non-spontaneous reactions, on the other hand, cannot occur naturally. Now, when we talk about spontaneous reactions, we're talking about spontaneous, non-spontaneous, and being at equilibrium.

And with these ideas, we have the variables of Gibbs free energy, entropy of our universe, which is tied to the second law of thermodynamics, and then our equilibrium constant K. So if we take a look at spontaneous reactions, here we have our reactants A and B producing our product C. For this to be a spontaneous result, we would say that the entropy of our universe would have to be greater than 0, following the second law of thermodynamics. Gibbs free energy Δg would have to be less than 0, and then our equilibrium constant K would have to be greater than 1. Typically, when we're talking about a spontaneous reaction that means that the reactants naturally flow in the forward direction to create products.

We can attach this idea to the whole thing of the reaction quotient q. To move in the forward direction, the reaction quotient q would have to be less than k. Remember that q means the shift to get to equilibrium, to get to k. Since q is less than k, q would move in the forward direction to get to k, which would mean that the reaction would also move in the forward direction. Now, if we're to talk about Gibbs free energy in terms of an energy diagram, realize that we have our reactants and then we're trying to get to our products.

For a reaction to be favorable, we'd want our products to be lower in overall energy. So here we'd see that our reactants are starting up at a higher energy level, and our products are ending at a lower level. Here to understand the value associated with this is just that Δg equals products minus reactants. So this is the equation we can link to each one of these energy diagrams. As a result of products being lower in energy, that means our Δg value overall is negative or what we call exergonic.

For a non-spontaneous reaction, it's basically the opposite process. So signs get flipped; here we'd say that our entropy of the universe would be less than 0, Gibbs free energy would be greater than 0, k would be less than 1. In a non-spontaneous reaction, that means that it's not favorable in the forward direction, but it would be favorable in the reverse direction. So here, to favor the reverse direction, that happens when q, our reaction quotient, is greater than k. So here since q is greater than k, q always shifts to wherever k is, so it's going to shift in the forward reverse direction to obtain equilibrium.

Here, when we're talking about the energy diagram again, this time, products will be higher in energy, which is not something we want. The whole purpose of most chemical reactions is to create a product that is lower in energy. Following products minus reactants, ΔG would be positive here, meaning that it is endergonic. And then finally, when we're neither spontaneous nor non-spontaneous, we are at equilibrium. So here ΔS of Universe would be equal to 0.

We'd say that Gibbs free energy is equal to 0. K is equal to 1. Since we're at equilibrium, our reaction quotient q is equal to our equilibrium constant k, so there's no shifting. And then looking at our energy diagrams, reactant products will end at the same energy level, so overall our Δg value will be equal to 0. Now, beside these conceptual ideas, we can also talk about the formulas associated with our Gibbs free energy.

Gibbs free energy can also be calculated through the use of various equations. So here we have Gibbs free energy under non-standard conditions equals the change in enthalpy minus T times the change in entropy. Here, realize that there's no circle here. That means that when there's no circle here, that's non-standard conditions. If I were to talk about standard conditions, I'd put a little circle there for each of them.

Standard conditions would mean that our pressure is 1 atmosphere. Standard conditions would also mean that our molarity is 1 molar. Standard conditions here could also mean that our temperature is 25 degrees Celsius. Now with our Gibbs free energy under non-standard conditions, we can have it equal to Gibbs free energy under standard conditions plus RT times lnq. R here represents our gas constant and since we're talking about energy it would be in the form of 8.314 joules over moles times k.

T here would be our temperature in Kelvin, and q again is our reaction quotient which is equal to products over reactants. And then finally, we can associate our Gibbs free energy under standard conditions with our equilibrium constant k by the use of this equation negative RT ln k. So, just remember that a spontaneous reaction can occur naturally without the use of outside energy. Associated with this idea of spontaneity, we have variables of Delta S of the universe, our equilibrium constant k, as well as, of course, our Gibbs free energy which is Delta g.

Gibbs Free Energy

Recall that a spontaneous reaction is one that happens naturally without the need of outside energy. Whereas a non-spontaneous reaction is one that doesn't normally occur unless some outside force is in play. If we take a look here, we have to figure out which of the following is an example of a non-spontaneous process. Alright. First, we have ice melting at room temperature.

Now we know that as long as the temperature is above 0 degrees Celsius, which room temperature is, ice will naturally melt. Because this happens in real world scenarios, it is a spontaneous process. Next, sodium metal reacting violently with water. This one, probably not as well known. Just remember here that when it comes to group 1a and 2a metals, because of the low number of valence electrons they have, they will violently react with water in order to lose an electron to become more like a noble gas.

Here, this is true. Because it happens naturally in the real world, it's a spontaneous process. Here, rusting of iron at room temperature. So old cars, when it rains, can rust. This is a natural process that happens every day.

And because of that, it's a spontaneous process. A ball rolling downhill. So a ball can naturally roll down a hill using its momentum as well as gravity to push itself down the hill. We can see that this happens, just by imagining it. You could take a ball and throw it down a hill.

You'll see it roll down naturally, so it's a spontaneous process. Now even if you weren't able to determine if the initial four were spontaneous or not, you should know that "e" is a non-spontaneous process. Here, it says water freezing at room temperature. Room temperature is much too warm for water to freeze. Water has to be at 0 degrees Celsius or lower in order to begin to freeze.

We should know that this makes no sense. It's not a natural occurrence. It could only happen if we invested some outside energy. For example, we have a refrigerator where we put an ice tray in. The refrigerator is in a room at room temperature but inside the refrigerator, it's much lower than that.

Therefore, water could freeze. The last option here, we're gonna say, does not naturally occur. Water will not freeze at room temperature under natural conditions. Therefore, it is the process that is non-spontaneous. Also remember, as we said above, spontaneous reactions move in the forward direction.

They never move in the reverse direction because if it's spontaneous, it's going to move forward. It is non-reversible or irreversible. Non-spontaneous processes are not spontaneous in the forward direction, so they tend to move in the reverse direction to become spontaneous. So non-spontaneous processes are reversible.

Gibbs Free Energy Calculations 1

Here it says, consider the decomposition of a metal oxide to its elements where m represents a generic metal. Here we have M₃O₄ solid decomposes to give us 3 moles M solid plus 2 moles of oxygen gas. We're also given the Gibbs free energies of formation, so ΔG_f here, of these 4 separate compounds. Now if we look at part 1, it says, what is the standard change in Gibbs energy for the reaction as written in the forward direction? So they're asking us to find ΔG of the reaction.

And standard here means that we have this little circle here. So remember, whether you're looking for ΔG of reaction, ΔS of reaction, or ΔH of reaction, all of them equal products minus reactants. So realize here that the Gibbs free energy of formation for M solid and oxygen gas are both 0. That's because when an element is in its natural standard state, its Gibbs free energy will be 0 just like its enthalpy would be 0.

Here, we're going to say we have 3 moles of M solid which really isn't going to change anything and the 3 comes from here times 0 plus 2 moles O₂. Each one is also 0. Minus, we have 1 mole of M₃O₄. Its Gibbs free energy of formation is this negative 9.50 kilojoules per mole.

So all of this here is 0, so we can ignore that. And we have a negative of a negative which comes out to a positive. So that's a positive 9.50 kilojoules because here, the moles cancel out. Now for part 2, it says, what is the equilibrium constant of this reaction as written in the forward direction at 298 Kelvin? From the previous page, we've seen that ΔG can be connected to our equilibrium constant k by this formula.

<math><mi>ΔG</mi> = <mo>−</mo><mi>R</mi><mi>T</mi><mi>ln</mi><mi>K</mi></math>. But here's the thing. We don't want to find ΔG. We already found that in part 1. Now in part 2, we're looking for what our equilibrium constant k is.

Finding k means we rearrange the equation now to become k = e, which is the inverse of the natural log, to the negative ΔG₀ divided by R T. This is the equation we're going to use now. It's e to the negative. R here is in joules, so we're going to have to convert the ΔG we found earlier also into joules. So remember, 1 kilojoule is equal to 1,000 joules.

So that's 9,500 joules. So 9,500 joules per mole here divided by 8.314 joules over moles times k, which is the value of our R constant. And the temperature here is 298 Kelvin. Here, moles will cancel out, joules will cancel out and kelvins will cancel out. Meaning that our equilibrium constant has no units.

This becomes e to the negative 3.8344. When you plug that into your calculator, that's going to give you 2.16 times 10 to the negative 2 for my value, for my equilibrium constant k. So now that we found that, we finally figure out the last portion, part 3. What is the equilibrium pressure of O₂ over M solid at 298 Kelvin? Alright.

So now we're being asked to figure out the equilibrium pressure of this gas. Now here because they're using the term 'equilibrium pressure' or 'equilibrium concentration,' that means we're going to have to use the equilibrium constant we just found. So k = products over reactants. Remember, your equilibrium constant ignores solids and liquids. This is a solid, so it's ignored.

This is a solid, so it's ignored. That means that your equilibrium constant is just equal to O₂. Because there's a coefficient of 2 in front of that O₂ in the equation, that becomes the power. So it's O₂². All we do now and we're going to say here, since we're looking for equilibrium pressure, this is actually K_p.

Now we're going to say here K_p is the k that we found here. That's 2.16 times 10 to the negative 2 equals the pressure of O₂ squared. Since we're looking for the equilibrium pressure, we should have done this. We use those brackets to represent concentration if we're dealing with finding the equilibrium concentration of O₂.

Now, all we do is take the square root of this side and the square root of this side, and what's left will be 0.147 atmospheres. This represents the equilibrium pressure of O₂ gas. If we were asked to find the equilibrium concentration, we would have used K_c and it would have looked like this. In that case, when we took the square root of both sides, we'd have found our answer in molarity.

But again, we're looking for equilibrium pressure here and not equilibrium concentration. So realize these are the fundamental steps you need to take whether you're looking for the Gibbs free energy of a reaction, your equilibrium concentration of any given compound within your balanced equation. So keep these in mind as you approach questions like this later on. Now that you've seen this example, look to see if you can do example 2 that's given below. Remember, we have the equations that relate ΔG to different variables on the previous page.

Utilize one of those equations to help answer example 2. Once you've done that, you can come back and see how I approach that same example 2 question.

Gibbs Free Energy Calculations 1

So here we're told for the reaction of 2 moles of carbon graphite reacting with 1 mole of hydrogen gas produces 1 mole of ethylene gas. Here we're told that Gibbs free energy under standard conditions is 209.2 kilojoules at 25 degrees Celsius. Now we're also told that if the partial pressure of hydrogen gas equals 100 atmospheres and the partial pressure of ethylene gas equals 0.10 atmospheres, calculate Gibbs free energy under non-standard conditions for the reaction. Here we're dealing with Gibbs free energy under standard conditions and non-standard conditions. The equation that connects them together is Gibbs free energy under non-standard conditions equals Gibbs free energy under standard conditions plus RTlnq.

Remember, q is just your reaction quotient. It's equal to products over reactants just like your equilibrium constant. Just like your equilibrium constant, we're going to ignore solids and liquids. Here this would be pC2H2pH2 divided by H2. Here, we're going to plug in their partial pressures.

Technically, we're dealing with pressure, so it's pC2H4 and then pH2 here on the bottom. Here, my q would be 0.10 divided by 100 for q. Now, R has joules in its units, so we should convert the 209.2 kilojoules into joules. Remember, 1 kilojoule is equal to 1000 joules, so that's 209,200 joules.

Take that number, plug it in, plus R which is 8.314 joules over moles times K. Temperature has to be in Kelvin. So to the 25 degrees Celsius, you add 273.15, which comes out to 298.15 Kelvin. And then ln of 0.10 divided by 100. Here, when we work this out, that's going to come out to be 192077 joules as our units.

But all the answers here are in kilojoules, so we do one more conversion. Remember, 1 kilojoule is equal to 1000 joules. That's 192.077 kilojoules which rounds up to 192.1 kilojoules, making option A our correct choice. So just remember, in this question, we're dealing with Gibbs free energy both under standard conditions and non-standard conditions. So you just have to recall what equation connects those two variables together and then plug in the desired units to find your answer at the end.

Gibbs Free Energy Calculations 2

So here it states, sodium carbonate can be made by heating sodium bicarbonate. It says, given that the enthalpy under standard conditions equals 128.9 kilojoules per mole and the standard Gibbs free energy is 33.1 kilojoules per mole at 25 degrees Celsius. Above what minimum temperature will the reaction become spontaneous under standard state conditions? All right. We're given in this question delta H, delta G and temperature.

We know the equation that can link these variables together is ΔG=ΔH-tΔs. They're asking us what minimum temperature. Normally for a question like this where they're asking for the minimum temperature, or asking for the temperature to make it spontaneous in general, we would set delta G to equal to 0. The issue with that is, if I set delta G equal to 0, I'm still missing another variable. I'm missing delta S.

What I need to do first is determine what delta S will be within this question. We're going to input all the values we have for delta H, delta G, and temperature right now. Realize here we're going to plug in delta G, which is 33.1 kilojoules per mole. Delta H is 128.9 kilojoules per mole.

Remember, the temperature here needs to be in Kelvin, so we're going to add 273.15 to this. So my temperature becomes 298.15 Kelvin. Then here, we don't know what delta S is. That's what we're solving for. First, we're going to subtract 128.9 kilojoules per mole.

-95.8kJ/mol=-298.15K×Δs. Divide out -298.15 K. At this point, Δs=0.321315kJ/mol×K. Now that we have that, we have to solve for the temperature needed for this question. We write the equation again, ΔG=ΔH-tΔs. This time, to find the temperature, we're going to set delta G equal to 0 and input the values we have for delta H and our newly found delta S.

Delta H is still 128.9 kilojoules per mole. We don't know what the new temperature is, that's what we're solving for. Delta S as found is 0.321315 kilojoules/mole×K. Subtract 128.9 kilojoules per mole, from both sides. So -128.9kJ/mol=-t×0.321315kJ/mol×K.

So divide all this out. So right now our temperature equals 401.164 K. Now let's talk about this answer. This question is asking above what minimum temperature.

Why exactly does it say above? Why doesn't it say below? Why doesn't it say at? Well, first, we're looking for it to be spontaneous. Right? For us to be spontaneous, that means that delta G will have to be less than 0. At exactly 0, we are at equilibrium. At exactly this temperature, our reaction is not spontaneous. In fact, it is at equilibrium. Alright.

We know why the answer is not exactly that number. But again, why is it above what minimum temperature? Why is it not below what minimum temperature? If we think about the equation ΔG=ΔH-tΔs, and using the analysis of signs for delta H and delta S, we conclude that if both delta H and delta S are positive, then increasing the temperature will increase the spontaneity of the reaction. That's why it says above what minimum temperature. This number is the minimum temperature where the reaction becomes spontaneous. So d would be our answer.

For it to use the word below, we would have to fall here in the Punnett Square. That would mean that my delta S would have to be negative, and my delta H would have to be negative. In that case, we'd say the lower your temperature gets, so below what minimum temperature would you become spontaneous. Just remember, words are important here. It helps us to determine basically if a reaction will be spontaneous or not. Think about what conditions help to make delta G less than 0. The lower delta g becomes, the more spontaneous it becomes. And remember this Punnett Square to help you understand how the signs of delta h and delta s have a direct relationship with determining the sign for delta g.

Here’s what students ask on this topic:

What is Gibbs free energy and why is it important in chemical reactions?

Gibbs free energy (ΔG) is a thermodynamic quantity that combines enthalpy (ΔH) and entropy (ΔS) to predict the spontaneity of a reaction. It is defined by the equation:

$\mathrm{\Delta G}=\mathrm{\Delta H}-T\mathrm{\Delta S}$

where T is the temperature in Kelvin. A negative ΔG indicates a spontaneous reaction, meaning it can occur without external energy. Conversely, a positive ΔG indicates a non-spontaneous reaction, requiring energy input. Understanding ΔG helps chemists predict reaction behavior, optimize conditions, and design processes efficiently.

How does Gibbs free energy relate to the equilibrium constant (K)?

Gibbs free energy (ΔG) is related to the equilibrium constant (K) through the equation:

$\mathrm{\Delta G}=-\mathrm{RT}lnK$

where R is the gas constant (8.314 J/mol·K) and T is the temperature in Kelvin. At equilibrium, ΔG is zero, and the equation simplifies to:

$\mathrm{\Delta G}=\mathrm{\Delta G}°+\mathrm{RT}lnQ$

where ΔG° is the standard Gibbs free energy change and Q is the reaction quotient. This relationship helps determine the direction and extent of a reaction under different conditions.

What is the significance of ΔG being less than, greater than, or equal to zero?

The sign of ΔG determines the spontaneity of a reaction:

• ΔG < 0: The reaction is spontaneous and can proceed without external energy. This is also known as an exergonic reaction.
• ΔG > 0: The reaction is non-spontaneous and requires energy input to proceed. This is known as an endergonic reaction.
• ΔG = 0: The reaction is at equilibrium, meaning the forward and reverse reactions occur at the same rate, and there is no net change in the concentrations of reactants and products.

Understanding these conditions helps predict and control chemical processes.

How do temperature and entropy affect Gibbs free energy?

Temperature (T) and entropy (ΔS) significantly influence Gibbs free energy (ΔG). The relationship is given by:

$\mathrm{\Delta G}=\mathrm{\Delta H}-T\mathrm{\Delta S}$

where ΔH is the enthalpy change. An increase in temperature can make a reaction more spontaneous if ΔS is positive, as the TΔS term becomes larger, reducing ΔG. Conversely, if ΔS is negative, increasing temperature can make the reaction less spontaneous. Thus, temperature and entropy are crucial in determining the spontaneity and feasibility of chemical reactions.

What are standard conditions in the context of Gibbs free energy?

Standard conditions for Gibbs free energy (ΔG°) refer to a set of specific conditions used to compare different reactions. These conditions are:

• Pressure: 1 atmosphere (atm)
• Concentration: 1 molar (M) for solutions
• Temperature: 25°C (298 K)

Under these conditions, the standard Gibbs free energy change (ΔG°) can be calculated and used to predict the spontaneity of reactions. It provides a reference point for comparing the thermodynamic properties of different reactions.