Gravimetric Analysis - Online Tutor, Practice Problems & Exam Prep

Electrogravimetric Analysis Example 1

In gravimetric analysis, the mass of a product within a chemical reaction can be used to determine the amount of the original analyte. Now if we take a look here at this example, it states a 25 milliliter solution containing bromide ion was treated with excess lead(II) sulfate to precipitate 0.7550 grams of lead(II) bromide. What was the molarity of the bromide ion in the unknown? Alright. So we're being asked to determine the molarity of bromide ion. The molarity of bromide ion would equal the moles of bromide ion divided by liters of bromide ion solution. Now from the question given, we can see that we know the milliliters of bromide ion solution. It's 25 ml. So what I'm going to do here first is I'm going to convert 25 ml into liters. Remember, 1 milli is 10e-3l liters. So that comes out to be 0.025 liters. So we've determined the bottom portion of this molarity. Now we need to determine the moles of bromide ion. Now we know the amount of product obtained in the chemical reaction, so we can rely on stoichiometry and use those grams of lead(II) bromide to help us determine the moles of bromide ion. So we have 0.7550 grams of lead(II) bromide. It's composed of 1 lead and 2 bromines within the compound. When you look up their atomic masses from the periodic table and add them together, you'll get approximately 367.008 grams of lead(II) bromide, and that's for every 1 mole. Grams of lead(II) bromide cancel out. Now we just have to look at our balanced chemical equation and we see that for every 2 moles of bromide ion, I have 1 mole of lead(II) bromide. So 2 moles to 1 mole. So for every 1 mole of lead(II) bromide, I have 2 moles of bromide ion. When we work that out, we'll get 4.114×10-3moles of bromide ion. Take those moles and plug them into our molarity equation. That's negative 3. So when we work when we plug that in, we get 0.1646 molar bromide ion. Now realize here that our number at the end has 4 significant figures because 25.00 has 4 sig figs and 0.7550 grams also has 4 significant figures. Because of that, our answer will have 4 significant figures. Now that we've seen this example, move on to the next question as we delve deeper into gravimetric analysis.

Electrogravimetric Analysis Practice

So here in this practice question, it states, the iron in a 1.1530 gram sample of iron ore is precipitated as iron 3 oxide connected to an unknown amount of water molecules by the addition of ammonia. The residue is ignited at high temperatures to give 0.6310 grams of pure iron 3 oxide. Calculate the weight percent of iron in the ore. Alright. So they want us to determine the mass percent or weight percent of iron within our sample of iron ore. We're going to say here that the weight percent or mass percent of iron equals the grams of iron divided by the mass of the ore, so grams of ore. Now we already know how much of our iron ore we have. We're told that in the very first sentence. So we're going to plug that in. So that's 1.1530 grams of ore. What we need to do next though is we need to determine the amount of our iron analyte, and the way we're able to determine that is by using the mass of the product that we obtained from the chemical reaction. Alright. So we're going to take these 0.6310 grams of iron 3 oxide, we're going to change it into moles. So we're going to say 1 mole of iron 3 oxide, oxide. How many grams are involved? Well, it's composed of 2 irons and 3 oxygens, so the combined molar mass of it would come out to 159.687 grams. Here, the grams of iron 3 oxide cancel out and we're left with moles of iron 3 oxide. Now we need to make the jump from moles of iron 3 oxide to just moles of iron. Now if we look at our balanced equation, we can see that it's a 1 to 1 relationship. We can assume that, actually, a 1 to 2, sorry, 1 to 2 relationship between iron 3 oxide and iron 3 ion, and we can say that that iron 3 originates from the pure non charged iron form. So we can make that jump. So we're going to say here that for every 1 mole of iron 3 oxide, we have 2 moles of iron 3 ion, and by extension, 2 moles of iron. So these moles cancel out, and then we're gonna say here that we need grams of iron. So we're going to say here for every 1 mole of iron, its atomic mass according to the periodic table is 55.845 grams. Moles of iron cancel out, and at the end, we'll have 0.44134 grams of iron. Take those grams of iron and plug them in, multiply this by a 100 to get our percent, and when we do that we get approximately 38.3 percent as the weight percent of our iron within this ore sample. So, again, with the information from the amount of product obtained, we're able to gain information on the amount of our original analyte and by extension, in this case, the weight percent of that analyte.

So now that we've seen these two problems, let's move on to our next question dealing with gravimetric analysis.

Electrogravimetric Analysis Calculations Example 1

So here in this example, it states that the reaction between Piperazine and acetic acid creates an adduct product known as Piperazine diacetate. Here we have Piperazine, 1 mole of Piperazine, reacting with 2 moles of acetic acid to produce 1 mole of this larger product. Now, it says a 7.50 gram sample of impure pyrosine contained 83.01% Piperazine. How many grams of Piperazine diacetate would be formed in the process? They are asking us to determine the amount of our product, the grams of it, and they're giving us the grams of the impure Piperazine and its mass percent. With this information, we should be able to determine the mass of the product. Up to this point, we've been given the mass of our product and been able to use that to find the analyte; we're just trying to figure out how much product we have. You can approach this in the same way as any dimensional analysis question coupled with stoichiometry.

We have 7.50 grams of our impure Piperazine, which represents the grams of our solution. Since the Piperazine was pure, that would be 7.50 grams of it. Given the mass percent of Piperazine, for every 83.01 grams of Piperazine, there are 100 grams of our solution. By setting it up this way, we can see that the grams of solution will cancel out. Now that we have our grams of Piperazine, we can use stoichiometry to go from grams of Piperazine, to moles of Piperazine, to moles of our product. Piperazine itself means that each corner represents a carbon. Carbon itself must make 4 bonds, and those carbons to make 4 bonds have hydrogens that are not visible.

The formula here has 4 carbons, 10 hydrogens, and 2 nitrogens, giving us the molar mass of Piperazine. molarmass= 86.136g/mol . This corresponds to 1 mole. With grams of Piperazine now determined, we see from the balanced equation above that there's a 1:1 relationship, so for every 1 mole of Piperazine we have 1 mole of Piperazine diacetate. Moles cancel out, and we now have moles of our product. We just need to convert those moles into grams. The combined mass of the structure comes out to 206.236g/mol . Moles cancel out, leaving us with grams of Piperazine diacetate.

After working that out, we get 14.9 grams. Here, my answer has 3 significant figures because 7.50 has 3 significant figures and the percentage here has 4 significant figures. We always go with the least number of significant figures, so 14.9 will be our best answer. This is an example of how gravimetric analysis can be used; usually, we are given the mass of our product and with that, we determine the amount of our original analyte. In this case, we are given information on the analyte and use that to figure out the amount of product. Now that we've gone through this question, let's move on to the next.

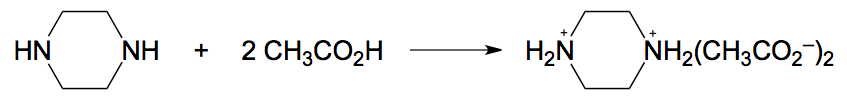
Electrogravimetric Analysis Calculations Example 2

So, in example 2, it says the amount of iron within an ore sample was determined by an oxidation reduction titration using potassium permanganate or KMnO₄ as the titrant. We are told that a 0.5600 gram sample of the ore was placed into acid and the newly freed iron ion was then reduced to iron ion. The titration of the solution required 39.82 milliliters of 0.0315 Molar Potassium Permanganate to reach the endpoint. Determine the mass percent of iron oxide in the sample. Alright, so in this question, we are dealing with volumetric determination. We are trying to figure out the content of iron within this sample by using the known molarity or known concentration of a standard of potassium permanganate.

Now, we want to find the mass percent of iron oxide in the sample. So, that would be mass percent of iron oxide equals the grams of iron oxide divided by grams of sample times 100. We're already given one of these right off the bat. We're told that our sample weighs 0.5600 grams. So that's going to go here on the bottom. What we have to do now is we have to use stoichiometry to isolate the grams of iron oxide. So we're going to do that by using the only other piece of information given to us that we have 39.82 ml of 0.0315 Molar potassium permanganate. Remember, if we can change these ml's into liters and multiply it by the molarity, we'll get the moles of potassium permanganate.

At this point, we should realize that we have a balanced equation before us, but it doesn't have exactly potassium permanganate within it. What it has closest to that is just the permanganate ion. So, we're going to convert our moles of potassium permanganate into moles of just permanganate, and we're going to say here, according to the relationship, for every one mole of my entire compound of potassium permanganate, there's exactly one mole of just permanganate.

Now that we have to find the grams of iron³ oxide, Iron³ oxide is also not in this balanced equation, but iron³ oxide has in it the iron³ ion, which is part of this equation. So we're going to continue by saying that according to my balanced equation, for every one mole of this, there are 5 moles of this. So, we have that now, and because of that, we can establish a relationship with iron³ oxide. We're going to say for every one mole of the entire compound of iron oxide, there are 2 moles of iron³ oxide. And then finally, we need grams. So for every 1 mole of iron³ oxide, how many grams of iron³ oxide do we have? So for that, we have to calculate the weight. So if we look at our periodic table, we have 2 irons and 3 oxygens in the compound. Iron weighs 55.845 grams, and oxygen weighs 15.99994 grams. So that gives us 111.69, 47.9982, which together is 159.688 grams. We take that and plug it in.

So we've just isolated our grams of iron³ oxide. Remember, analytical chemistry is the chemistry of precision, so we cannot round until the very end. So we're going to write all these numbers down. So there goes the grams of iron³ oxide. So take that and plug it into my mass percent formula. So that's going to give me a percentage of 89.42%, and we could just do 89.4, which has 3 significant figures, like this number here has 3 significant figures.

Just remember, the wording of a lot of these questions can be complicated at times, but what we've always done is we've written down first what they're asking me to find, then from that write down all the given information and try to decipher what parts can cancel out to get my desired units at the end. Take this to heart as we approach further questions dealing with titrations and eventually back titrations. Utilize the techniques we've learned to get the answers to those questions as well.